NOAA Manual NOS NGS 5 - State Plane Coordinate System of 1983

NOAA Manual NOS

NGS

5

State Plane Coordinate

System of 1983

James

E.

Stem

Rockville,

MD

January 1989

Reprinted with minor corrections

March 1990

U.S.

DEPARTMENT

OF

COMMERCE

National Oceanic and Atmospheric Administration

National Ocean Service

Charting

and

Geodetic Services

NOAA

Manual

NOS NGS 5

State Plane Coordinate

System of 1983

James

E.

Stem

National Geodetic Survey

Rockville,

MD

January 1989

Reprinted with minor corrections

March 1990

Reprinted February

1991

Reprinted

July

1992

~eprinted

January

1993

Reprinted

August

1993

Reprinted

April

1994

Re~rinted

January

1995

Re?rinted

September

1995

U.S. DEPARTMENT OF COMMERCE

C. William Verity, Secretary

National Oceanic and Atmospheric Administration

William

E.

Evans, Under Secretary

National Ocean Service

Thomas

J.

Maginnis,

Assistant

Administrator

Charting

and

Geodetic Services

R.

Adm. Wesley

V.

Hull

For

sale

by the National Geodetic Information Center, NOAA, Rockville,

MD

20852

PREFACE

This

manual

explains

how

to

perform

computations

on

the

State

Plane

Coordinate

System

of

1983 (SPCS

83).

It

supplements

Coast

and

Geodetic

Survey

Special

Publication

No.

235,

"The

State

coordinate

systems,"

and

replaces

Coast

and

Geodetic

Survey

Publication

62-4,

"State

plane

coordinates

by

automatic

data

processing.''

These

two

widely

distributed

publications

provided

the

surveying

and mapping

profession

with

information

on

deriving

1927

State

plane

coordinates

from

geodetic

coordinates

based

on

the

North

American Datum

of

1927

(NAD

27)

plus

information

for

traverse

and

other

computations

with

these

coordinates.

This

manual

serves

the

similar

purpose

for

users

of

SPCS

83

derived

from

the

North

American Datum

of

1983

(NAD

83).

Emphasis

is

placed

on

computations

that

have

changed

as

a

result

of

SPCS

83.

This

publication

is

neither

a

textbook

on

the

theory,

development,

or

applications

of

general

map

projections

nor

a manual

on

the

use

of

coordinates

in

survey

computations.

Instead

it

provides

the

practitioner

with

the

necessary

information

to

work

with

three

conformal

map

projections:

the

Lambert

conformal

conic,

the

transverse

Mercator,

and

the

oblique

Mercator.

Derivatives

of

these

three

map

projections

produce

the

system

which

the

National

Geodetic

Survey

(NGS)

has

named

the

State

Plane

Coordinate

System (SPCS).

Referred

to

NAD

83

or

NAD

27,

this

system

of

plane

coordinates

is

identified

as

SPCS

83

or

SPCS

27,

respectively.

The

equations

in

chapter

3,

Conversion

Methodology,

form a

significant

portion

of

the

manual.

Chapter

3

is

required

reading

for

programmers

writing

software,

but

practitioners

with

software

available

may

skip

this

chapter.

Although

a

modification

of

terminology

and

notation

was

suggested

by some

reviewers,

consistency

with

NGS

software

was deemed more

important.

Hence,

chapter

3

documents

the

SPCS

83

software

available

from

the

National

Geodetic

Survey.

iii

ACKNOWLEDGMENT

The

mathematics

given

in

this

manual

were

compiled

or

developed

by T.

Vincenty

prior

to

his

retirement

from

the

National

Geodetic

Survey

(NGS).

His

consultation

was

invaluable

to

the

author.

Principal

reviewer

was

Joseph

F.

Dracup,

NGS,

retired.

His

many

excellent

suggestions

were

incorporated

into

the

manual.

Once

again,

Joe

gave

generously

of

his

time

to

assist

in

the

education

of

the

surveying

profession.

The

author

appreciates

the

review

and

contributions

made by

Earl

F.

Burkholder,

Oregon

Institute

of

Technology.

Earl

spent

a summer

at

NGS

researching

the

subject

of

map

projections

and

maintains

a

continuing

interest

in

the

subject.

In

addition,

the

manual was

reviewed

by

Charles

A.

Whitten,

B.

K.

Meade,

and

Charles

N.

Claire,

all

retired

employees

of

the

former

Coast

and

Geodetic

Survey

(now NGS). The

author

was

very

fortunate

to

have

such

experts

donate

their

services.

Finally,

the

author

appreciates

the

helpful

guidance

of

John

G.

Gergen

and

Edward

J.

McKay,

present

NGS

employees.

iv

CONTENTS

Preface

........•..••...•.•...••........•..••.....•..........................

iii

Acknowledgment..............................................................

iv

1.

1

1.

2

1.

3

1.

4

1.

5

1 • 6

1.

7

2.

1

2.2

2.3

2.4

2.5

2.6

2.7

3.

3. 1

3.

11

3.

1 2

3.

1 3

3.

1 4

3.15

3.2

3.

21

3.22

3.23

3.24

3.25

3.26

3.3

3.

31

3.32

3.33

3.34

3.35

3.36

3.4

3.

41

3.42

Introduction

..••...•••.•••....•••..........................•...•••..•

Requirement

for

SPCS

83

..............................••...•.•.......•.

SPCS

27

background

...•...........................••.....•............•

SPCS

83

design

............................•.....••...•.•....•....•..••

SPCS

83

local

selection

•.•••....•••.................•.........••..•...

SPCS

83

State

legislation

..............•...•••....•...••••..••...•.•..

SPCS

83

unit

of

length

•....•.......•..........••...•.•....••...•...•..

The new

GRS

80

ellipsoid

..••..........................................

Map

projections

...•••..••.•...•.•...............•••.•.••••..•••..•...•

Fundamentals

••..•....•••...................................••...•...••

SPCS

83

grid

.......•.....••....•••...•..............•.•.••..•..•.••..•

Conformal

it

y

••.....................•........................•...••....

Convergence

angle

...••.•...••........................•..•••..••..••••.

Grid

azimuth

"t"

and

projected

geodetic

azimuth

"T''···················

Grid

scale

factor

at

a

point

•••..•••................•.•..••..•••.••••.

Universal

Transverse

Mercator

projection

.•..•••....•....••..•....••...

Conversion

methodology

.•......•.•...•.•..•••....•••....•..............

Lambert

conformal

conic

mapping

equations

(note

alternative

method

given

in

sec.

3.

4)

•..•••••.•••••...............•...••...••...

No

tat

ion

and

definitions

•.•.••••....•••..••••••.•.....................

Computation

of

zone

constants

...••••.••••••.••••...•.........•....••..

Direct

conversion

computation

..•••....••.........................•....

Inverse

conversion

computation

......•.••.•.•.••••..•.••.•.•..••....••.

Arc-to-chord

correction

(t-T)

..•••....•••..•.•....................•...

Transverse

Mercator

mapping

equations

..•.•••.......•..................

Notation

and

definition

.........•.....••...•.•••..••.....•....••..••..

Constants

for

meridional

distance

•..••...•••.•..••••.••...............

Direct

conversion

computation

•••.•••...••....•..............•..•......

Inverse

conversion

computation

•.•..•.........•...•••...••...••••......

Arc-to-chord

correction

(t-T)

••.••••.................••...•••....•...•

Grid

scale

factor

of

a

line

.•.•..•......•..•.•••.••••........•.•.....•

Oblique

Mercator

mapping

equations

...•••..••..........................

Notation

and

definition

•....•...•••...•••.........•...............•...

Computation

of

GRS

80

ellipsoid

constants

•...•........................

Computation

of

zone

constants

••...•.............••.•••...••••....•...•

Direct

conversion

computation

..••••....••.....••..•.............•....•

Inverse

conversion

computation

..••.••••...••••...................•....

Arc-to-chord

correction

(t-T)

and

grid

scale

factor

of

a

line

••••.•••..........•••••..•••••.•.•••..............•.......

Polynomial

coefficients

for

the

Lambert

projection

.•....•....•.•......

Direct

conversion

computation

.•.••••..................•..•.••..•..••.•

Inverse

conversion

computation

•••......•••.•.......•...•....•.........

v

2

4

5

8

11

12

14

1 4

16

17

18

21

24

26

27

28

29

32

33

35

37

38

39

40

41

42

44

45

4.

Line

conversion

methods

required

to

place

a

survey

on

SPCS

83.........

46

4.1

Reduction

of

observed

distances

to

the

ellipsoid

......................

46

4.2

Grid

scale

factor

k

12

of

a

line......................................

49

4.3

Arc-to-chord

correction

(t-T).........................................

51

4.4

Traverse

example

.........••...........................................

53

Bibliography................................................................

62

Appendix

A.

Defining

constants

for

the

State

Plane

Coordinate

System

of

1983......................................................

•.

63

Appendix

B.

Model

act

for

State

Plane

Coordinate

Systems

....•..•....•......

73

Appendix

C.

Constants

for

the

Lambert

projection

by

the

polynomial

coefficient

method.............................................

76

FIGURES

1

.4

State

Plane

Coordinate

System

of

1983

zones...........................

6

2.1a

The

three

basic

projection

surfaces

•••.•••............................

15

2.1b

Surfaces

used

in

State

Plane

Coordinate

Systems

..••...•...••..••......

16

2.5

Azimuths

•............••.•••...•.•...••...•...•.•......................

19

2.6

Scale

factor..........................................................

20

2.7

Universal

Transverse

Mercator

zones

........•....••.•••..••..••.•.••...

22

3.

4 The

Lambert

grid......................................................

43

4.1a

Geoid-ellipsoid-surface

relationships

...•.••.........•.•.•........••..

46

4.1b

Reduction

to

the

ellipsoid

•.•..••.....•.........•.....................

47

4.1c

Reduction

to

the

ellipsoid

(shown

with

negative

geoid

height)

........•

48

4.2

Geodetic

vs.

grid

distances...........................................

49

4.3

Projected

geodetic

vs.

grid

angles

•..•••••..•••..••.••••..••..•...•.•.

53

4.

4a

Sample

traverse.......................................................

54

4.4b

Fixed

station

control

information

..•...•••...••..••.•..••...•.•...•...

56

4.4c

(t-T)

correction

......................................................

58

4.

4d

Azimuth

adjustment....................................................

59

4.4e

Traverse

computation

by

latitudes

and

departures

•..••.................

60

4.4f

Adjusted

traverse

data

................................................

60

vi

TABLES

1.5

Status

of

SPCS

27

and

SPCS

83

legislation............................

9

3.

0 Summary

of

conversion

methods........................................

24

3.1

True

values

of

(t-T)

and

computational

errors

in

their

determination......................................................

32

3.22

Intermediate

constants

for

the

transverse

Mercator.

projections.......

34

4.3a

Approximate

size

of

(t-T)

in

seconds

of

arc

for

Lambert

or

transverse

Mercator

projection..........................

51

4.3b

Sign

of

(t-T)

correction.............................................

52

vii

THE

STATE

PLANE

COORDINATE

SYSTEM

OF

1983

James

E.

Stem

National

Geodetic

Survey

Charting

and

Geodetic

Services

National

Ocean

Service,

NOAA

Rockville,

MD

20852

ABSTRACT.

This

manual

provides

information

and

equations

necessary

to

perform

survey

computations

on

the

State

Plane

Coordinate

System

of

1983

(SPCS

83),

a

map

projection

system

based

on

the

North

American Datum

of

1983

(NAD

83).

Given

the

geodetic

coordinates

on

NAD

83

(latitude

and

longitude),

the

manual

provides

the

necessary

equations

to

compute

State

plane

coordinates

(northing,

easting)

using

the

"forward"

mapping

equation

(cp,

>.

..

N,

E).

"Inverse"

mapping

equations

are

given

to

compute

the

geodetic

position

of

a

point

defined

by

State

plane

coordinates

(N,E .. cp,>.).

The

manual

addresses

corrections

to

angles,

azimuths,

and

distances

that

are

required

to

relate

these

geodetic

quantities

between

the

ellipsoid

and

the

grid.

The

following

map

projections

are

defined

within

SPCS

83:

Lambert

conformal

conic,

transverse

Mercator,

and

oblique

Mercator.

A

section

on

t~a

Universal

Transverse

Mercator

(UTM)

projection

is

included.

UTM

is

a

derivative

of

the

general

transverse

Mercator

projection

as

well

as

another

projection,

in

addition

to

SPCS

83, on which

NAD

83

is

published

by

NGS.

1.

INTRODUCTION

1.1

Requirement

for

SPCS

83

The

necessity

for

SPCS

83

arose

from

the

establishment

of

NAD

83.

When

NAD

27

was

readjusted

and

redefined

by

the

National

Geodetic

Survey,

a

project

which

began

in

1975 and

finished

in

1986,

SPCS

27

became

obsolete.

NAD

83

produced

new

geodetic

coordinates

for

all

horizontal

control

points

in

the

National

Geodetic

Reference

System (NGRS).

The

project

was

undertaken

because

NAD

27

values

could

no

longer

provide

the

quality

of

horizontal

control

required

by

surveyors

and

engineers

without

regional

recomputations

(least

squares

adjustments)

to

repair

the

existing

network.

NAD

83

supplied

the

following

improvements:

o

One

hundred

and

fifty

years

of

geodetic

observations

(approximately

1.8

million)

were

adjusted

simultaneously,

eliminating

error

propagation

which

occurs

when

projects

must be

mathematically

assembled

on a

"piecemeal"

basis.

o The

precise

transcontinental

traverse,

satellite

triangulation,

Doppler

positions,

baselines

established

by

electronic

distance

measurements

(EDM),

and

baselines

established

by

very

long

baseline

interferometry

(VLBI),

improved

the

internal

consistency

of

the

network.

o A

new

figure

of

the

Earth,

the

Geodetic

Reference

System

of

1980

(GRS

80),

which

approximates

the

Earth's

true

size

and

shape,

supplied

a

better

fit

than

the

Clarke

1866

spheroid,

the

reference

surface

used

with

NAD

27.

o The

origin

of

the

datum was moved from

station

MEADES

RANCH

in

Kansas

to

the

Earth's

center

of

mass,

for

compatibility

with

satellite

systems.

Not

only

will

the

published

geodetic

position

of

each

control

point

change,

but

the

State

plane

coordinates

will

change

for

the

following

reasons:

o The

plane

coordinates

are

mathematically

derived

(using

•mapping

equations•)

from

geodetic

coordinates.

o The

new

figure

of

the

Earth,

the

GRS

80

ellipsoid,

has

different

values

for

the

semimajor

axis

•a•

and

flattening

"f"

(and

eccentricity

•e•

and

semiminor

axis

"b"

) •

These

ellipsoidal

parameters

are

often

embedded

in

the

mapping

equations

and

their

change

produces

different

plane

coordinates.

o The mapping

equations

given

in

chapter

3

are

accurate

to

the

millimeter,

whereas

promulgated

by

NGS

were

derivatives

of

logarithmic

calculations

with

generally

accepted

approximations.

o The

defining

constants

of

several

zones

have

been

redefined

by

the

States.

o The

numeric

grid

value

of

the

or1g1n

of

each

zone

has

been

significantly

changed

to

make

the

coordinates

appear

clearly

different.

o The

State

plane

coordinates

for

all

points

published

on

NAD

83 by

NGS

will

be

in

metric

units.

o The

SPCS

83

uses

the

Gauss-Kruger

form

of

the

transverse

Mercator

projection,

whereas

the

SPCS

27

used

the

Gauss-

Schreiber

form

of

the

equations.

1.2

SPCS

27

Background

The

State

Plane

Coordinate

System

of

1927 was

designed

in

the

1930s

by

the

U.S.

Coast

and

Geodetic

Survey

(predecessor

of

the

National

Ocean

Service)

to

enable

surveyors,

mappers,

and

engineers

to

connect

their

land

or

engineering

surveys

to

a

common

reference

system,

the

North

American Datum

of

1927.

The

following

criteria

were

applied

in

the

design

of

the

State

Plane

Coordinate

System

of

1927:

2

o Use

of

conformal

mapping

projections.

o

Restricting

the

maximum

scale

distortion

(sec.

2.6)

to

less

than

one

part

in

10,000.

o

Covering

an

entire

State

with

as

few

zones

of

a

projection

as

possible.

o

Defining

boundaries

of

projection

zones

as

an

aggregation

of

counties.

It

is

impossible

to

map

a

curved

Earth

on

a

flat

map

using

plane

coordinates

without

distorting

angles,

azimuths,

distances,

or

area.

It

is

possible

to

design

a

map

such

that

some

of

the

four

remain

undistorted

by

selecting

an

appropriate

"map

projection."

A

map

projection

in

which

angles

on

the

curved

Earth

are

preserved

after

being

projected

to

a

plane

is

called

a

"conformal"

projection.

(See

sec.

2.3.)

Three

conformal

map

projections

were

used

in

designing

the

original

State

plane

coordinate

systems,

the

Lambert

conformal

conic

projection,

the

transverse

Mercator

projection,

and

the

oblique

Mercator

projection.

The

Lambert

projection

was

used

for

States

that

are

long

in

the

east-west

direction

(e.g.,

Kentucky,

Tennessee,

North

Carolina),

or

for

States

that

prefer

to

be

divided

into

several

zones

of

east-west

extent.

The

transverse

Mercator

projection

was

used

for

States

(or

zones

within

States)

that

are

long

in

the

north-south

direction

(e.g.,

Vermont

and

Indiana),

and

the

oblique

Mercator

was

used

in

one

zone

of

Alaska

when

neither

of

these

two

was

appropriate.

These

same

map

projections

are

also

often

custom

designed

to

provide

a

coordinate

system

for

a

local

or

regional

project.

For

example,

the

equations

of

the

oblique

Mercator

projection

produced

project

coordinates

for

the

Northeast

Corridor

Rail

Improvement

project

when a

narrow

coordinate

system

from

Washington,

DC,

to

Boston,

MA,

was

required.

Land

survey

distance

measurements

in

the

1930s

were

typically

made

with

a

steel

tape,

or

something

less

precise.

Accuracy

rarely

exceeded

one

part

in

10,000.

Therefore,

the

designers

of

the

SPCS

27

concluded

that

a

maximum

systematic

distance

scale

distortion

(see

sec.

2.6,

"Grid

scale

factor")

attributed

to

the

projection

of

1:

10,000

could

be

absorbed

in

the

computations

without

adverse

impact

on

the

survey.

If

distances

were

more

accurate

than

1:10,000,

or

if

the

systematic

scale

distortion

could

not

be

tolerated,

the

effect

of

scale

distortion

could

be

eliminated

by

computing

and

applying

an

appropriate

grid

scale

factor

correction.

Admittedly,

the

one

in

10,000

limit

was

set

at

an

arbitrary

level,

but

it

worked

well

for

its

intended

purpose

and

was

not

restrictive

on

the

quality

of

the

survey

when

grid

scale

factor

was

computed

and

applied.

To

keep

the

scale

distortion

at

less

than

one

part

in

10,000

when

designing

the

SPCS

27,

some

States

required

multiple

projection

"zones."

Thus some

States

have

only

one

State

plane

coordinate

zone,

some

have

two

or

three

zones,

and

the

State

of

Alaska

has

1 O

zones

that

incorporate

all

three

projections.

With

the

exception

of

Alaska,

the

zone

boundaries

in

each

State

followed

county

boundaries.

There

was

usually

sufficient

overlap

from

one

zone

to

another

to

accommodate

projects

or

surveys

that

crossed

zone

boundaries

and

still

limit

the

scale

distortion

to

1:

1 o,ooo.

In

more

recent

years,

survey

accuracy

usually

exceeded

1

:10,000.

More

surveyors

became

accustomed

to

correcting

distance

3

observations

for

projection

scale

distortion

by

applying

the

grid

scale

factor

correction.

When

the

correction

is

used,

zone

boundaries

become

less

important,

as

projects

may

extend

farther

into

adjacent

zones.

1.3

SPCS

83

Design

In

the

mid~1970s

NGS

considered

several

alternatives

to

SPCS

83.

Some

geodesists

advocated

retaining

the

design

of

the

existing

State

plane

coordinate

system

(projection

type,

boundaries,

and

defining

constants)

and

others

believed

that

a

system

based

on

a

single

projection

type

should

be

adopted.

The

single

projection

proponents

contended

that.

the

present

SPCS

was

cumbersome,

since

three

projections

involving

127 zones were employed.

A

study

was

instituted

to

decide

whether

a

single

system

would meet

the

principal

requirements

better

than

SPCS

27.

These

requirements

included

ease

of

understanding,

computation,

and

implementation.

Initially,

it

appeared

that

adoption

of

the

Universal

Transverse

Mercator

(UTM)

system

(sec.

2.7)

would be

the

best

solution

because

the

grid

had

long

been

established,

to

some

extent

was

being

used,

and

the

basic

formulas

were

identical

in

all

situations.

However, on

further

examination,

it

was

found

that

the

UTM

6-degree

zone

widths

presented

several

problems

that

might

impede

its

overall

acceptance

by

the

surveying

profession.

For

example,

to

accommodate

the

wider

zone

width,

a

grid

scale

factor

of

1

:2,500

exists

on

the

central

meridian

while

a

grid

scale

factor

of

1:1,250

exists

at

zone

boundaries.

As

already

discussed,

similar

grid

scale

factors

on

the

SPCS

rarely

exceeded

1:10,000.

In

addition,

the

"arc-to-chord"

correction

term

(sec.

2.5)

that

converts

observed

geodetic

angles

to

grid

angles

is

larger,

requiring

application

more

frequently.

And

finally,

the

UTM

zone

definitions

did

not

coincide

with

State

or

county

boundaries.

These

problems

were

not

viewed

as

critical,

but

most

surveyors

and

engineers

considered

the

existing

SPCS

27

the

simpler

system

and

the

UTM

as

unacceptable

because

of

rapidly

changing

grid

scale

factors.

The

study

then

turned

to

the

transverse

Mercator

projection

with

zones

of

2°

in

width.

This

grid

met

the

primary

conditions

of

a

single

national

system.

By

reducing

zone

width,

the

scale

factor

and

the

arc-to-chord

correction

would

be

no

worse

than

in

the

SPCS

27.

The

major

disadvantage

of

the

2°

transverse

Mercator

grid

was

that

the

zones,

being

defined

by

meridians,

rarely

fell

along

State

and

county

boundaries.

A more

detailed

review

showed

that

while

many

States

would

require

two

or

more

zones,

the

2°

grid

could

be

defined

to

accommodate

those

who

wanted

the

zones

to

follow

county

lines.

Furthermore,

seldom

did

this

cause

larger

scale

factor

or

arc-to-chord

corrections

than

in

the

existing

SPCS

27,

although

several

of

the

larger

counties

would

require

two

zones.

However,

the

average

number

of

zones

per

State

was

increased

by

this

approach.

Throughout

this

study,

three

dominant

factors

for

retaining

the

SPCS

27

design

were

evident:

SPCS

had been

accepted

by

legislative

action

in

37

States.

The

grids

had been

in

use

for

more

than

40

years

and

most

surveyors

and

engineers

were

familiar

with

the

definition

and

procedures

involved

in

using

them. Except

for

academic

and

puristic

considerations

the

philosophy

of

SPCS

27

was fundamen-

tally

sound.

With

availability

of

electronic

calculators

and

computers,

little

merit

was found

in

reducing

the

number

of

zones

or

projection

types.

There

was

merit

in

minimizing

the

number

of

changes

to

SPCS

legislation.

For

these

reasons

a

decision

was made

to

retain

the

basic

design

philosophy

of

SPCS

27

in

SPCS

83.

4

The

above

decision

was

expanded

to

enable

NGS

to

also

publ i.sh

UTM

coordi.nates

for

those

users

who

preferred

that

system.

Both

grids

are

now

fully

supported

by

NGS

for

surveying

and mapping

purposes.

It

is

recognized

that

requirements

will

arise

when

additional

projections

may

be

required,

and

there

is

no

reason

to

limit

use

to

only

the

SPCS

83

and

UTM

systems.

1.4

SPCS

83

Local

Selection

The

policy

deci.sion

that

NGS

would

publish

NAD

83

coordinates

in

SPCS

83,

a

system

designed

similar

to

SPCS

27,

was

first

announced

J.n

the

Federal

Register

on

March 24, 1977.

From

April

1978

through

January

1979,

NGS

soli.ci.ted

comments

on

this

published

policy

by

canvassing

member

boards

of

the

National

Council

of

Engineering

Examiners,

all

individual

land

surveyor

members

of

each

board,

the

secretary

of

each

section

and

affiliate

of

the

American

Congress

on

Surveying

and

Mappi.ng

(ACSM),

and

State

and

local

public

agencies

familiar

to

NGS.

As

of

August 1988,

the

1978-79

solicitations

and

responses

to

subsequently

published

articles

had

produced

committees

or

liaison

contacts

in

43

States.

Through

these

people

NGS

presented

the

options

to

be

considered

in

delineation

of

SPCS

83

zones

and

options

in

the

adoption

of

the

defining

mathematical

constants

for

each

zone.

Although

most

States

left

unchanged

the

list

of

counti.es

that

comprised

a

zone,

three

States

(South

Carolina,

Montana,

and

Nebraska)

elected

to

have a

single

zone

cover

the

entire

State,

replacing

what

was

several

zones

on

the

SPCS

27.

In

these

States

the

grid

scale

factor

correction

to

distances

now

exceeds

1:10,000,

and

the

arc-to-chord

correction

to

azi.muths

and

angles

may

become

si.gnifJ.cant.

(See

secs.

2.5

and

4.3.)

A

zone

definition

change

also

occurred

in

New

Mexico

due

to

creation

of

a

new

county,

and

in

California

where zone 7

of

the

SPCS

27

was

incorporated

into

zone 5

of

the

SPCS

83.

Figure

1.

4

depl.cts

the

zone

identiflcation

numbers and

boundaries

of

the

SPCS

83.

In

1982,

NGS

printed

a

map

titled

"Index

of

State

Plane

Coordinate

(SPC) Zone

Codes,"

which

depicts

the

boundaries

and

identification

numbers

of

the

SPCS

27

zones.

Figure

1.4

differs

from

that

map

in

the

following

States

and

Possessions.

CALIFORNIA:

CA

7 No. 0407

was

eliminated,

and

its

area,

the

County

of

Los

Angeles,

included

in

CA

5,

No.

0405.

MICHIGAN:

MI

E No. 2101,

MI

C No.

2102,

and

MI

W No.

2103,

were

eliminated

in

favor

of

the

Lambert

zones.

MONTANA:

MT

N No.

2501,

MT

C No.

2502,

and

MT

S No. 2503 were

eliminated

in

favor

of

a

single

State

zone

MT

No. 2500.

NEBRASKA:

NE

N

No.

2601 and

NE

s No. 2602 were

eliminated

in

favor

of

a

single

State

zone

NE

No. 2600.

SOUTH

CAROLINA:

SC

N No.

3901

and

SC

S 3902 were

eliminated

in

favor

of

a

single

State

zone

SC

No. 3900.

PUERTO

RICO

AND

VIRGIN

ISLANDS:

PR

5201,

VI

5201, and

VI

SX

5202

were

eliminated

in

favor

of

a

single

zone

PR

5200.

5

'

••..

:::1:····

--·~.;

-

.·,,~

_,.

·36()2

:·-.:--

~--

'

--

........

;

04oi··:·

. ' '

....

.

6

-~

- -- .

..

,.,

...

-

...

",_,..,_

~-~

~..:-

·-

·

·--State

Figure

1 4

Plane

Coard.

inate

7

-

-·.

,.

System

of

1983

zones.

Several

States

chose

to

modify

one

or

more

of

the

defining

constants

of

their

zones.

Appendix A

contains

the

defining

constants

for

all

zones

of

the

SPCS

83.

Where

the

constant

differs

from

the

SPCS

27

definition,

it

is

flagged

with

an

asterisk.

Some

of

these

changes

increase

the

magnitude

of

the

grid

scale

factor

and

arc-to-chord

correction

terms.

In

addition

to

the

flagged

changes,

all

"grid

origins

11

are

different

because,

within

the

SPCS

83,

origins

that

were

redefined

are

defined

in

meters.

This

new

grid

origin

was

selected

by

liaison

with

the

States

based

on

the

following

criteria:

o

Keeping

the

number

of

digits

in

the

coordinate

to

the

minimum.

o

Creating

a new

range

for

easting

and/or

northing

in

meters

on

the

1983 datum

that

would

not

overlap

the

range

of

x

and/or

y

in

feet

on

the

existing

1927

datum.

If

an

overlap

could

not

be

avoided,

the

location

of

the

band

of

overlap

(i.e.,

where

the

range

of

x

and/or

yon

the

1927 datum

intersects

with

the

range

on

the

1983

datum)

could

be

positioned

anywhere

through

selection

of

appropriate

grid

origin.

o

Selecting

different

grid

origins

(either

in

northing

or

easting)

for

each

zone

so

the

coordinate

user

could

determine

the

zone

from

the

magnitude

of

the

coordinate.

This

usually

requires

the

nfalse-easting"

to

be

the

smallest

in

the

easternmost

zone

to

avoid

easting

values

close

in

magnitude

for

points

near

boundaries

of

adjacent

transverse

Mercator

zones.

It

requires

the

"false-northing"

of

the

northernmost

zone

to

be

the

smallest

for

adjacent

Lambert

zones

for

the

same

reason.

o

Creating

different

orders

of

magnitude

for

northing

and

easting

to

reduce

the

possibility

of

transition

errors.

The

grid

origin

selection

influenced

only

the

appearance

of

the

coordinate

system,

not

its

accuracy

or

usefulness.

1.5

SPCS

83

State

Legislation

Before

the

NAO

83

project

began,

37

States

had

passed

a

State

Plane

Coordinate

System

Act,

the

first

in

1935.

As

of

August

1988,

42

States

had

legislated

a

"1927

State

Plane

Coordinate

System."

The most

recent

additions

during

the

NAO

83

project

included

Illinois,

New

Hampshire,

North

Dakota,

South

Carolina,

and

West

Virginia.

Of

these

five,

only

Illinois

did

not

simultaneously

include

the

definition

of

the

SPCS

83

within

its

initial

SPCS

27

legislation.

As

of

August 1988,

26

States

had

enacted

1983

State

Plane

Coordinate

System

legislation

(table

1.5).

For

SPCS

83,

as

for

SPCS

27,

NGS

prepared

a model

act

to

implement

SPCS

legislation

by

the

States.

The

act

was

generally

followed

by

the

States

except

for

minor

changes,

some

of

Which

are

discussed

below.

The

model

SPCS

83

act

may

be

found

in

appendix

B.

In

addition

to

providing

mathematical

definitions

of

SPCS

83,

enacted

and

proposed

legislation

contains

other

sections

that

warrant

discussion.

In

the

old

model a

section

stated

that

no

coordinates

11

purporting

to

define

the

position

of

a

point

on a

land

boundary,

shall

be

presented

to

be

recorded

in

any

public

land

records

or

deed

records

unless

such

point

is

within

one-half

mile"

of

8

Table

1.5.--status

of

SPCS

27

and

SPCS

83

legislation

(as

of

August

1,

1988)

NO

SPCS

EXISTING

NAO

27

SPCS

LEGISLATION

ca

States)

(

16

States)

""

NAO

83

"o

Correspondence

NAO

83

corresponaence

1eglslat1on

corresponaence

wlth

leo;ilslatlon

wlth

NGS

drafted

wtth

NGS

reconmendatlons

draftea

(4

States)

(4

States)

(3

States)

(7

States)

(6

States)

Hawal 1

Iowa

Arkansas

Alabama

Colorado{S)

Kansas

Mlsslsslppl

Pennsy1 van

la

Delaware

Massachusetts

Kentucky

•Nebraska••(

SJ

Tennessee

Flor

1aa

New

Jersey

Oklahoma Wyomlno••CSJ

IClaho

New

Me!\

lCO

I

11

lnols

New

York

North

Dakota

wash1no;iton

W1sconsln

•NOTE>

The

only

States

that

authorized

chano;ies

ln

zone

bounoarles

are·

Montana.

Nebraska,

ano

South

Carollna

Cal\fornla.

••NOTE: These

States

have

not

wrltten

leo;i\slatlon,

out

riave

corresoonded

def1nlte

new

SPCS

parameters

to

NGS.

UNITS s •

u.

s.

survey

feet

and

meters

I -

Internat1ona1

feet

and

meters

A11

others

only

meters

ENACTED

NAO

83

SPCS

LECHSLATtON

(26

States)

Alaska

Ar1zona(l)

•Cal\fornla(S)

Connect

1

cut

( S l

Georo1a

1na1<1na(SJ

Lou1s1ana

Malne

Mary1and[S)

M1ch1oan(

I)

Minnesota

M!ssourl

•Montana(

J l

Nevada

New

Ha[ff!St'11re

North

Caroltna{Sl

0010

Oreo;ion( I J

Rhooe

lslana

•Soutn

Carol

Ina(

l)

Soutn

Dakota

Te~as(SJ

utan(

I)

verroont

Vlro;ilnla

West

Vlri;ilnia

a

first-

or

second-order

control

point.

The

new

model

changes

only

the

"one-half

mile

11

to

"1

kilometer,

11

and

references

the

Federal

Geodetic

Control

Committee

(FGCC)

as

the

source

of

the

classifications

of

first-

and

second-order

geodetic

control

points.

The

intent

of

this

section

has

not

been

well

understood.

To

determine

a

boundary

coordinate,

the

act

explicitly

states

that

at

least

a

second-order

monumented

point

must

exist

not

more

than

1

km

away.

It

does

not

say

that

the

second-order

point

must

already

exist.

Adding

that

an

"existing

or

newly

established''

control

point

needs

to

be

within

1

km

may

clarify

this

confusion.

The

intent

was

that

a

property

surveyor

would

either

recover

an

existing

point

or

use

any

survey

methodology

to

establish

a

permanently

monumented

point

of

at

least

second-order,

class

II

accuracy

in

an

accessible

but

protected

location

within

1

km

of

the

property

to

be

surveyed.

Then,

using

this

point,

coordinates

of

the

11

temporarily

11

monumented

(essentially

unmonumented)

property

corners

would be

determined.

These

corners,

if

determined

from a

second-order,

class

II

point,

are

of

third-order

accuracy

( 1 : 1 0,

000),

following

the

usual

practice

of

establishing

the

point

to

the

accuracy

standard.

Another

approach

would

have

been

to

legislate

that

property

coordinates

would

be

determined

using

FGCC

third-order

(1

:10,000)

positional

standards

but

eliminate

the

monwnentation

standards.

This

approach

may

serve

well

with

Global

Positioning

System

methods,

but

it

eliminates

the

nearby

control

point

needed

for

retracement

surveys

by

conventional

means. The 1-km

limit

from monwnented

control

is

perhaps

appropriate

only

for

urban

or

suburban

conditions.

Of

importance

is

not

the

distance,

but

the

existence

of

monumented

control.

Land

values

may

also

affect

the

specifications

for

a

State

or

county.

The

following

examples

illustrate

how

some

States

have

addressed

the

above

requirement

in

their

1983

SPCS

laws.

South

Carolina's

law

states

that

no

point

9

"

can

be

recorded

unless

11

•••

such

point

is

established

in

accordance

with

the

Federal

Geodetic

Control

Committee

specification

for

second-order,

class

II

Virginia's

law

reads

that

no

point

can be

recorded

unless

"

.••

such

point

is

within

2

km

of

a

public

or

private

monumented

horizontal

control

station.

,,

11

established

in

conformity

with

first-

or

second-order

FGCC

specifications.

Minnesota

is

to

be

applauded

for

writing

the

most unambiguous

section.

The law

reads

that

no

point

would be

recorded

unless

"

...

coordinates

have

been

established

in

conformity

with

the

national

prescribed

standards

for

third-order,

class

II

horizontal

control

surveys,

and

provided

that

these

surveys

have

been

tied

to

or

originated

off

monumented

first

or

second-order

horizontal

control

stations,

which

are

adjusted

to

and

published

in

the

national

network

of

geodetic

control

and

are

within

3

km

of

the

said

boundary

points

or

land

corners.

11

The

statement

continues

by

defining

the

national

standards

to

be

those

of

the

FGCC.

Another

debated

portion

of

the

model

SPCS

legislation

involves

the

role

of

coordinates

within

legal

land

descriptions.

The

section

that

states,

11

It

shall

be

considered

a

complete,

legal,

and

satisfactory

description

of

such

location

to

give

the

position

of

the

survey

station

or

land

boundary

corner

on

the

system

of

plane

coordinates

••

,

11

has

been

passed

by many

States.

This

section,

in

conjunction

with

the

previously

discussed

section

dealing

with

the

accuracy

and

recording

of

such

points,

should

be

sufficient

to

permit,

but

not

to

require,

the

use

of

the

SPCS

83

to

describe

real

property

or

supplement

parcel

descriptions.

Often

found

in

the

1927

SPCS

laws,

especially

in

Public

Land

Survey

System

(PLSS)

States,

and

carried

over

to

the

SPCS

83

legislation,

is

a

section

that

specifies

coordinates

are

supplemental

to

any

other

means

of

land

description,

and

in

case

of

conflict

the

conventional

description

shall

prevail

over

the

description

by

coordinates.

This

legislates

an

unconditional

priority

to

the

order

of

evidence

and

could

prevent

the

best

surveyor

from

submitting

sound

boundary

evidence

based

on

coordinates.

There

are

many

who

believe

that

the

intent

should

be

to

evaluate

each

situation

on

its

own

merit

and

not

to

impose an

invariable

rule.

Language

such

as,

11

In

case

of

conflict

between

elements

of

a

description,

cast

out

doubtful

data

and

adhere

to

the

most

certain"

may

suffice.

Perhaps

this

is

currently

being

accomplished

by

common

law.

Several

States

have

addressed

the

above

situation

with

the

statement,

11

•••

the

conventional

description

shall

prevail

over

the

description

by

coordinates

unless

said

coordinates

are

upheld

by

adjudication,

at

which

time

the

coordinate

description

will

prevail."

This,

at

least,

provides

the

opportunity

for

the

competent

land/property

line

surveyor

to

defend

the

use

of

coordinates

in

retracement

surveys.

Pertaining

to

the

use

of

coordinates

on

plats,

States

have

inserted

additional

individual

sections.

Georgia

defines,

"Grid

North"

and

requires

the

convergence

term

on

,,

..•

maps

of

survey

that

are

purported

oriented

to

a

Georgia

Coordinate

System

Zone.

11

Illinois

states

that

plats

of

survey

referencing

the

SPCS

must

indicate

the

zone

and "

...

geodetic

stations.

azimuth,

angles,

and

distances

used

for

establishing

the

survey

connection."

Virginia

added

that

"Nothing

contained

in

this

chapter

shall

be

interpreted

as

preventing

the

use

of

the

Virginia

Coordinate

System

in

any

unrecorded

deeds,

maps,

or

computations.

11

The

last

section

of

most

SPCS

acts

assigns

responsibility

for

the

act

to

a

specific

State

agency.

Our model

law

stated

that

sections

of

the

law

11

could

be

modified

by a

State

agency

to

meet

local

conditions."

Many

States

specifically

1 0

assign

responsibility

to

a

particular

department.

For

example,

the

South

Carolina

Geodetic

Survey

"

••.

shall

maintain

the

South

Carolina

coordinate

system,

files,

and

such

other

maps and

files

as

deemed

necessary

to

make

station

information

readily

available

.•..

11

Similarly,

the

Virginia

Polytechnic

Institute

and

State

University

is

"

.••

the

authorized

State

agency

to

collect

and

distribute

information,"

and

it

authorizes

such

modifications

as

are

referred

to

elsewhere

in

the

law.

NGS

encourages

the

development

of

State

level

surveying

and

mapping

offices.

Responsibility

for

the

States'

geodetic

networks

would be

one

function

of

such

an

office.

The

need

for

SPCS

83

legislation

provides

an

opportunity

to

designate

this

lead

agency.

1.6

SPCS

83

Unit

of

Length

A

Federal

Register

notice

published

jointly

on

July

l,

1959

(24

Fed.

Reg. 5348)

by

the

directors

of

the

National

Bureau

of

Standards

(NBS)

(now

National

Institute

of

Standards

and

Technology)

and

the

U.S.

Coast

and

Geodetic

Survey

refined

the

definition

of

the

yard

in

metric

terms.

The

notice

also

pointed

out

the

very

slight

difference

between

the

new

definition

of

the

yard

(0.9144

m)

and

the

1893

definition

(3600/3937

m), from

which

the

U.S.

survey

foot

is

derived.

The

"international

foot"

of

0.3048

meter

is

shorter

than

the

U.S.

survey

foot

by

2

parts

per

million.

The 1959

notice

stated

that

the

U.S.

survey

foot

would

continue

to

be

used

11

until

such

time

as

it

becomes

desirable

and

expedient

to

readjust

the

basic

geodetic

survey

networks

in

the

United

States,

after

which

the

ratio

of

a

yard,

equal

to

0.911.\4

m,

shall

apply."

Because

the

profession

desired

to

retain

the

U.S.

survey

foot,

and

because

it

is

incorporated

in

legal

definitions

in

many

States

as

well

as

in

practical

usage,

a

tentative

decision

was made

by

NBS

not

to

adopt

the

international

foot

of

0.3048

m

for

surveying

and

mapping

activities

in

the

United

States.

However,

before

reaching

a

final

decision

in

this

matter,

it

was deemed

appropriate

and

necessary

to

solicit

the

comments

of

land

surveyors;

Federal,

State,

and

local

officials;

and

any

others

from among

the

public

at

large

who

are

engaged

in

surveying

and

mapping

or

are

interested

in

or

affected

by

surveying

and

mapping

operations.

NBS

and

NGS

published

their

preliminary

decision

(Federal

Register

Doc.

88-

16174)

and

as

of

this

writing

are

awaiting

comments. A

final

decision

will

be

reached

after

careful

consideration

of

all

the

comments

received.

The

final

decision

will

be

published

in

the

Federal

Register

and

will

be

publicly

announced

in

the

communications

media

as

deemed

appropriate.

Even

if

the

final

decision

affirms

the

preliminary

decision

not

to

adopt

the

international

definition

of

the

foot

in

surveying

and

mapping

services,

it

should

be

noted

that

the

Office

of

Charting

and

Geodetic

Services,

National

Ocean

Service,

NOAA,

in

a 1977

Federal

Register

notice

(42

Fed.

Reg.

15943),

uses

the

meter

exclusively

and

is

providing

the

new

SPCS

83

coordinates

in

meters.

In

the

1927

SPCS

legislation,

the

"foot"

was

the

defining

unit

of

measure,

the

conversion

factor

defined

by

the

"U.S.

survey

foot"

being

implicit.

In

the

States

that

have

prepared

1983

SPCS

legislation,

the

"U.S.

survey

foot"

was

explicitly

stated

as

the

unit

of

measure

when

using

SPCS

27.

If

a

foot

unit

has

been

selected

for

SPCS

83,

it

is

explicitly

written

into

the

SPCS

83

legislation.

11

Most

States

define

a

metric

SPCS

83.

When

the

NAO

83-SPCS 83

publication

policy

was

developed,

and

published

in

the

Federal

Register

on

March

24,

1977,

the

Department

of

Commerce had

established

a

policy

that

the

agency

would

use

metric

units

exclusively.

NGS

concurs

with

the

advantages

of

the

metric

system,

and

except

for

SPCS

27

applications,

has

always

worked

totally

in

metric

units.

Accordingly,

a

metric

SPCS

83 was recommended

to

the

States.

Except

for

Arizona,

States

that

have

enacted

legislation

defined

a

metric

system.

In

addition,

10

States

defined

which

"foot

11

unit

to

use

when

converting

from

meters.

(See

table

1.

5.

)

When

the

metric

grid

origin

of

an

SPCS

83

zone

is

other

than

a

rounded

number,

it

was

derived

from a

rounded

foot

value

using

one

of

the

definitions:

0.3048

m

exactly

international

foot

1200/3937 m

U.S.

survey

foot

1.7

The

New

GRS

80

Ellipsoid

The

mathematics

of

map

projection

systems

convert

point

and

line

data

from

the

ellipsoid

of

a datum

to

a

plane.

Accordingly,

the

dimensions

of

the

ellipsoid

are

an

inherent

part

of

the

conversion

process.

As

discussed

above,

NAO

83

adopted

a

new

ellipsoid,

the

Geodetic

Reference

System

of

1980

(GRS

80).

Therefore,

the

dimensions

of

this

ellipsoid

must be

incorporated

within

any

map

projection

equations,

SPCS

83

or

otherwise,

when

the

requirement

is

to

project

NAO

83

geodetic

data

into

a

plane

system.

Whereas

the

ellipsoid

constants

were

imbedded

within

the

map

projection

equations

promulgated

by

NGS

(and

its

predecessors)

for

the

SPCS

27, and

hence

they

were

invisible

to

the

user,

the

formulation

given

in

chapter

3

requires

entry

of

ellipsoid

constants.

An

ellipsoid

is

formed

by

rotating

an

ellipse

about

its

minor

axis.

For

geodetic

purposes

this

regular

mathematical

surface

is

designed

to

approximate

the

irregular

surface

of

the

Earth

or

portion

thereof.

The

SPCS

27

incorporated

the

defining

parameters

of

an

ellipsoid

identified

as

the

Clarke

spheroid

of

1866,

the

ellipsoid

of

NAO

27.

The

parameters

defining

the

Clarke

spheroid

of

1866 were

the

semimajor

axis

"a"

of

6,378,206,4

m

and

the

semiminor

axis

"b"

of

6,356,583.s

m.

The

ellipsoid

that

forms

the

basis

of

NAO

83,

and

consequently

the

SPCS

83,

is

identified

as

the

Geodetic

Reference

System

of

1980

(GRS

80).

GRS

80

provides

an

excellent

global

approximation

of

the

Earth's

surface.

The

Clarke

spheroid

of

1866,

as

used

for

NAD

27

approximated

only

the

conterminous

United

States.

Because

the

geoid

separation

at

point

MEADES

RANCH

was assumed

equal

to

zero,

a

translation

exists

between

ellipsoids.

The

ellipsoid

change

is

the

major

contributor

of

the

coordinate

shift

from

NAO

27

to

NAO

83.

The

parameters

of

GRS

80

were

adopted

by

the

XVII

General

Assembly

of

the

International

Union

of

Geodesy

and

Geophysics

meeting

in

1979

at

Canberra,

Australia.

Since

only

one

of

the

four

GRS

80

defining

parameters

(semimajor

axis

"a

11

)

is

an

element

of

the

geometric

ellipsoid,

a

second

geometric

constant

(

11

b

11

,

"1

/f,"

or

"e

2

")

must be

derived

from

the

three

GRS

80

parameters

of

physical

geodesy.

Accordingly,

the

geometric

definition

of

the

GRS

Bo

is:

12

a=

6,378,137.

m

(exact

by

definition)

1/f

298.25722210088

(to

14

significant

digits

by

computation)

From

these

two

numbers,

any

other

desired

constants

of

geometric

geodesy

may

be

derived.

For

example,

to

11.!

significant

digits:

b

6,356,752.3141403

e'

0.0066943800229034.

1 3

2.

MAP

PROJECTIONS

A

"projection"

is

a

function

relating

points

on one

surface

to

points

on

another

surface

so

that

for

every

point

on

the

first

surface

there

corresponds

exactly

one

point

on

the

second

surface.

A "map

projection

11

is

a

function

relating

coordinates

of

points

on a

curved

surface

to

coordinates

of

points

on a

surface

of

different

curvature.

The

mathematical

function

defines

a

relationship

of

coordinates

between

the

ellipsoid

and a

sphere,

between

that

sphere

and a

plane,

or

directly

between

the

ellipsoid

and

a

plane.

In

horizontal

surveying

operations,

field

observations

are

collected

on

the

surface

of

the

irregular

nonmathematical

Earth.

For

most

applications,

it

is

more

convenient

to

represent

the

spatial

relationship

between

surveyed

points

by

a

set

of

coordinates,

the

basis

of

which

is

a

regular

mathematical

surface.

Part

of

the

process

of

reducing

field

survey

observations

consists

of

computing

equivalent

values

for

the

survey

observations

from

the

measured

value

on

the

Earth's

surface

to

their

reduced

value

on

the

regular

surface

on

which

one

wishes

to

compute

coordinates.

Because

a

selected

regular

surface

can

only

approximate

the

physical

surface

on

which

the

survey

points

are

actually

located,

the

degree

of

approximation,

and

hence

selection

of

the

regular

surface,

is

usually

a

function

of

the

ultimate

accuracy

requirements

of

the

points.

It

would

not

show

good

judgment

to

perform

difficult

reductions

of

survey

observations

and

place

them on a complex

mathematical

surface

if

the

final

required

accuracy

of

the

points

was

such

as

could

be

achieved

with

more

simple

reducing

procedures.

One

mathematical

surface

traditionally

used

by

surveyors

is

the

local

tangent

plane

with

few,

if

any,

reductions

made

to

the

field

observations.

Resulting

plane

coordinates

from

computations

of

this

nature

are

sufficient

for

independent

projects

of

a

small

extent.

On

the

other

end

of

the

spectrum,

survey

observations

are

often

reduced

to

an

ellipsoid

of

revolution

and

its

associated

datum,

with

subsequent

computations

performed

using

geodetic

coordinates

and

ellipsoidal

geometry.

To

the

majority

of

surveyors

and

engineers,

the

use

of

"map

projections"

provides

a compromise

solution

to

either

of

these

two

extremes.

2.1

Fundamentals

In

the

study

of

map

projections

the

ultimate

surface

on

which

survey

observations

are

reduced

and

on

which

coordinates

are

computed

is

a

plane.

Usually

it

is

a

plane

that

has

been

"developed

11

from

another

regular

mathematical

surface,

as

a

cone

in

Lambert's

conic

projections

or

the

cylinder

in

Mercator's

projections.

Survey

observations

are

"projected

11

or

reduced

to

a

predefined

cylinder

or

cone,

as

is

the

11

graticule"

of

latitude

and

longitude.

The

regular

mathematical

surface

of

the

cylinder

or

cone

is

then

cut

open,

or

"developed,

11

and

laid

flat

into

a

plane.

The

"grid"

of

northings

and

eastings

is

then

overlaid.

Map

projection

systems

provide

a compromise

solution

between

a

limited-in-extent

and

approximate

local

plane

system,

and

performing

ellipsoidal

computations

on

the

geodetic

datum.

Theoretically,

field

observations

are

first

reduced

to

the

ellipsoid

and

then

to

the

map

projection

surface.

But

in

practice

this

is

often

accomplished

as

one

step.

The

conversion

of

angles,

azimuths,

distances,

and

coordinates

between

an

ellipsoid

(GRS

80

for

NAD

83) and

developable

surfaces

is

one

role

of

the

science

of

map

projections.

Figure

2.

1a

illustrates

the

three

basic

projection

surfaces.

S!ngle Point

/---n"Z-,

of

Contacl

1

'

',,

l \

:=~--

::#+==::-

- ' '

' '

' I

: !

PLANE

-----~

'

I

'

' '

'

:i

CONE

...

--·-

-1--,,

_,

---

• Ur>e

ol

.--~-~--·~:_-_-_----~-it

ConlJCI

--

CYLINDER

Una ot

Contaet

Figure

2.1a.--The

three

basic

projection

surfaces.

The

plane,

cone,

or

cylinder

can

be

defined

such

that

instead

of

being

tangent

to

the

datum

surface,

as

illustrated

in

figure

2.1a,

they

intersect

the

datum

surface

as

in

figure

2.1b.

This

11

secant"

type

of

projection,

a

secant

cone

in

Lambert's

projections

and

secant

cylinder

in

Mercator

1

s

projections,

has

been

used

for

SPCS

27

and

SPCS

83,

In

the

Mercator

projection,

the

secant

cylinder

has

been

rotated

90°

so

the

axis

of

the

cylinder

is

perpendicular

to

the

axis

of

rotation

of

the

datum

surface,

hence

becoming

a

"transverse

11

Mercator

projection.

Occasionally

the

cylinder

is

rotated

into

a

predefined

azimuth,

creating

an

"oblique"

Mercator

projection.

Conceptually

this

is

how

one

SPCS

zone

in

Alaska

was

designed.

The

secant

cone

intersects

the

surface

of

the

ellipsoid

along

two

parallels

of

latitude

called

"standard

parallels

11

or

"standard

lines,

11

Specifying

these

two

parallels

defines

the

cone.

Specifying

a

"central

meridian"

orients

the

cone

with

respect

to

the

ellipsoid.

The

transverse

secant

cylinder

intersects

the

surface

of

the

ellipsoid

along

two

small

ellipses

equidistant

from

the

meridian

through

the

center

of

the

zone.

The

secant

cylinder

is

defined

by

specifying

this

central

meridian,

plus

the

desired

grid

scale

factor

on

the

central

meridian.

The

ellipses

of

intersection

are

standard

lines.

Their

location

is

a

function

of

the

selected

central

meridian

grid

scale

factor.

The

specification

of

the

latitude-longitude

of

the

grid

origin

and

the

linear

grid

values

assigned

to

that

origin

are

all

that

remain

to

uniquely

define

a

zone

of

either

the

Lambert

or

transverse

Mercator

projection.

The

above

minimum

specified

values

are

the

"defining

constants"

for

a

single

zone

of

a

projection.

1 5

z

Lambert Conformal

Conic Projection

EIUpsotd

I

B D

I

Transverse

Mercator Projection

EIUpsold

Figure

2.1b.--Surfaces

used

in

State

Plane

Coordinate

Systems.

Figure

2.lb

illustrates

the

Lambert

projection

provides

the

closest

approximation

to

the

datum

surface

for

a

rectangular

zone

greatest

in

east-west

extent,

whereas

the

transverse

Mercator

projection

provides

the

closest

fit

for

an

area

north-south

in

extent.

The

narrower

the

strip

of

Earth's

surface

which

it

is

desired

to

portray

on a

plane,

the

smaller

will

be

the

scale

distortion

designed

in

the

projection.

At a maximum

width

of

254 km, a maximum

grid

scale

factor

of

1

:10,000

will

exist

at

the

zone

boundaries.

This

maximum

grid

scale

factor

was

designed

into

the

SPCS

27

system,

but

in

the

SPCS

83

this

maximum

has

been

exceeded

in

the

States

of

Montana

1

Nebraska,

and

South

Carolina.

2.2

The

SPCS

83

Grid

As

with

SPCS

27

or

any

plane-rectangular

coordinate

system,

the

SPCS

83

is

represented

on a

map

by two

sets

of

uniformally

spaced

straight

lines

intersecting

at

right

angles.

The

network

thus

formed

is

termed

a

11

grid."

One

set

of

these

lines

is

parallel

to

the

plane

of

a

meridian

passing

through

the

center

of

the

grid

1

and

the

grid

line

corresponding

to

that

meridian

is

the

"northing

axis"

of

the

grid.

It

is

also

termed

the

"central

meri.dian"

of

the

grid.

Forming

right

angles

with

the

northing

axis

and

to

the

south

of

the

area

covered

by

the

grid

is

the

easting

axis.

The

point

of

intersection

of

these

axes

is

the

"grid

origin"

of

the

plane

coordinate

system.

The

grid

origin

differs

from

the

"projection

origin"

by

a

constant.

Knowledge

of

the

origin

is

not

required

to

use

SPCS

83.

The

latitude

and

longitude

of

the

grid

origin

are

required

defining-constants

of

a

zone.

The

position

of

any

point

represented

on

the

grid

can

be

defined

by

stating

two

distances,

termed

"coordinates.

11

One

of

these

distances,

known

as

the

11

northing

coordinate,

11

gives

the

position

in

a

northern

direction

from

the

easting

axis.

The

other

distance,

known

as

the

''easting

coordinate,"

gives

the

position

in

an

east

or

west

direction

relative

to

the

northing

axis.

The

northing

coordinates

increase

numerically

from

south

to

north,

the

easting

coordinates

increase

from

west

to

east.

Within

the

area

covered

by

the

grid,

all

northing

coordinates

are

assured

to

be

positive

by

placement

of

the

grid

origin

south

of

the

intended

grid

coverage.

Easting

coordinates

are

made

positive

by

16

assigning

the

grid

origin

of

the

easting

coordinates

a

large

constant.

For

any

point,

the

easting

equals

this

value

adopted

for

the

grid

origin,

often

identified

as

the

"false

easting,

11

plus

or

minus

the

distance

(E')

of

the

point

east

or

west

from

the

central

meridian

(northing

axis).

Some

zones

have

also

assigned

a

"false

northing"

value

at

the

grid

origin.

Accordingly,

the

northing

equals

this

adopted

value

plus

the

distance

of

the

point

north

of

the

easting

axis.

The

linear

distance

between

two

points

on

the

SPCS

83,

as

obtained

by

computation

or

scaled

from

the

grid,

is

termed

the

grid

length

of

the

line

connecting

those

points.

The

angle

between

a

line

on

the

grid

and

the

northing

axis,

reckoned

clockwise

from

north

through

360°,

is

the

grid

azimuth

of

the

line.

The

computations

involved

in

obtaining

a

grid

length

and a

grid

azimuth

from

grid

coordinates

are

by means

of

the

formulas

of

plane

trigonometry.

2.3

Conformality

The commonly

used

examples

of

a

developed

cone

for

the

Lambert

grid

and

a

developed

cylinder

for

the

transverse

Mercator

grid

serve

as

excellent

illustrations

of

the

principles

of

map

projections.

Although

some

projections

are

truly

"perspective,"

for

SPCS

83

the

mathematical

equations

of

the

map

projection

define

the

orderly

system

whereby

the

meridians

and

parallels

of

the

ellipsoid

are

represented

on

the

grid.

Through

the

equations,

controlled

and

computable

distortion

is

placed

into

the

map,

the

unavoidable

result

of

representing

a

spherical

surface

on

a

flat

plane.

If

correct

relative

depiction

of

an

area

is

important,

then

"equal-area"

mapping

equations

are

selected.

If

correct

depiction

of

select

distances

or

azimuths

is

important,

then

other

sets

of

mapping

equations

are

selected.

In

surveying

and

engineering,

correct

depiction

of

shapes

is

important.

This

is

accomplished

by

mathematically

constraining

the

grid

scale

factor

(sec.

2.6)

at

a

point,

whatever

it

may

be,

such

that

it

is

the

same

in

all

directions

from

that

point.

This

characteristic

of

a

projection

preserves

angles

between

infinitesimal

lines.

That

is,

all

lines

on

the

grid

cut

each

other

at

the

same

angles

as

do

the

corresponding

lines

on

the

ellipsoid

for

very

short

lines.

Hence,

for

a

small

area,

there

is

no

local

distortion

of

shape.

But

since

the

scale

must

change

from

point

to

point,

distortion

of

shape

can

exist

over

large

areas.

Furthermore,

for

long

lines

the

angle

on

the

ellipsoid

may

not

exactly

equal

the

angle

on

the

grid.

This

angular

relationship

is

the

property

of

conformality

that

has

been

mathematically

imposed

on

SPCS

27,

SPCS

83,

the

universal

transverse

Mercator

projection,

and

most

projections

used

in

surveying

and

engineering.

Although

angles

converted

from

the

datum

surface

to

the

grid

are

preserved

unchanged

only

for

angles

between

lines

of

infinitesimal

length,

the

angular

difference

of

a

single

direction

between

the

infinitesimal

length

and

a

finite

length

is

a

computable

quantity

identified

as

the

"arc-to-chord"

or

(t-

T)

correction.

(See

secs.

2.5

and

4.3.)

By

numerical

example,

the

reader

may

verify

that

the

distortion

injected

into

the

map

projection

is

an

exactly

defined

and

computable

quantity.

All

too

often

the

concept

of

distortion

in

a

map

projection

system

is

interpreted

as

an

error

of

the

system.

However,

map

projection

systems

provide

for

a

rigorous

mathematical

conversion

of

quantities

between

surfaces,

and,

as

such,

any

inexactness

that

enters

the

conversion

is

caused

by

computational

approximations.

17

2.4

Convergence

Angle

The

construction

of

all

SPCS

grids

is

such

that

geodetic

north

and

grid

north

do

not

coincide

at

any

point

in

a

zone

except

along

the

central

meridian.

This

condition

is

caused

by

the

fact

that

the

meridians

converge

toward

the

poles

while

the

north-south

grid

lines

are

parallel

to

the

central

meridian.

since

geodetic

azimuths

are

referred

to

meridians

and

grid

azimuths

are

referred

to

north-south

grid

lines,

it

is

evident

that

geodetic

azimuths

and

grid

azimuths

must

differ

by

a

certain

amount

that

depends

on

the

position

of

the

point

of

origin

of

the

azimuth

in

relation

to

the

central

meridian

of

the

SPCS

zone.

The

"convergence

angle,"

often

also

identified

as

the

"mapping

angle,"

is

this

angular

difference

between

grid

north

and

geodetic

north.

Defined

another

way,

the

convergence

angle

is

the

difference

between

a

geodetic

azimuth

and

the

projection

of

that

azimuth

on

the

grid.

Convergence

is

not

the

difference

between

geodetic

and

grid

azimuths.

(See

sec.

2.5.)

The

"projected

geodetic

11

azimuth

is

not

the

grid

azimuth.

Geodetic

azimuths

are

symbolized

as

"a"

and

the

convergence

angle

is

symbolized

as

"Y

11

• Note

the

change

from

SPCS

27,

where

the

symbol "8

11

was

used

for

convergence

within

Lambert

projections

and

11

.tio."

for

convergence

within

transverse

Mercator

systems,

to

SPCS

83

where

nyn

represents

convergence

regardless

of

the

projection

type.

2.5

Grid

Azimuth

"t"

and

Projected

Geodetic

Azimuth

11

T"

The

projection

of

the

geodetic

azimuth

11

0:"

onto

the

grid

is

not

the

grid

azimuth,

but

the

"projected

geodetic

azimuth

11

symbolized

as

"T".

Convergence

"Y"

is

defined

as

the

difference

between

geodetic

and

projected

geodetic

azimuths.

Hence

by

definition,

a.=

T+Y,

and

the

sign

of

"Y"

should

be

applied

accordingly.

The

angle

obtained

from two

projected

geodetic

azimuths

is

a

true

representation

of

an

observed

angle.

When

an

azimuth

is

computed from two

plane

coordinate

pairs,

the

resulting

quantity

is

the

grid

azimuth

symbolized

as

"t",

or

sometimes

"a

".

The

g

relationship

between

projected

geodetic

azimuth

11

T" and

grid

azimuth

"t"

is

subtle

and

may

be more

clearly

understood

in

figure

2.5.

The

difference

between

these

azimuths

is

a

computable

quantity

symbolized

as

"6",

or

more

often

as

(t-T).

For

the

purpose

of

sign

convention

it

is

defined

as

6 =

t-T.

For

reasons

apparent

in

figure

2.

5,

this

term

is

also

identified

as

the

"arc-to-

chord"

correction.

Given

the

above

definition

of

a

and

6,

we

obtain

t = a-Y+O.

Sometimes

the

convergence/mapping

angle

is

incorrectly

defined

as

the

difference

between

the

geodetic

azimuth

and

the

grid

azimuth.

This

incorrect

definition

assumes

the

magnitude

of

(t-T)

to

be

insignificant.

While

for

many

applications

that

assumption

may

be

correct,

(t-T)

is

often

considered

a

"second-

term11

correction

to

the

convergence

term.

Whether

identified

as

6, (

t-T),

arc-

to-chord

or

second-term,

the

correction

should

be

understood

and

always

considered.

2.6

Grid

Scale

Factor

at

a

Point

The

grid

scale

factor

is

the

measure

of

the

linear

distortion

that

has

been

mathematically

imposed

on

ellipsoid

distances

so

they

may

be

projected

onto

a

plane.

At a

given

point,

the

ratio

of

the

length

of

a

linear

increment

on

the

grid

to

the

length

of

the

corresponding

increment

on

the

ellipsoid

is

identified

1 8

PROJECTED

MERIDIAN

GEODETIC

NORTH

GRID

NORTH

N

t=a-,,+b

a = GEODETIC AZIMllTH RECKONEO FROM NORTH

T

,.

PROJECTED GEODETIC

AZIMUTH

I & GRID

AZIMUTH

RECKONED FROM NORTH

1' = MAPPING ANGLE=CONVERGENCE ANGLE

2

PROJECTED GEODETIC LINE

~

• 1-T"' SECOND-TERM CORRECTION"' ARC-TO-CHORD CORRECTION

Figure

2.5.--Azimuths.

as

the

grid

scale

factor

at

that

point,

and

symbolized

by

the

letter

"k".

The

grid

scale

factor

is

constant

at

a

point,

regardless

of

the

azimuth,

when

conformal

map

projections

are

used

as

in

the

SPCS

83

and

UTM

systems.

{See

sec.

2.3,

Conformality.)

The

grid

scale

factor

is

variable

from

point

to

point;

mathematics

refers

to

it

as

applying

only

to

infinitesimal

distances

at

a

point.

The

grid

scale

factor

is

equal

to

1.0

along

the

"standard

lines"

(sec.

2.1)

of

the

projection.

Since

the

SPCS

and

UTM

grids

are

secant

type

projections

grid

scale

factors

are

less

than

1,0

for

the

portion

of

the

grid

within

the

standard

lines

and

greater

than

1.0

for

the

remainder

of

the

grid.

In

Lambert

zones,

the

grid

scale

factor

is

less

than

1.0

between

the

two

standard

parallels

that

define

the

zone.

In

transverse

Mercator

zones

the

scale

factor

is

less

than

1.0

between

two

north-south

lines--the

projection

of

the

"ellipse

of

intersection"

{sec.

2.1),

their

distance

from

the

central

meridian

being

a

function

of

the

scale

factor

assigned

to

that

central

meridian

as

part

of

the

zone

definition.

Figure

2.6,

although

exaggerated,

illustrates

this

concept.

Sometimes

grid

scale

factor

is

defined

as

"scale

distortion."

On

a map,

map

scale

is

correct

only

along

the

standard

lines

of

the

projection

on which

the

map

was

cast.

Everywhere

else

on

the

map,

scale

distortion

exists

and

is

defined

as

the

ratio

of

the

map

scale

at

a

given

point

to

the

map

scale

along

a

standard

line.

The

scale

distortion

is

identical

to

the

grid

scale

factor.

In

small

scale

mapping,

scale

distortion

is

often

expressed

as

"scale

error"

in

percent,

where

scale

error

(%)

=

(scale

distortion

minus

1.0)*100.

1 9

Lambert Projection - Cone Secant to Sphere

Defined by Two Standard Parallels and the Origin

Transverse Mercator Projection -

Cylinder Secant

to

Sphere

Defined by Central Meridian and Its Scale

Factor, and the Origin

Figure

2.6.--Scale

factor.

20

2.7

Universal

Transverse

Mercator

Projection

The

zones

of

the

UTM

projection

system

differ

from

the

zones

of

other

transverse

Mercator

projections

by

only

the

zone-defining

constants.

The

basic

mapping

equations

given

in

this

manual

for

the

transverse

Mercator

zones

of

the

SPCS

83

may

be

used

to

obtain

NAO

83

UTM

coordinates

upon

substitution

of

UTM

zone

constants.

The

UTM

zone

constants

have

not

changed,

but

when

the

constants

are

referenced

to

the

GRS

80

ellipsoid

of

NAD

83,

then

1983

UTM

coordinates

will

be

obtained.

The

UTM

specifications,

i.e.,

defining

constants,

on

NAO

27

appear

in

many

manuals

of

the

Department

of

Army,

originator

of

the

system

(e.g.,

Department

of

the

Army

1958).

To

update

the

Department

of

Army

specifications

for

NAO

83

1

only

the

ellipsoid

(

1

'spheroid"

in

the

Army

specifications)

requires

changing.

The 1983

UTM

specifications

for

the

northern

hemisphere

are

as

follows.

Projection:

Ellipsoid:

Transverse

Mercator

(Gauss-Kruger

type)

in

6°

wide

zones

GRS

Bo

in

North

America

Longitude

of

origin:

Latitude

of

origin:

Unit:

Meter

False

northing:

O

Central

meridian

of

each

zone

0°

(the

equator)

False

easting:

500,000

Scale

factor

at

central

meridian:

0,9996

(exactly)

Zone

numbering:

Starting

with

No. 1 on

the

zone from 180°

west

to

174°

west,

and

increasing

eastward

to

No.

60

on

the

zone

from 174°

east

to

180°

east.

(See

fig.

2.7.)

Latitude

limits

Of

system:

0°

to

80°

north

Limits

of

zones:

The

zones

are

bounded by

meridians

whose

longitudes

are

multiples

of

6°

west

or

east

of

Greenwich.

21

:

·.~

······

I

'.;

I··

.:,

j

--

'·

'

.\

--

I :

'1

: r---4'--

L_

' '

..

'·1·

_-:-'-··:

- - i

..

, : .J

/

:1

.

-,

-..

,,.___·;

. I

··-..:..

-:,

..

---1~·'1·

.

-·

-·,

-

_,

; . j

·~

22

,_

-

'--·-

'\,

\l

'

--

"'"

"

~·

·.~~.-~I'.

I

'--

.

"

·"

. I

l

~

:

_.

___

:;. - ..l:...J

I

'

.;_-.-.

J.'

Figure

2.7.--Universal

Transverse

Mercator

zones.

23

3.

CONVERSION

METHODOLOGY

This

chapter

addresses

both

11

manual"

and

"automated

11

methods

for

performing

11

conversions"

on

any

Lambert

conformal

conic,

transverse

Mercator,

or

oblique

Mercator

projections.

Included

is

conversion

from

NAO

83

latitude/longitude

to

SPCS

83

northing/easting,

plus

the

reverse

process.

For

these

processes

this

manual

uses

the

term

11

conversion,"

leaving

the

term

11

transformation

11

for

the

process

of

computing

coordinate

values

between

datums,

for

example,

transforming

from

NAO

27

to

NAO

83

or

transforming

from

SPCS

27

to

SPCS

83.

In

addition

to

converting

point

coordinates,

methods

for

conversion

of

distances,

azimuths,

and

angles

are

also

given.

The

"automated'

1

methods

for

conversions

given

in

sections

3.1

through

3.

3

are

equations

that

have

been

sequenced

and

structured

to

facilitate

programming.

11

Manual" methods

are

generally

a

combination

of

simple

equations,

tables,

and

intermediate

numerical

input,

requiring

only

a

calculator

capable

of

basic

arithmetic

operations.

Section

3.4

provides

such

a manual method

for

the

Lambert

projection

where

the

intermediate

numerical

input

is

polynomial

coefficients.

Table

3.0

summarizes

the

conversion

computational

methods

that

were

used

for

SPCS

27 and

the

methods

discussed

in

this

manual

for

SPCS

83.

Datum

SPCS

27

SPCS

83

Table

3.0.--Summary

of

conversion

methods

Mode

Manual

Automated

Manual

Automated

Projection

Lambert and

Transverse

Mercator

Oblique

Mercator

Lambert,

transverse

Mercator,

and

oblique

Mercator

Lambert

Transverse

Mercator

Oblique

Mercator

Lambert

Transverse

Mercator

Oblique

Mercator

24

Method

Projection

tables

Intersection

tables

Equations

and

constants

described

in

C&GS

Publication

62-4

(Claire

1968)

Polynomial

coefficients

(sec.

3.4)

New

projection

tables

(future)

Automated

only

Polynomial

coefficients

or

new

mapping

equations

(sec.

3. 1)

New

mapping

equations

(sec.

3. 2)

New

mapping

equations

(sec.

3.3)

The

mapping

equations

given

in

sections

3. 1

through

3, 3

are

not

really

"new" and

may

differ

little

from

equations

found

in

geodetic

literature.

However,

they

are

new

in

the

sense

that

they

are

not

in

the

same form

as

the

equations

published

or

programmed by

NGS

or

its

predecessors

in

connection

with

SPCS

27.

Whereas

the

SPCS

27

equations

given

in

C&GS

Publication

62-4

were

designed

to

reproduce

exactly

the

numerical

results

of

an

earlier

manual method

using

logarithmic

computations

and

projection

tables,

the

equations

here

were

designed

for

accuracy

and

computational

efficiency.

The

Gauss-Kruger

form

of

the

transverse

Mercator

equations

was

used

in

SPCS

83

and

the

Gauss-Schreiber

form

in

SPCS

27

equations.

Because

the

mapping

equations

of

the

automated

approac~

apply

equally

to

main-

frame

computers

and programmable

hand-held

calculators,

the

availability

of

sufficient

significant

digits

warrants

consideration.

The

equations

of

transverse

and

oblique

projections

as

given

here

will

produce

millimeter

accuracy

on any

machine

handling

10

significant

digits.

For

the

Lambert

projection,

the

method

of

polynomial

coefficients

(sec

3.4)

was

developed

for

machines

with

only

10

significant

digits.

With

less

than

12

digits,

the

general

mapping

equations

could

not

guarantee

millimeter

accuracy

in

all

Lambert

zones,

particularly

in

Florida,

Louisiana,

Texas,

South

Carolina,

Nebraska,

and

Montana. However,

the

polynomial

coefficient

method

may

also

prove

to

be

the

most

efficient

for

any

machine.

The

general

mapping

equations

will

produce

submillimeter

accuracy

when

adequate

significant

digits

are

available

for

the

computation.

Since

the

equations

are

not

difficult,

the

polynomial

coefficient

method

also

fills

the

requirement

for

a manual method

for

the

Lambert

projection.

A manual

method

for

the

SPCS

83

transverse

Mercator

projections

has

not

been

fully

developed

by

NGS

pending

the

demonstrated

requirement

for

such

a

method.

While

it

is

easy

to

visualize

map

projections

by

considering

them a

perspective

projection

of

the

meridians

and

parallels

of

the

datum

surface

onto

a

surface

that

develops

into

a

plane,

in

this

age

of

coordinate

plotters

a

graticule

is

generally

not

constructed

by

these

means.

Although

a

set

of

mechanical

procedures

can

sometimes

be

defined

by

which

meridians

and

parallels

can

be

geometrically

constructed

on

the

grid

using

a

ruler,

compass,

and

scale,

a

pair

of

functions,

N = f

1

{¢,A)

and

E = f

2

(¢,A),

always

exist.

That

is,

for

a

point

of

given

latitude

(¢)

and

longitude

(A),

there

exist

equations

to

yield

the

northing

coordinate

and

equations

to

yield

the

easting

coordinate

when ¢

and

A

are

substituted

into

the

equations.

Likewise,

equations

must

exist

to

compute

the

convergence

angle,

Y = f

3

(¢,A),

and

grid

scale

factor,

k =

f~(¢,A).

These

four

functions,

or

equations,

comprise

the

direct

conversion

process.

Furthermore,

it

must be

possible

to

perform

the

inverse

computation,

requiring

another

pair

of

formulas,

latitude

(¢)

= f

5

(N,E)

and

longitude

(A)

= f

6

(N,E).

Similarly

needed

are

convergence

and

grid

scale

factor

as

a

function

of

the

plane

coordinates,

Y = f

7

(N,E)

and

k = f

6

(N,E).

Because

these

are

one-to-one

mappings,

the

inverse

computation

must

reproduce

the

original

values.

This

chapter

provides

these

eight

11

mapping

equations"

for

each

of

these

projections:

Lambert

conformal

conic

(sec.

3.1),

transverse

Mercator

(sec.

3.2),

and

oblique

Mercator

{sec.

3.3),

For

each

projection,

the

definition

of

the

adopted

symbols

will

be

given

first.

Two

sets

of

symbols

are

listed,

the

conventional

set

which

incorporates

the

Greek

alphabet

and

a

set

available

on

standard

keyboards.

The

equations

in

this

chapter

will

use

the

conventional

notation.

The

entries

in

the

notation

section

flagged

with

an

asterisk

are

the

constants

required

to

uniquely

define

one

specific

zone

of

that

general

type

of

25

map

projection.

The

values

of

those

zone-defining

constants

as

adopted

and

legislated

by

the

States

are

listed

in

appendix

A.

Included

within

the

notation

section

are

the

symbols

and

definition

of

ellipsoid

constants.

Although

several

geometric

ellipsoid

constants

are

used

within

the

mapping

equations,

only

two

geometric

constants

are

required

to

define

an

ellipsoid.

The

SPCS

83

uses

the

GRS

80

ellipsoid.

Those

constants

are

discussed

in

section

1.7.

All

other

geometric

ellipsoid

constants

are

then

derived

from

the

two

defining

constants,

usually

for

the

purpose

of

eliminating

repeated

computations.

A

section

on

computation

of

zone

constants

follows

each

section

on

notation

and

definitions.

Within

this

section

are

equations

to

compute

intermediate

quantities

derived

from

the

zone-defining

constants

of

appendix

A.

These

need

only

to

be

derived

once.

The

derived

"intermediate

computing

constants"

of

this

section

that

need

to

be

saved

for

future

computations

are

flagged

with

an

asterisk.

The

advantage

of

segmenting

the

general

mapping

equations

is

to

eliminate

repeated

computations.

Subsequent

sections

under

each

projection

type

list

the

equations

of

the

direct

and

inverse

coordinate

conversion

process.

The

equations

for

the

(t-T)

line

correction

term

(see

also

sec,

4.3)

are

provided

in

a

final

section.

The

solution

of

the

ultimate

mapping

equations

will

require

the

values

of

the

asterisked

terms

of

the

first

two

sections

(defining

constants

plus

intermediate

constants).

3.1 Lambert

Conformal

Conic

Mapping

Equations

3.11

Notation

and

Definitions

For

some

terms

an

optional

symbol

appears

in

parentheses.

This

optional

symbol

available

on

all

keyboards

is

used

exclusively

in

section

3.4

and

appendix

C.

Asterisked

terms

define

the

projection.

Their

values

are

listed

in

appendix

A.

These

terms

are

the

"zone

defining

constants

11

included

within

State

SPCS

legislation

where

enacted.

<P

*

•s

*

•n

<P

0

*

<Pb

A

*

Ao

k

k,,

k,

y

6

N

*

Nb

N,

(

8)

(BS)

(B

)

n

(Bo)

(Bb)

( L)

(Lo)

(

c)

(t-T)

Parallel

of

geodetic

latitude,

positive

north

Southern

standard

parallel

Northern

standard

parallel

Central

parallel,

the

latitude

of

the

true

projection

origin

Latitude

of

the

grid

origin

Meridian

of

geodetic

longitude,

positive

west

Central

meridian,

longitude

of

the

true

and

grid

origin

Grid

scale

factor

at

a

general

point

Grid

scale

factor

of

a

line

(between

point

1 and

point

2)

Grid

scale

factor

at

the

central

parallel

$

0

Convergence

angle

Arc-to-chord

or

second-term

correction

Northing

coordinate

(formerly

y)

The

northing

value

for

~b

at

the

central

meridian

(the

grid

origin).

Sometimes

identified

as

the

false

northing.

Northing

value

at

the

intersection

of

the

central

meridian

with

the

central

parallel

(the

true

projection

origin)

26

*

E

E,

R

Rb

R,

K

Q

a

b

f

e

Easting

coordinate

(formerly

x)

The

easting

value

at

the

central

meridian

A

0

•

Sometimes

identified

as

the

false

easting

Mapping

radius

at

latitude

¢

Mapping

radius

at

latitude

¢b

Mapping

radius

at

latitude

¢

0

Mapping

radius

at

the

equator

Isometric

latitude

Semimajor

axis

of

the

geodetic

ellipsoid

Semiminor

axis

of

the

geodetic

ellipsoid

Flattening

of

the

geodetic

ellipsoid

=

(a-b)/a

First

eccentricity

of

the

ellipsoid

=

(2f-fz)

112

3.12

Computation

of

Zone

Constants

In

this

section

the

zone

defining

constants,

ellipsoid

constants,

and

parts

of

the

Lambert

mapping

equations

are

combined

to

form

several

intermediate

computing

constants

that

are

zone

specific.

These

intermediate

constants,

flagged

with

an

asterisk,

will

be

required

within

the

working

equations

of

sections

3.13

through

3.15.

All

angles

are

in

radian

measure

where

1

radian

equals

180/11

degrees.

Linear

units

are

identical

to

the

units

of

the

ellipsoid

(a

and

b)

and

grid

origin

(Nb

and

E

0

).

+

sin

cps

sin

cps

e

~n

,__•_e_s~i-n-,.<1>~

e

sin

.+.

o/

s

Similarly

for

Qn' W

0

, Qb, Q

0

,

and

W

0

upon

substitution

of

the

appropriate

latitude

where

*

sin

¢

0

in

[Wncos ¢

5

/(W

5

cos

¢

0

)]

Qn

Qs

a

cos

¢

exp(Q

sin¢

)

s s 0

* K

x

exp(x)

= £

W

sin

¢

0

s

a

cos

¢ exp(Q

sin

¢

0

)

n n

£ = 2.718281828

.••

(the

base

of

natural

logarithms)

*

27

*

R,

K/exp(Q

0

sin

¢

0

)

(R

0

used

in

0

computation)

*

k,

*

3,13

Direct

Conversion

Computation

This

computation

starts

with

the

geodetic

coordinates

of

a

point

(¢,A)

from

which

the

Lambert

grid

coordinates

(N,E)

are

to

be

computed,

with

convergence

angle

(Y),

and

grid

scale

factor

(k).

Q

R

y

N

E

1

2

[

+

sin

¢ + e

sin

¢]

tn

--~-~

- e

.2.n

-----~.

-

sin

¢ - e

sin

~

K/exp(Q

sin

¢a)

0,

-

A)

sin

¢

0

Rb

+

Nb

- R

cos

y

E,

+

R

sin

y

3.1~

Inverse

Conversion

Computation

In

this

computation

the

Lambert

grid

coordinates

of

a

point

(N,E)

are

given

and

the

geodetic

coordinates

(¢,A),

convergence

(Y),

and

grid

scale

factor

(k)

are

to

be

computed.

RI

Rb

- N +

Nb

E

1

E - E

0

y

tan-

1

(E'/R')

A

A, -

Y/sin

¢1

0

R

(RI

2

+

E,.._)1/2

Q

[tn(K/R)]/sin

.,.

Computation

of

latitude

is

iterative.

Sin

¢i

Starting

with

the

approximation

exp(2Q)

-

exp(2Q)

+ 1

28

solve

for

sin

¢

three

times,

as

follows:

r,

f,

2

+

sin

cp

sin

¢i

- e

tn

e'

+ e

sin

...

]

c--~-"7'~:

- Q

- e

sin

'I'

Add

a

correction

of

(-f

1

/f

2

)

to

sin

cp

and

iterate

two

times

before

obtaining

¢.

k =

(1

- e

2

sin

2

¢i)

112

(R

sin

¢

0

)/(a

cos¢).

Latitude

can

also

be

obtained

without

iteration

as

shown

in

computations

for

the

oblique

Mercator

projection.

Four

additional

ellipsoid

constants

required

for

this

alternative,

F

0

,

F

2

,

F

4

,

F

6

,

are

computed

in

section

3,32.

If

only

k

is

desired

from

the

grid

coordinates,

an

approximate

¢will

suffice

and

its

computation

shortened.

After

computing

Q,

compute

sin

6

= exp(ZQ) - 1

exp(2Q)

+ 1

¢i

= 6

+(A

sin

6

cos

6)(1

+ B

cos

2

e)

in

which

A=

e

2

(1

- e

2

/6)

and

B =

7e

2

/6.

For

the

GRS

80

ellipsoid

A

and B

=

0.0078.

The

grid

scale

factor

may

be

approximated

by

the

equation

0.0066869

The

quantity

r

0

is

defined

in

section

3,15,

Values

of

r

0

,

k

0

and N

0

for

each

zone

are

given

in

appendix

C.

A

further

approximation

is

given

by

the

equation:

I 4 2 2

k = k

0

+ (N-N

0

)

2

(1.231

X 10 ) +

(N-N

0

)

3

(tanif>

0

)(6.94

X 10

).

These

approximations

may

not

be

sufficiently

accurate

in

the

States

with

a

single

Lambert

zone.

To

derive

the

grid

scale

factor

at

a

point

directly

from

the

grid

coordinates,

the

method

given

in

section

3.4,

the

method

of

polynomial

coefficients,

also

warrants

consideration.

3.15

Arc-to-Chord

Correction

"0"

(alias

"t-T

11

)

(see

also

sec.

4.3.)

The

relationship

among

grid

azimuth

(t),

geodetic

azimuth

(a),

convergence

angle

(Y),

and

arc-to-chord

correction

(0)

at

any

given

point

is

t = a - Y +

O.

29

To

compute

6

requires

knowledge

of

the

coordinates

of

both

ends

of

the

line

to

which

6

is

to

be

applied.

If

geodetic

coordinates

of

the

endpoints

are

available

(¢

1

,

A

1

and

¢

2

,

A

2

)

1

the

6 from

point

1

to

point

2

can

be

computed

from

where

¢

3

=

(2¢

1

+ ¢

2

)/3

and

¢

0

is

the

computed

constant

for

the

zone.

practice,

however,

6

is

desired

as

a

function

of

the

grid

coordinates.

end

the

following

sequence

of

equations

will

produce

the

best

possible

determination

of

6

12

,

given

points

N

1

,

E

1

and

N

2

, E

2

:

In

normal

To

that

p,

N'

-

N,

p,

N,

-

N,

q,

E,

-

E,

q,

E,

-

E,

R'

R,

-

p,

R'

R,

-

p,

'

""

N,

-

N'

M,

k,a

( 1

-

e

2

)/(1

-

e2sin2¢0)3/2

NOTE:

M

0

is

the

scaled

radius

of

curvature

in

the

meridian

at

¢

0

scaled

to

the

grid.

The

value

of

M

0

for

each

zone

appears

in

appendix

3

as

a

"computed

constant."

( 1 )

For

most

applications

a

less

accurate

det.ermination

of

¢

will

suffice.

For

example,

the

original

Coast

and

Geodetic

Survey

formula

(Adams

and

Claire

1948:

p.

13)

should

be

adequate

for

all

applications

except

the

most

precise

surveys

in

the

largest

Lambert

zones.

(

2)

6E:E

2

-E

1

The

quantity

r

0

is

the

geometric

mean

radius

of

curvature

at

¢

0

scaled

to

the

grid

and

is

constant

for

any

one

zone.

The

value

of

r

0

for

each

zone

has

been

included

with

the

computed

constants

in

appendix

C. A

single

value

of

1/(2

r

0

2

)

is

often

used

and

combined

with

the

constant

to

convert

radians

to

seconds

(1

radian

648000/n

seconds).

"

Hence,

6

12

=

25.4

(p

1

+

6N/3)(6E)10

seconds,

where

the

coordinates

are

in

meters.

Sometimes

the

notation

(~N)

replaces

(p

1

+

6N/3).

Then

the

above

equation

is

analogous

to

the

expression

often

used

in

connection

with

NAD

27:

2.36

llx

6y

10

seconds

30

where

the

coordinates

are

in

feet.

This

expression

also

serves

for

NAO

83

coordinates

that

have been

converted

to

feet.

For

the

SPCS

27

Lambert

systems

NGS

suggested

two

other

appropriate

methods

that

provided

more

accurate

(t-T)

corrections

at

the

zone

extremities.

One

was

similar

to

equation

3.15

(1)

and

gave

essentially

the

same

results.

Since

the

computing

effort

was

somewhat

greater

than

for

3.

15

(1)

it

is

not

given

here.

The

second,

while

not

as

accurate

as

3.15(1),

may

be

simpler

for

manual

calculations

because

it

uses

the

SPCS 83

zone

constants

and

readily

understood

rotation

and

translation

formulas.

where

n

= D + E'

sinY

+

N'

COSY

e =

E'

COSY

-

N'

sinY

(in

radian

measure)

11

Y

11

is

the

average

convergence

angle

for

the

survey

area

and

is

considered

positive.

Y

to

minutes

is

sufficient.

D =

2R

0

sin

2

Y/2

N'

N - N

0

E'=E-E

0

The

size

of

6

varies

linearly

with

the

length

of

the

6E

(6A) component

of

the

line

and

With

the

distance

of

the

standpoint

from

the

central

parallel.

It

does

not

vary

with

distance

of

standpoint

from

the

central

meridian.

Hence

the

size

of

6

depends

on

the

direction

of

the

line,

varying

from a

zero

value

between

points

on

the

same

meridian

to

maximum

values

over

east-west

lines.

Table

3.1

gives

an

overview

of

the

true

numeric

value

of

the

arc-to-chord

correction

(6)

and

of

the

computational

errors

expected

from

equations

(1)

and

(2).

The

examples

were

computed

for

a

hypothetical

zone

with

central

parallel

of

approximately

42°

(standard

parallels

41°

and

43°),

on

the

GRS

80

ellipsoid.

Two

cases,

1°

and

2°,

are

illustrated

for

the

distance

of

the

standpoint

from

the

central

parallel

¢

0

•

Two

cases,

5°

and

10°,

are

given

for

the

distance

of

the

standpoint

from

the

central

meridian.

Although

the

magnitude

of

C

is

not

a

function

of

the

distance

of

the

standpoint

from

the

central

meridian,

equation

(2)

becomes

less

accurate

as

this

distance

increases.

Table

3.1

also

gives

three

cases

for

the

orientation

of

the

line.

in

azimuths

of

90°,

135°,

and

180°.

Again

note

that

although

the

true

6

equals

zero

in

an

azimuth

of

180°,

the

equations

only

approximate

zero.

The

final

assumption

in

table

3.1

is

that

the

length

of

the

line

for

which

C

is

being

computed

is

20

km.

Dividing

the

line

into

several

traverse

legs

results

in

a

proportional

decrease

in

the

required

correction

to

a

direction.

It

does

nothing

to

diminish

the

closure

error

in

azimuth

because

errors

due

to

omission

of

6

are

cumulative.

From

data

given

in

table

3,

1

the

persons

performing

the

computing

must

decide

which

reduction

formula

is

appropriate

for

their

needs,

remembering

that

the

accuracy

of

the

formula

should

exceed

the

expected

accuracy

of

the

field

work by

one

order

of

magnitude

and

that

an

error

of

1"

in

direction

corresponds

to

a

linear

error

of

about

1

:200,000,

or

5 ppm.

31

Table

3.1.--True

values

of

(t-T)

and

computational

errors

in

their

determination

(in

seconds

of

arc)

¢,

-

¢,

1 ,

2'

1 0

20

! '

-

!,

50

10°

Azimuth

90°

90° 90°

True 6

5.67

11

•

44

5.67

11

•

44

Error

by

( 1 )

0.00

0.02

0.03

0.

11

Error

by

( 2)

0.53 0.38

2.28

2.07

Azimuth

1

35

° 1

35

° 135°

1

35

°

True

6

3.83

7.

91

3.83

7.

91

Error

by

( 1 )

o.oo

0.

01

0.02

0.08

Error

by

( 2)

0.

1 4

-0.20

0.99

0.38

Azimuth

180

°

180° 180°

180°

True

6

0.00

Error

by

(

1)

0.00

Error

by

( 2)

-0.34

-0.67

-0.90

-1.

55

3.2

Transverse

Mercator

Mapping

Equations

3.

21

Notation

and

Definitions

Asterisked

terms

define

the

projection

for

which

values

are

given

in

appendix

A.

These

zone

specific

11

defining

constants

11

are

included

within

State

SPCS

legislation

where

enacted.

¢

Parallel

of

geodetic

latitude,

positive

north

A

Meridian

of

geodetic

longitude,

positive

west

w

Rectifying

latitude

N

Northing

coordinate

on

the

projection

(formerly

y)

E

Easting

coordinate

on

the

projection

(formerly

x)

* A

0

Central

meridian

* E

0

False

easting

(value

assigned

to

the

central

meridian)

S

Meridional

distance

*

¢,

* N,

s,

*

k,

k

k,,

hN

hE

E'

y

6"

a

b

f

e'

Latitude

of

grid

origin

False

northing

(value

assigned

to

the

latitude

of

grid

Meridional

distance

from

the

equator

to

¢

01

multiplied

central

meridian

scale

factor

Grid

scale

factor

assigned

to

the

central

meridian

Grid

scale

factor

at

a

point

Grid

scale

factor

for

a

line

(between

points

1

and

2)

N

2

-

N

1

E

2

-

E

1

E - E

0

Meridian

convergence

Arc-to-chord

correction

(t-T)

(from

point

1

to

point

2)

Semimajor

axis

of

the

ellipsoid

Semiminor

axis

of

the

ellipsoid

Flattening

of

the

ellipsoid

=

(a

-

b)/a

First

eccentricity

squared

=

(a

2

-

b

2

)/a

2

32

2r

- r

2

origin)

by

the

e

12

Second

eccentricity

squared=

(a

2

-

b

2

)/b

2

= e

2

/(1

- e

2

)

n

(a

-

b)/(a

+

b)

=

f/(2

-

f)

R

Radius

of

curvature

in

the

prime

vertical

=

a/(1

- e

2

sin

2

¢)

112

r

0

Geometric

mean

radius

of

curvature

scaled

to

the

grid

r

Radius

of

the

rectifying

sphere

t

tan

¢

(secs.

3,23

and

3.24)

t

grid

azimuth

(sec.

3.25

and

others)

TJ

2

e'

2

cos

2

¢

3.22

Constants

for

Meridional

Distance

In

this

section

nine

ellipsoid

specific

constants

and

one

zone

specific

intermediate

computing

constant

are

derived.

These

intermediate

constants,

flagged

with

an

asterisk,

will

be

required

within

the

working

equations

of

sections

3.23

through

3.26.

The

following

ellipsoid

specific

constants

may

be

directly

entered

into

software.

The

equations

are

given

for

those

with

requirements

for

other

ellipsoids.

• r

u,

a(1

-

n)(1

- n

2

)(1 + 9n

2

/4

+ 225n

..

/64)

-3n/2

+ 9n

3

/1

6

6367449.14577

m

(GRS

80)

15n

2

/16

- 15n

..

/32

-35n'/48

315n"/512

* U

0

2(u

2

-

Zu,.

+ 3u

6

-

4u

8

)

* U

2

8(u~

- 4u

6

+ 10u

8

)

*

U.,

32(u

6

-

6u

8

)

* u 6 1 28 us

v

2

~

3n/2

- 27n

3

/32

21n

2

/16

-

55n"/32

151n

3

/96

v,

• v 0

• v,

•

v,

1 097n "/51 2

2(v

2

-

2v., +

3v

6

-

4v

8

)

8(v.,

-

4v

6

+ 10 V

8

)

32{v

6

-

6v

0

)

128v

8

-0.00504

82507

76

(GRS

BO)

0.00002

12592 04

(GRS

80)

-0.00000

01114 23

(GRS

80)

0.00000

00006 26

(GRS

80)

0.00502

28939 48

(GRS

80)

0.00002

93706

25

(ORS

80)

0.00000

02350 59

(GRS

80)

0.00000

00021

81

(GRS

80)

The

following

meridional

constant

is

a

zone

specific

constant

computed

once

for

a

zone.

Table

3.22

contains

S

0

for

each

SPCS

83

transverse

Mercator

zone.

Wo

¢0

+

sin

¢0

cos

¢o(Uo +

UzCOS

2

¢o

+ U.,cos"$o +

UsCOS

6

¢o)

* S

0

k

0

w

0

r

3.23

Direct

Conversion

Computation

The

following

computation

starts

with

the

geodetic

coordinates

of

a

point

(¢,

A)

from which

the

transverse

Mercator

grid

coordinates

(N,E),

convergence

angle

(Y),

and

the

grid

scale

factor

(k)

are

computed.

All

angles

are

in

radian

measure

where

one

radian

equals

180/~

degrees.

Linear

units

match

the

units

of

the

ellipsoid

and

false

origin.

33

Table

3.22.--Intermediate

constants

for

transverse

Mercator

projections

State-zone-

code

AL-E-01

01

AL-W-0102

AK-2-5002

AK-3-5003

AK-4-5004

AK-5-5005

AK-6-5006

AK-7-5007

AK-8-5008

AK-9-5009

AZ-E-0201

AZ-C-0202

AZ-W-0203

DE---0700

FL-E-0901

FL-W-0902

GA-E-1

001

GA-W-1002

HI-1-5101

HI-2-5102

HI-3-51

03

HI-4-5104

HI-5-5105

ID-E-1101

ID-C-11 02

ID-W-1103

IL-E-1201

State-zone-

s,

1/(2

r

0

2

)*

code

s,

(m)

( 1 0 l

..

)

(m)

3,375,406.7112

1.23256

IL-W-1202

4,

056,

280.6721

3,319,892.0570

1.

23262

IN-E-1301

4,

151'863.

7425

5,

985,

317.

4367

1.22473

IN-W-1302

4,

151,863.

7425

5,985,317.4367

1.

22473

ME-E-1801

4,

836,

302.

3615

5,985,317.4367

1.22473

ME-W-1802

4,744,046.5583

5,

985,

317.

4367

1.

22473 MS-E-2301

3,264,526.0416

5,985,317.4367

1.

22473 MS-W-2302

3,264,526.0416

5,

985,

317.

4367

1.

22473

MO-E-2401

3,966,785.2908

5,

985,

317.

4367

1.22473

MO-C-2402

3,

966,

785.

2908

5,

985,

317.

4367

1.

22473 MO-w-2403

4,003,800.5632

3,

430,

631.

2260

1.

23244 NV-E-2701

3,

846,

473.

6437

3,

430,

631.

2260

1.

23244

NV-C-2702

3,846,473.6437

3,430,745.5918

1.

23236

NV-W-2703

3,846,473.6437

4,207,476.9816

1.

23083

NH---2800

4,707,019.0442

2,692,050.5001

1.

23387

NJ---2900

4,299,571.6693

2,692,050.5001

1.

23387

NM-E-3001

3, 430,

662.

41

67

3,319,781.3865

1.

23271

NM-C-3002

3.430,631.2260

3,319,781.3865

1.

23271

NM-W-3003

3,

430,

688.

4089

2,083,

150.1655

1.

23570

NY-E-3101

4,299,571.6693

2,249,

193.4045

1.

23532

NY-C-3102

4,

429,

252.

1847

2,341,506.4725

1

.23527

NY-W-3103

4,429,252.1847

2,415,321.4658

1.

23507

RI---3800

4,

549,

799.

41 41

2,396,891.1333

1.

23505

VT---4400

4,707,007.8366

4,614,370.6555

1.

22980

WY-E-4901

4,484,768.4357

4,

614,

370.

6555

1 •

22952

WY-EC-4902

4,484,768.4357

4,614,305.8890

1.

22926

WY-WC-4903

4,484,768.4357

4,059,417.9793

1.

23060

WY-W-4904

4,

484,

768.

4357

L

"'

(A

- A

0

)

cos

¢

Note:

The

sign

convention

used

in

SPCS

21 was

0,

-

•).

Suggestion:

Use

nested

form.

S k

0

w r

R k

0

a/(1

- e

2

sin

2

¢)

112

34

1/(2

r

0

2

)*

( 1 0 I

'+)

1.

23068

1 . 23062

1.

23062

1.

22906

1.22918

1.

23258

1.

23258

1.

23126

1.23126

1.

231

24

1.23106

1.

231

06

1.23106

1.

22976

1.

23078

1 • 23242

1.

23244

1.

23240

1.

22992

1.

22983

1.22983

1.

22998

1.

22948

1 . 22983

1.

22983

1.

22983

1.

22983

A,

12

[5

- t

2

+ n

2

(9

+

1ln

2

)J

A,

1

[

61

-

58t

2

+

t'

+

11

2

(270

-

330t')]

360

N

s -

s,

+

N,

+ A

2

L

2

[1

+ L

2

(A

..

+ A

6

L

2

)]

A,

-R

A,

2_

( 1

6

-

t'

+

n2)

A,

1

[5

-

18t

2

t'

+

11

2

(14

-

S8t

2

)J

+

120

1

A1

=

SOlJO

(61 -

479t

2

+

179t"

- t

6

)

c,

- t

l (1 +

311

2

+

2n")

3

F,.

=

~

2

[5

-

4t

2

+ n

2

(9 -

24t

2

)]

The A

6

,

A

7

,

C

5

,

and

F

..

terms

are

negligible

approximate

boundaries

of

the

SPCS

83

zones.

defined

SPCS

83

boundaries

and

to

compute

UTM

these

terms

should

be

evaluated.

3.21l

Inverse

Conversion

Computation

when

computing

within

the

To

use

the

SPCS

83

beyond

the

coordinates,

the

significance

of

This

computation

starts

with

the

transverse

Mercator

grid

coordinates

of

a

point

(N,E) from

which

the

geodetic

coordinates

(¢,A),

convergence

angle

(Y),

and

grid

scale

factor

(k)

are

computed.

35

(This

is

sometimes

referred

to

as

the

11

footpoint

latitude.

11

)

Suggestion:

Use

nested

form.

Q

B,

[5

+

3t

2

+ n

2

(1 -

9t

2

) - 4n

"]

12

f f f f

B,

[5 +

28t

2

+ 24t

..

+ n

2

(6 +

Btr'll

120

f f f

L Q[ 1

+

Q2{83

+

Q2(B5

+

B,Q'))]

'

Ao

-

L/cos

>r

D,

tf

1

, -

n

2

-

2n

..

)

D,

- - ( 1 + t

3

f

f f

D, 1

(2

+

5tf

,

+

3t

..

)

15

f

y

D,Q[1

+

Q2(Ds

+

DsQ2)]

G,

1

( 1

+ n

2)

2 f

c

..

=

~

2

(1 +

5nf

2

)

36

The 8

6

,

8

7

,

0

5

,

and

G~

terms

are

negligible

approximate

boundaries

of

the

SPCS

83

zones.

83

boundaries

and

to

compute

UTM

coordinates,

evaluated.

when

using

To

compute

the

use

of

the

SPCS

83

within

the

beyond

the

defined

SPCS

these

terms

should

be

For

most

requirements

the

point

grid

scale

factor

k

may

be

determined

from

the

approximation:

where

r

0

is

the

geometric

mean

radius

of

curvature

scaled

to

the

grid

defined

in

section

3.15,

and

evaluated

at

the

mean

latitude

of

the

zone.

Table

3.22

contains

(1/2r

0

2

)

for

each

of

the

transverse

Mercator

zones.

3.25

Arc-to-Chord

Correction

(t-T)

The

relationship

among

grid

azimuth

(t),

geodetic

azimuth

(a),

convergence

angle

(Y),

and

arc-to-chord

correction

(6)

at

any

given

point

is

t =

o:

- Y +

6.

(Remember

that

6

is

defined

as

t-T).

To

compute 6

requires

knowledge

of

the

coordinates

of

both

ends

of

the

line

to

which

6

is

to

be

applied.

The

following

equations

will

compute 6

12

,

the

6 from

point

(N

1

,

E

1

)

to

(N

2

,

E

2

):

N

m

w

F

E,

w

+ V

0

sin

w

cos

w

1 1

- 6

6N

E

3

F(1

-

27

E

3

2

F).

When

computing

within

the

approximate

boundaries

of

the

SPCS

83

zones,

the

term

11

~

7

E

3

2

F

11

is

negligible

and a

single

value

of

F

can

be

precomputed

for

a mean

latitude.

Often

a

single

value

of

(F/2)

is

combined

with

the

constant

to

convert

radians

to

seconds

(1

radian~

648000/TI

seconds)

yielding

the

expression:

where

the

coordinates

Then

this

equation

is

the

SPCS

27:

are

in

meters.

Sometimes

the

notation

8E

replaces

(E

3

/3).

analogous

to

the

expression

often

used

in

connection

with

-10

2.36

6x

8y

10

seconds

37

where

the

coordinates

are

in

feet.

This

expression

would

serve

for

SPCS

83

coordinates

that

have

been

converted

to

feet

upon

insertion

of

the

negative

sign

to

conform

to

the

sign

convention.

This

expression

with

SPCS

27

coordinates

derived

the

correct

sign

graphically

or

from a

table.

3.26

Grid

Scale

Factor

of

a

Line

As

covered

in

section

4,2,

the

grid

scale

factor

is

different

at

each

end

of

a

line,

but

a

single

value

is

required

to

reduce

a

measured

line.

Given

the

grid

scale

factor

of

endpoints

of

a

line

(k

1

and

k

2

),

a

grid

scale

factor

of

the

line

(k

12

)

is

required.

Below

is

an

alternative

to

the

methods

stated

in

section

4.2.

This

equation

computes

k

12

using

the

function

11

Fn

derived

in

section

3.25

for

the

0

12

correction.

k

1

2

= k

0

[1

+ G(1 +

G/6)].

As

above,

the

term

"G/6

11

is

often

negligible

within

the

bounds

of

the

SPCS

83 and

a

single

value

of

F

will

usually

give

results

within

±(3)(10-

7

)

at

zone

extremes.

3.3

Oblique

Mercator

Mapping

Equations

3,

31

Notation

and

Definition

Asterisked

terms

define

the

projection.

Their

value

can

be

found

in

appendix

A

for

the

one

zone

in

SPCS

83

that

uses

this

projection.

*

•

*

¢

x

Q

x

N

E

N,

E,

k

y

¢c

Xe

"c

k

c

a,

Parallel

of

geodetic

latitude,

positive

north

Meridian

of

geodetic

longitude,

positive

west

Isometric

latitude

Conformal

latitude

Northing

coordinate

Easting

coordinate

False

northing

False

easting

Point

grid

scale

factor

Convergence

Latitude

of

local

origin

Longitude

of

local

origin

Azimuth

of

positive

skew

axis

(u-axis)

at

local

origin

Grid

scale

factor

at

the

local

origin

Azimuth

of

positive

skew

axis

at

equator

A

0

Longitude

of

the

true

origin

ki

2

Line

scale

factor

(between

points

1

and

2)

0

12

Arc-to-chord

correction

(t-T)

(from

point

1

to

point

2)

a

f

Equatorial

radius

of

the

ellipsoid

Flattening

of

the

ellipsoid

3.32

Computation

of

GRS

80

Ellipsoid

Constants

This

section

lists

the

equations

for

the

ellipsoid-specific

constants

and

the

38

constants

derived

for

the

GRS

80

ellipsoid.

The

asterisked

terms

are

required

in

section

3.34

through

3.36.

e

2

=

2f

- f

2

c,

e

2

/2

+

5e

..

/24

+ e

6

/12

+ 13e

8

/360

c,

7e

"I

48

+ 29e

6

/240

+

811e

8

/11520

c,

7e

6

/120

+

81e

8

/1120

c,

4279e

8

/161280

•

F,

2(c

2

- 2c,.

+ 3c6

- 4c

8

)

0.00668

69209

27

(GRS

80)

•

F,

8(c~

- 4c

6

+

1

Oc

s)

0.00005

20145

84

(GRS

80)

•

F,

32(c

6

-

6c

8

)

0.00000

05544 30

(GRS

80)

•

F,

1

28

c,

0.00000

00068 20

(GRS

80)

3.33

Computation

of

Zone

Constants

In

this

section

the

zone

defining

constants,

ellipsoid

constants,

and

expressions

within

the

oblique

Mercator

mapping

equations

are

combined

to

form

several

intermediate

computing

constants

that

are

zone

and

ellipsoid

specific.

These

intermediate

constants,

flagged

with

an

asterisk,

will

be

required

within

the

working

equations

of

section

3.34

through

3.36.

All

angles

are

in

radian

measure,

where

1

radian

equals

180/n

degrees.

Linear

units

are

in

meters.

•

A=

aB(l

- e

2

)

112

/W

2

c

Q

,,,

(l/

2

)~n

1

+sin

q,

0

c

-,~_~~~-

Sin

¢'

C

c

-1

co

sh

- e

in

Note:

-1

cash

x

1/2

in[x+(x

2

-1)

]

39

+ e

sin

- e

sin

•

D

k

A/B

c

sin

"•

=

(a

sin

0:

cos

<I>

)/(AW

)

c c c

For

zone

5001

'

tan

"c

-0.75

sin

"

-0.6

c

cos

"c

+0.8

>,

A

+

{sin

-1

[sin

a:

0

sinh(BQ

C)

I

cos

i:xo]}/B

+

c

•

Note:

sinh

x:

x

-x

(e

-e

)/2

(e

base

of

natural

logarithms)

F =

sin

o.

0

•

G

* I = k

A/a

c

For Alaska zone 1.

these

constants

are:

B 1.00029 64614

04

c 0.00442 68339

26

D 6 386 186.73253

F =-0.32701 29554 38

G 0.94501 98553

34

I 1.00155 89176

62

A

0

101.51383 9560

degrees

3.34

Direct

Conversion

Computation

This

computation

starts

with

the

geodetic

coordinates

of

a

point

(¢,A),

and

computes

the

oblique

Mercator

grid

coordinates

(N,E),

convergence

angle

(Y),

and

the

grid

scale

factor

(k).

Q

c 1

/2)

En

+

sin

¢

~-="--"'

- e

£n

-

sin

$

J

sinh(BQ

+ C)

K cosh(BQ +

C)

+ e

sin

~

-esiniJ

Note:

cosh

x =

(ex

-x

+ e

)/2

(e

=

base

of

natural

logarithms)

-1

u = D

tan

[(JG

- F

sin

L)/cos

L]

D K -

FJ

- G

sin

L

v = 2

~n

K +

FJ

+ G

sin

L

E u

sin

ac

+ v

cos

a

0

+ E

0

For

zone

5001'

N

0.8

u

+

o.6

v

-

5,000,000.

E

.

-0.6

u

+

0.8

v

+

5,000,000.

-1 F

-

JG

sin

L

y

tan

-

"c

KG

cos

L

k =

1(1

- e

2

sin

2

¢)

112

cos{u/D)

cos

¢

cos

L

3.35

Inverse

Conversion

Computation

This

computation

starts

with

the

oblique

Mercator

grid

coordinates

(N,E)

and

computes

the

geodetic

coordinates

(¢,A).

To

compute

the

convergence

angle

(Y)

and

the

grid

scale

factor

(k),

the

computed

(¢,A)

is

then

used

in

the

equations

of

the

direct

conversion

computation.

u •

IE

For

zone

5001 :

u

=

-0.6E

+

0.8N

+

7,000,000.

v =

0.8E

+ 0.6N -

1,000,000.

R

sinh(v/D)

S

cosh(v/D)

Note:

cash

x

T

=

sin(u/D)

Q

E1/2)9.n

x -x

(e

+ e

)12

S -

RF

+

GT

S +

RF

-

GT

41

x

2

tan-1

exp(Q)

-

exp(Q)

+ 1

where

exp(Q)

~

eQ

and

e

~

2,718281828

•••

(base

of

natural

logarithms)

1

RG

+ TF

tan

B

cos(u/D)

3,36

Arc-to-chord

Correction

(t-T)

and

Grid

Scale

Factor

of

a

Line

Having

first

obtained

coordinates

(u

1

,v

1

)

and

(u

2

,v

2

) from

either

the

direct

or

inverse

conversion

computation,

the

(t-T)

correction

for

the

line

from

point

1

to

point

2 (6

12

)

and

line

correction

k

12

may

be

computed.

Q

k,,

+

sin

rp

-

sin

rp

- e

in

+ e

sin

e

sin

cos

rp

cash

(SQ

+

C)

3.4

Polynomial

Coefficients

for

the

Lambert

projection

Conversion

of

coordinates

from

NAD

83

geodetic

positions

to

SPCS

83

plane

coordinate

positions,

and

vice

versa,

can

be

greatly

simplified

for

the

Lambert

projection

using

precomputed

zone

constants

obtained

by

polynomial

curve

fitting.

NGS

developed

the

Lambert

npolynomial

coefficient

1

'

approach

as

an

alternative

to

the

rigorous

mapping

equations

given

section

3.1.

For

many

zones

the

solution

of

the

textbook

mapping

equations

for

the

Lambert

projection

requires

the

use

of

more

than

10

significant

digits

to

obtain

millimeter

accuracy,

and

in

light

of

the

programmable

calculators

generally

in

use

by

surveyors/engineers,

an

alternative

approach

was

warranted.

The

mapping

equations

of

the

transverse

Mercator

projection

do

not

present

the

same

numerical

problem

as

does

the

Lambert

projection.

Therefore,

10

significant

digits

are

adequate.

For

the

polynomial

coefficient

method

of

the

Lambert

projection,

10

significant

digits

will

produce

millimeter

accuracy

in

all

zones.

Given

the

precomputed

polynomial

coefficients,

the

conversion

process

by

this

method

reduces

to

the

solution

of

simple

algebraic

equations,

requiring

no

exponential

or

logarithmic

functions.

It

is

therefore

very

efficient

for

hand

calculators

and

small

computers.

In

addition,

the

conversion

is

not

too

difficult

to

apply

manually

without

the

aid

of

programming.

For

this

reason,

the

polynomial

coefficient

approach

has

also

been

listed

as

a manual

approach

in

table

3.0.

When

programmed,

this

approach

may

be more

efficient

than

the

mapping

equations

of

section

3,1.

42

Rb

R'

Northing Axis

Ao

\

Y\

\

Ro

\

lNo'--.--\\

LN

+·

R

N'=N-No

E'=E-e;,

R'=

R

0

-N'=Rb-N+Nb

+

+•

~-

Nb.l--==::::::::::::__j__----::---:---:c-:-

1 1 Easting Axis

Eo

E

Figure

3.4.--The

Lambert

grid.

The

equations

in

this

section

are

similar

to

those

in

section

3.1,

with

the

symbols

representing

the

same

quantities.

Four

new

symbols

are

introduced,

three

of

which

are

for

polynomial

coefficients--L's,

G's,

and

F's--and

the

fourth

is

the

symbol

11

u".

From

the

equations

and

figure

3.l!,

it

will

be

discovered

that

"u"

is

a

distance

on

the

mapping

radius

"R" between

the

central

parallel

and a

given

point.

The

"L"

coefficients

(L

1

,

L

2

,

L

3

,

etc.)

are

used

in

the

forward

conversion

process

(sec.

3.41),

the

"G"

coefficients

(G

1

,

G

2

,

G

3

,

etc.)

are

used

in

the

inverse

conversion

process

(sec.

3.1!2),

and

the

"F"

coefficients

are

used

in

the

computation

of

grid

scale

factor.

For

the

computation

of

(t-T),

the

methods

in

section

3.15

are

applicable.

The

fundamental

polynomial

equations

of

this

method

are

The

determination

of

"u"

in

meters

on a

plane

by a

polynomial,

given

point

(¢,A)

in

the

forward

conversion,

and

the

determination

by

a

polynomial

of

6¢

in

radians

on

the

ellipsoid

given

point

(N,E)

in

the

inverse

conversion,

is

the

unique

aspect

of

this

method.

The

L-coefficients

perform

the

functions:

(1)

computing

the

length

of

the

meridian

arc

between

¢and

¢

0

,

and

(2)

converting

that

length

to

(R

0

-R) which

is

its

equivalent

on

the

mapping

radius.

The

G-

coefficients

serve

the

same two

stage

process,

but

in

reverse.

The

polynomial

coefficients

of

these

equations,

L's

and

G's,

were

separately

determined

by

a

least

squares

curve

fitting

program

that

also

provided

information

as

to

the

43

accuracy

of

the

fit.

Ten

data

points

were

used

for

each

Lambert

zone

and

the

model

solved

for

the

fewest

number

of

coefficients

possible

that

provided

0.5

mm

coordinate

accuracy

in

the

conversion.

Consequently,

some

small

zones

required

only

three

coefficients,

three

L's

and

three

G

1

s,

whereas

a few

large

zones

required

five

coefficients

for

each

the

forward

and

inverse

conversion.

Appendix

C

discusses

the

computed

constants

and

coefficients

required

for

this

method,

which

are

defined

as

follows:

The

Defining

Constants

of

a Zone:

¢s

or

B

s

Southern

standard

parallel

¢n

or

B

n

Northern

standard

parallel

.b

or

Bb

Latitude

of

grid

origin

,,

or

L,

Central

meridian

-

longitude

of

true

and

grid

origin

Nb

Northing

value

at

grid

origin

(Bb)

E,

Easting

value

at

grid

and

projection

origin

(L,)

The

Derived

Constants:

¢,

or

B,

N,

k,

R,

Rb

M,

The

Polynomial

L,

through

L,

G,

through

G,

F,

through

F,

Central

parallel

-

Latitude

of

the

projection

origin

Northing

value

at

projection

origin

(8

0

)

Grid

scale

factor

at

the

central

parallel

Mapping

radius

at

(8

0

)

Mapping

radius

at

(Bb)

Scaled

radius

of

curvature

in

the

meridian

at

8

0

used

in

section

3.15.

Coefficients:

used

in

the

forward

conversion

used

in

the

inverse

conversion

used

in

the

grid

scale-factor

computation.

3.41

Direct

Conversion

Computation

The

computation

starts

with

the

geodetic

position

of

a

point

($,A),

and

computes

the

Lambert

grid

coordinates

(N,E),

convergence

angle

(Y),

and

grid

scale

factor

(k).

6$ = ¢ - 8

0

(6¢

in

decimal

degrees)

Note:

The

only

required

terms

are

those

for

which

polynomial

coefficients

are

provided

in

appendix

C.

Either

three,

four,

or

five

L's

are

required

depending

on

the

size

of

the

zone.

Suggestion:

Use

nested

form.

44

R

R,

-

u

y

(L,

-

u

sin(B

0

)

E'

=

R

sinY

N'

=

u

+

E'

tan

(Y/2)

E

E'

+

E,

N

N'

+

N,

k

F,

+

F

2

u

2

+

F

3

U

3

3.42

Inverse

Conversion

Computation

convergence

angle

easting

northing

grid

scale

factor

This

computation

starts

with

the

Lambert

grid

coordinates

(N,E)

from

which

are

computed

the

geodetic

coordinates

(~,A),

convergence

angle

(Y),

and

grid

scale

factor

(k):

N'

N

-

N,

E'

E -

E,

R'

R,

-

N'

y

tan

(E'/R')

convergence

angle

! L,

Y/sin(B

0

)

longitude

u =

N'

-

E'tan(Y/2)

Note:

The

only

required

terms

are

those

for

which

polynomial

coefficients

are

provided

in

appendix

C.

Either

three,

four,

or

five

G's

are

required

depending

on

the

size

of

the

zone.

•

k

Suggestion:

Use

factored

form.

latitude

grid

scale

factor

45

~.

LINE

CONVERSION

METHODS

REQUIRED

TO

PLACE

A

SURVEY

ON

SPCS

83

State

plane

coordinates

are

derived

from

latitudes

and

longitudes.

Latitudes

and

longitudes

are

based

on

an

ellipsoid

of

reference

and

a

horizontal

datum

that

approximates

the

surface

of

the

Earth.

Accordingly,

field

observations

measured

on

the

ground

must

first

be

reduced

to

the

surface

of

the

horizontal

datum

before

they

are

further

reduced

to

the

map

projection

surface--the

grid.

The

mathematical

process

or

reduc.ing

f.i.eld

observations

does

not

necessarily

imply

that

the

numbers

are

reduced

in

magnitude

although

often

that

is

the

case.

Section

4.1

addresses

the

reduction

of

measured

distances

to

the

datum

surface,

not

a

subject

of

map

projections,

but

included

here

for

convenience.

Only

the

geometric

aspect

of

reduction

is

disCU.$Sed.

Reductions

relating

to

the

influence

of

the

atmosphere

are

not

included.

Section

4.2-contains

the

further

reduction

of

measured

distances

to

the

grid,

expanding

on

section

2. 6

and

applying

the

concept

of

point

grid

scale

factors

to

an

entire

measured

line.

Section

4.3

discusses

the

reduction

of

azimuths

and

angles

from

the

ellipsoid

to

the

grid,

applying

the

concepts

stated

in

sect1on

2.

5.

Reduct1on

of

angles

and

azimuths

to

the

ellipsoid

is

beyond

the

scope

of

this

manual.

The

reader

is

referred

to

texts

on

higher

geodesy.

4.1

Reduction

of

Observed

Distances

to

the

Ellipsoid

Before

a

measured

distance

can

be

reduced

to

a

grid

distance

in

a zone

of

the

SPCS

83,

it

must

first

be

reduced

to

a

geodetic

distance.

Classically,

observed

distances

have been

reduced

to

one

of

two

surfaces,

either

the

geoid

(sea

level)

or

the

ellipsoid.

(See

fig.

4.1a.)

To

which

surface

distances

were

reduced

depended on

available

information.

Generally,

in

conjunction

with

NAD

27,

distances

were

reduced

only

to

sea

level,

although

subsequent

computations

using

those

distances

were

performed

on

the

ellipsoid.

This

incomplete

reduction

was

adequate

for

NAD

27,

as

the

ellipsoid

of

NAD

27

{Clarke

Spheroid

of

1866)

closely

approximated

sea

level,

For

NAD

83,

due

to

availability

of

information

on

geoid-

ellipsoid

separation,

distances

may

be

reduced

to

the

ellipsoid.

Furthermore,

Figure

4.1a.--Geoid-ellipsoid-surface

relationships.

D

------

Center

of

Earth

- Sea-Level (Geoid)

- Ellipsoid

S=D(R+:+H)

Where S =Geodetic Distance

0

==

Horlzontal Distance

H

"'Mean

Elevation

N

=Mean

Geold Height

R

=Mean

Radius

ol

Earth

Figure

4.

lb.--Reduction

to

the

ellipsoid.

the

worldwide

datum

of

NAO

83

does

not

fit

the

North

American

continent

as

well

as

the

27.

The

impact

of

this

on

surveyors

may

be

the

requirement

to

use

this

geoidal

~eparation

information

in

connection

with

the

reduction

of

observed

distances

to

the

ellipsoid.

The

approximation

of

using

sea

level

may

not

always

be

adequate,

but

those

occurrences

should

be

few and

affect

only

surveys

of

highest

order.

To

reduce

measurements

to

the

ellipsoid

instead

of

sea

level

requires

the

addition

of

the

geoid

height

{sea

level/ellipsoid

separation)

to

the

station

elevations

prior

to

reduction.

In

the

conterminous

United

States

the

ellipsoid

is

above

the

geoid.

In

Alaska

the

ellipsoid

is

below

the

geoid.

Since

the

geoid

height

of

a

station

is

defined

as

the

height

abovethe

ellipsoid

minus

the

height

above

the

geoid,

except

in

Alaska

it

is

a

negative

value.

The

geoid

height

is

published

by

NGS

together

with

NAO

83

coordinate

information.

The

geodetic

height

of

a

control

station

{height

above

ellipsoid)

"h"

is

the

swn

of

elevation

above mean

sea

level

"H"

and

geoid

height

"N". The

failure

to

use

geoid

height

will

introduce

an

error

in

reduced

distance

of

0.16

ppm

for

each

meter

of

geoid

height.

A

geoid

height

of

-30

m

systematically

affects

all

reduced

distances

by

-4.8

ppm

(1:208,000).

Clearly

a

single

geoid

height

may

be

applied

for

a

region

or

project,

and

even

ignored

for

many

types

of

surveys.

The

application

of

geoid

height

and

the

precise

determination

of

a

radius

of

curvature

on

the

ellipsoid

{below)

are

the

only

occurrences

where

NAO

83

may

affect

changes

to

distance

reduction

procedures.

Knowledge

of

radii

of

curvature

on

ellipsoids

is

paramount

to

the

reduction

of

distances

measured on

the

surface

of

the

Earth

to

either

sea

level

or

the

47

ellipsoid

surface.

Ellipsoid

radii

are

a

function

of

both

latitude

and

azimuth.

Fortunately,

for

many

uses

any

mean

radius

of

curvature

is

often

a

satisfactory

approximation.

Distance

reductions

are

often

performed

on

a

sphere

having

a

radius

equal

to

the

mean

radius

of

curvature

of

the

ellipsoid

at

an

average

latitude

of

the

conterminous

United

States.

Figure

l!.1b

illustrates

the

quantities

involved

in

the

reduction

assuming

this

sphere.

From

the

proportion:

SID =

R/(R+h),

we

solve:

S .. D*R/(R+h)

h N + H

by

definition.

Therefore,

S = D*R/(R+N+H).

The

ratio

R/(R+N+H)

is

similar

to

the

familiar

sea

level

factor

except

that

the

average

elevation

of

line

"D

11

above

the

ellipsoid,

usually

denoted

as

"h"

and

called

sea

level

in

most

NAD

27

literature,

is

replaced

by

11

H

11

as

the

height

above

sea

level

(the

geoid)

and

"N"

the

ellipsoidal

separation.

To

emphasize

the

difference,

the

ratio

has

been

designated

as

an

elevation

factor.

On

NAD

83,

"h"

remains

the

height

above

the

ellipsoid,

but

is

obtained

by

adding

together

the

geoid-ellipsoid

separation

"N"

and

the

height

of

the

station

above

the

geoid

"H

11

•

In

line

reductions

by

this

method,

a mean

geoid

height

'

1

N

11

and

mean

elevation

"H"

are

used

to

obtain

a mean

height

of

the

line

11

h".

Figure

lt.1c

depicts

the

situation

of

a

negative

N,

as

is

the

case

in

the

conterminous

United

States.

D

s

------

R

------

Center

of

Earth

- Ellipsoid

- Sea-Level

(Geotd)

..§.

_ _JL_

D

-R+h

:.s=o(R:h)

h = N + H by Definition

:.s=o(R+:+H)

Figure

ll.1c.--Reduction

to

the

ellipsoid

{shown

with

a

negative

geoid

height).

48

The mean

radius

"R"

used

in

connection

with

NAD

27 was

20,906,000

ft,

or

6,372,000

m.

This

approximate

radius

serves

equally

well

for

NAD

83.

The

elevation

factor

is

often

combined

with

the

grid

scale

factor

of

a

line

(see

~.2)

to

form a

single

multiplier

that

reduces

an

observed

horizontal

dis-

tance

at

an

average

elevation

directly

to

the

SPCS

grid.

These

two

factors

are

quickly

combined

by

obtaining

the

product

of

the

factors.

This

product

is

approximated

by

subtracting

11

1

11

from

the

sum

of

the

two

factors.

Identified

as

the

"combined

factor,"

when

multiplied

by

the

horizontal

distance

it

has

the

same

effect

as

each

factor

multiplied

separately,

yielding

the

grid

distance.

If

the

area

of

a

parcel

of

land

at

ground

elevation

is

desired,

the

area

obtained

from

using

SPCS

83

coordinates

should

be

divided

by

the

square

of

the

combined

factor

(the

same

factor

that

was

used

to

reduce

the

measured

distances)

to

obtain

the

area

at

ground

elevation.

Although

the

above

approximate

method

serves

most

surveyors

and

engineers

well,

sometimes

a more

rigorous

reduction

procedure

will

be

required.

Such a

procedure

is

found

in

NOAA

Technical

Memorandwn

NOS

NGS-10, Use

of

calibration

base

lines,

appendix

I:

nThe

geometrical

transformation

of

electronically

measured

distances"

(1977).

4.2

Grid

scale

factor

k

12

of

a

Line

As

discussed

in

section

2.6

on

grid

scale

factor,

an

incremental

length

on

the

ellipsoid

must be

multiplied

by

a

grid

scale

factor

to

obtain

the

length

of

that

increment

on

the

grid.

However, measill'ed

survey

lines

are

not

infinitesimal

increments,

and

grid

scale

factor

ratios

change

from

point

to

point.

Therefore,

we

are

faced

with

the

problem

of

deriving

a

single

grid

scale

factor

that

can be

applied

to

an

entire

measured

length

(that

has

first

been

reduced

to

the

ellipsoid),

when

in

fact

the

value

of

the

grid

scale

factor

is

changing

from

point

to

point.

Required

is

a

grid

scale

factor

ratio

that

when

multiplied

by

the

measured

ellipsoid-reduced

distance

will

yield

the

grid

distance.

(See

fig.

4.2.)

This

grid

scale

factor

which

applies

to

a

line

between

points

l

and

2

is

symbolized

as

k

12

•

1------

LIMITS

OF

PROJECTION

------j

-!-----SCALE

TOO

SMALL----!-

SCALE SCALE

TOO TOO

LARGE \ LARGE

'

'•

~v~~

/·',_

\

~o~

I

',

\

1'.':\

I

B'

GAIO PLANE

c·

.......

Grid Dlsttn<:e

C'

10

0'

11

Lllrger Than

Geodellc

Ollttnce

c

10

o

Figure

4.2.--Geodetic

vs.

grid

distances.

49

Recalling

we

are

computing

on a

conformal

projection,

the

grid

scale

factor

is

the

same

in

any

direction,

but

increases

in

magnitude

with

distance

of

the

point

from

the

central

meridian

in

a

transverse

Mercator

projection,

or

central

parallel

in

a

Lambert

projection.

This

means

that

the

grid

scale

factor

is

different

at

each

end

of

a

measured

line,

and

that

this

difference

is

greatest

for

an

east-west

line

in

the

transverse

Mercator

projection,

or

north-south

line

in

the

Lambert

projection.

There

are

several

solutions

to

the

problem

of

deriving

a

grid

scale

factor

for

a

line.

The

solution

depends

on

the

required

accuracy

of

the

reduction,

the

lengths

of

lines

involved,

and,

to

a

lesser

degree,

the

areal

extent

of

the

zone

and

location

of

the

line

within

the

zone.

The

application

of

the

grid

scale

factor

to

measured

lengths

for

each

project

should

begin

with

an

analysis

of

the

magnitude

of

the

correction

within

that

project--a

function

of

the

average

length

of

measured

lines

and

location

of

the

project

within

the

zone--compared

with

the

desired

project

accuracy.

For

surveys

of

third-order

accuracy

or

less

(as

classified

by

the

Federal

Geodetic

Control

Committee)

a

single

scale

factor

for

all

lines

in

the

project

may

suffice.

This

grid

scale

factor

would

be computed

at

the

center

of

the

project.

It

may

be

determined

that

in

the

reduction

of

measured

geodetic

lengths

to

the

grid,

the

grid

scale

factor

could

be

ignored.

To

determine

an

appropriate

method

for

computing

the

line

grid

scale

factor

for

any

project,

it

is

suggested

that

one

first

determine

point

grid

scale

factors

for

the

worst

case

situation

in

the

project--ends

of

the

longest

line

that

run

in

a

direction

perpendicular

to

the

central

axis

of

the

projection,

and

at

the

greatest

distance

from

the

central

axis.

The

central

axis

is

the

central

meridian

in

the

transverse

Mercator

or

central

parallel

in

the

Lambert

projection.

The

appropriateness

of

approximations

for

each

line

or

for

a

project,

i.e.,

a

single

project

grid

scale

factor,

is

dependent

on

the

computing

error

that

can

be

tolerated.

When

a

single

grid

scale

factor

for

a

project

is

acceptable,

it

may

also

be an

acceptable

approximation

to

use

a

single

elevation

reduction

factor,

a

similar

looking

multiplier

that

reduces

a

measured

horizontal

line

on

the

Earth's

surface

to

its

equivalent

ellipsoid

length.

(See

sec.

4.1.)

Sometimes

a combined

project

factor

is

used

to

reduce

all

measured

horizontal

distances

from

the

average

elevation

of

the

project

directly

to

the

grid.

The

appropriateness

of

a

single

project

elevation

reduction

factor

requires

the

similar

analysis

as

a

project

grid

scale

factor.

When

a

line

grid

scale

factor

must

be

determined

for

each

measured

line

of

the

survey,

there

are

several

approaches

for

handling

the

fact

that

point

grid

scale

factors

are

different

at

each

end

of

a

line.

Each

approach

requires

computing

one

or

more

point

grid

scale

factors.

Approximate

equal

results

are

obtained

from

either

using

the

point

scale

factor

of

the

midpoint

of

the

line

or

a mean

scale

factor

computed from

the

point

scale

factor

for

each

end

of

the

line.

The

most

accurate

determination

of

the

line

grid

scale

factor

(k

1

~)

requires

computing

point

scale

factors

at

each

end

of

the

line

(k

1

and

kz)

plus

the

midpoint

(km) and

combining

according

to:

50

lJ.3

Arc-to-Chord

Correction

(t-T)

As

given

in

section

2.5,

the

representation

(projection)

of

a

geodetic

azimuth

"a:" on

any

plane

grid

does

not

produce

the

grid

azimuth

11

t"

but

the

projected

geodetic

azimuth

11

T

11

•

Figure

2.5

illustrates

the

small

difference,

11

t-T

11

•

11

t-Tn

(alias

"arc-to-chorctn

and

"second

difference")

is

an

angular

correction

to

the

line

of

sight

between

two

points,

whether

that

11

direction

11

is

an

azimuth

or

part

of

an

angle

measure.

The

11

t-T"

is

the

difference

between

the

"pointing

11

observed

on

the

ellipsoid

(generally

the

same

as

on

the

ground)

and

the

pointing

on

the

grid.

The

difference

is

often

insignificant.

From a

purely

theoretical

perspective,

grid

azimuths

should

be

used

with

grid

angles

and

directions,

while

geo9etic

azimuths

should

be

used

with

observed

angles

and

directions.

To

perform

survey

computations

on

a

plane,

observed

directions

should

be

corrected

for

the

arc-to-chord

(t-T)

correction

to

derive

an

equivalent

value

for

the

observed

direction

on

the

grid;

otherwise

observed

angles

should

be

used

with

geodetic

azimuths

with

survey

computations

performed

on

the

ellipsoid.

For

example,

in

a

traverse

computation

on

the

ellipsoid,

the

azimuth

would

need

to

be

carried

forward

by

geodetic

methods

where

forward

and

backward

azimuths

differ

by

approximately

6A"sin

¢ ±

180°.

m

From a

practical

perspective

in

many

survey

operations

the

(t-T)

correction

is

negligible,

observed

angles

are

used

with

grid

azimuths,

and

survey

computations

are

done

on

a

plane.

In

a

precise

survey

it

is

necessary

to

evaluate

the

magnitude

of

(t-T).

Table

~.3a

provides

an

approximation.

Table

4.3a.--Approximate

size

of

(t-T)

in

seconds

of

arc

for

Lambert

or

transverse

Mercator

projection

(see

note

1)

l>E

or

6N

(See

note

2)

(km)

2

5

1 0

20

Perpendicular

to

midpoint

50

0.3

o.6

1.

3

2.5

distance

from

central

axis

of

the

line

(see

note

3)

(km)

1 00

150

200

250

0,5 0.8

1.

0

1.3

1.

3

1.

9

2.5

3.2

2.5

3,8

5.

1

6.4

5.

1

7,6

1

0.

2 1 2, 7

(

1)

(t-T)

is

also

a

function

of

latitude,

but

often

is

(t-T)

25. 4

-10

estimated

by

=

(bN)(bE)10

seconds,

where

6N

and

'E

are

in

meters.

( 2)

The

length

of

the

line

to

which

the

correction

is

to

be

applied

is

in

a

direction

parallel

to

central

axis.

(3)

A

better

approximation

is

obtained

by

taking

the

distance

from

the

central

axis

to

a

point

one-third

of

the

distance

from

point

1

to

point

2 when

estimating

(t-T)

at

point

1.

51

*

Note

on

the

use

of

Table

4.3a

IN

A

STRAIGHT

TRAVERSE

OF

EQUAL

LINE

LENGTHS,

THE

CORRECTION

TO

AN

ANGLE

WILL

BE

DOUBLE

THE

ABOVE

•

*

CORRECTION

TO

EACH

DIRECTION,

AND

THESE

ANGULAR

*

CORRECTIONS

SYSTEMATICALLY

ACCUMULATE

ALONG

THE

*

TRAVERSE.

BECAUSE

OF

THE

DOUBLING

ACTION,

IF *

*

THIS

TABLE

IS

USED

TO

ESTIMATE

THE

PORTION

OF

AZIMUTH

*

MISCLOSURE

OF

AN

ENTIRE

TRAVERSE

SURVEY

ATTRIBUTED

*

•

*

TO

IGNORING

THIS

CORRECTION,

THE

CONTRIBUTION

WOULD

BE

TWICE

THE

TABLE

VALUE.

•

Use

of

the

table

requires

knowledge

of

two

items.

First,

the

approximate

perpendicular

distance

from

the

central

axis

(central

parallel

in

Lambert

projection

or

the

central

meridian

in

Mercator

projection)

to

the

midpoint

of

your

line

is

required.

The

midpoint

is

derived

from

differencing

mean

coordinates

of

the

line

(northings

for

Lambert and

eastings

for

Mercator)

from

the

northing

of

the

central

parallel

or

the

easting

of

the

central

meridian.

Also

needed

to

use

table

4.3a

is

the

length

of

the

line

in

a

direction

parallel

to

the

central

parallel

(Lambert)

or

parallel

to

the

central

meridian

(Mercator).

Again

~E

or

~N

is

derived

from

the

point

coordinates.

From

studying

table

4.3a

it

is

apparent

that

the

(t-T)

correction

will

be

its

largest

on

lines

parallel,

and

the

greatest

distance,

from

the

central

axis

of

the

projection

zone.

Table

4.3b.--Sign

of

(t-T)

correction

Map

projection

Lambert:

Transverse

Mercator:

Sign

of

N-N

0

Positive

Negative

Sign

of

E-£

0

(or

E

3

)

Positive

Negative

Azimuth

of

the

line

from

north

O

to

180°

180

to

360°

+

270-90'

90-270°

+

Figure

4.3

illustrates

the

relative

orientation

of

projected

geodetic

lines

(T)

and

grid

lines

(t)

for

traverses

located

on

either

side

of

the

central

axis.

It

should

be

observed

that

the

projected

geodetic

line

is

always

concave

towards

the

central

meridian

or

parallel.

This

fact

provides

a

visual

check

on

the

correct

sign

of

the

(t-T)

correction.

For a

conventional

nearly

straight

traverse,

the

signs

of

the

(t-T)

correction

on

each

direction

of

an

observed

angle

are

opposite,

thus

the

corrections

accumulate.

Therefore,

for

a

straight

traverse

of

52

'

,

'

,

'

,

Transverse Mercator Projection

'

,

-------------

Central Parallel

~~-~~~ ~~-

---

Lambert Projection

Figure

11.3.--Projected

geodetic

vs.

grid

angles.

approximately

equal

line

lengths,

the

(t-T)

correction

for

the

observed

angle

Will

be

twice

the

computed

correction

to

a

single

direction.

Although

the

formulas

in

chapter

3

will

provide

the

proper

sign

of

(t-T),

table

4.3b

may

also

be

used

as

a

guide.

4. 4

Traverse

Example

This

section

ill

us

tr

ates

the

solution

to

a

traverse

computation.

Given

the

terrestrial

survey

observations

for

a

closed

connecting

traverse

between

points

of

known

position,

final

adjusted

coordinates

are

c001puted

for

new

points.

The

emphasis

in

the

example

is

the

procedure

to

reduce

angles

and

dlstances

measured

on

the

surface

of

the

Earth

to

an

equivalent

value

on

the

grid

of

the

SPCS

83.

These

reduction

procedures

are

applicable

regardless

of

the

positioning

method

or

network

geometry.

After

the

field

data

are

reduced

to

the

SPCS

83

grid,

the

data

are

made

consistent

with

the

SPCS

83

plane

coordinates

of

the

fixed

control

station

using

the

method

of

the

compass

rule

adjustment.

Mathematical

rigor

plus

data

encoding

and

programming

consideration

often

make

adjustment

by

least

squares

the

preferred

method,

but

the

compass

rule

adjustment

is

Widely

used

and

serves

well

for

this

example

where

computations

on a

plane

are

being

illustrated.

Furthermore,

the

example

in

this

section

was a

sample

NAD

27 problem

used

at

more

than

30

workshops

instructed

by

NGS,

so

adj

us

ting

identical

field

data

to

NAO

83

control

illustrates

datum

differences.

53

Figure

4.4a

is

a

sketch

of

the

sample

traverse.

For

instructional

consideration,

it

purposely

violates

Federal

Geodetic

Control

Cc:mmittee

specifications

for

network

design.

Four

new

points

are

to

be

positioned

between

two

points

of

known

position.

The

starting

azimuth

(azimuth

at

station

number 1)

is

derived

from

published

angles

while

the

closing

azimuth

(azimuth

at

station

number 6)

is

derived

from

published

coordinates.

The

solid

lines

depict

grid

ines;

dotted

lines

show

projected

geodetic

lines.

The

difference

is

(t-T).

The

illustrated

northing

of

the

central

axis

together

with

the

approximate

point

coordinates

are

used

in

the

computation

of

(t-T).

The

computation

of

(t-T)

requires

the

distance

of

the

traverse

line

from

the

central

axis.

Similarly,

a

traverse

line

in

a

transverse

Mercator

zone

requires

E

0

,

the

easting

of

the

central

axis.

The

following

steps

are

required

to

compute any

traverse:

1.

Obtain

starting

and

closing

azimuth.

2. Analyze

the

grid

scale

factor

for

the

project.

A

mean

of

the

published

point

grid

scale

factors

of

the

control

points

may

be

adequate

for

all

lines

in

the

project,

or

a

grid

scale

factor

for

each

line

may

be

required.

3.

Analyze

the

elevation

factor

for

the

project.

A mean

of

the

published

elevations

of

the

control

points

corrected

for

the

geoid

height

(N)

may

be

adequate

to

compute

the

elevation

factor.

Otherwise

each

line

may

need

to

be

reduced

individually.

4.

If

a

project

grid

scale

factor

and

project

elevation

factor

are

applicable,

compute a

project

cooibined

factor.

No----

Church Spire

9

I

Central Axis

----

No'""

155,664.30

1

@-~_..,_,,,

__

:::_:=

__

::-,_,,,_>-9

2

@ Fixed Control Point

o New

Points

Point

1

2

3

4

5

6

'

3

Approximate Coordinates

.!:!

!.

61,400 meters

61,300

57,300

58,200

61,800

58,900

660,300 meters

665,100

665,400

670,300

670,500

674,000

5

6

~

Azimuth Mark

Figure

4.4a.--Sample

traverse.

(N

specifies

northing

component

and

E

specifies

easting

ccxnponent.)

5.

Reduce

the

horizontal

distances

to

the

grid.

6.

Using

preliminary

azimuths

derived

from

unreduced

angles

and

grid

distances,

compute

approximate

coordinates.

7. Analyze

magnitude

of

(t-T)

corrections,

and

if

their

application

is

required,

compute

the

(t-T)

corrections

for

each

line

using

approximate

coordinates

for

each

point,

8.

Apply

(t-T)

corrections

to

the

measured

angles

to

obtain

grid

angles.

9.

Adjust

the

traverse.

10. Compute

the

final

adjusted

1983

State

Plane

Coordinates

for

the

new

points,

adjusted

azimuths

and

distances

between

the

points,

and

if

required

ground

level

distances.

Step

To

obtain

the

starting

azimuth,

use

the

published

azimuth

and

angle

information

(Fig.

4.4b)

and compute

the

grid

azimuth

from

point

1

to

the

church

spire.

Plane

azimuth

Plus

observed

point

1

to

azimuth

mark

clockwise

angle

from

azimuth

Starting

azimuth

30°30'12.6''

mark

to

church

spire

329 50

18.6

0 °20

1

31.2

11

Recalling

that

plane

angles

are

used

with

plane

azimuths

and

observed

spherical

angles

are

used

with

geodetic

azimuths,

in

theory

the

above

observed

angle

should

have

been

corrected

for

the

(t-T)

correction.

However,

because

each

direction

of

the

angle

was

short,

the

(t-T)

correction

is

zero.

To

obtain

the

closing

azimuth,

use

the

published

coordinate

information

in

figure

4.4b

and

compute

the

grid

azimuth

from

Point

6

to

Point

6 Azimuth Mark

using·

a

plane

coordinate

inverse:

tan

azimuth

1

:z

azimuth

12

~arc

tan

(121.457/~85.047)

Steps

2

through

4

Grid

scale

factor

point

1

Grid

scale

factor

point

6

Mean

grid

scale

factor

1 • 0000420

1.

0000480

1.

0000450

Elevation

point

1

Elevation

point

6

Mean

H

Mean

N

Elevation

factor

830.0

ft

900.0

ft

865.0

ft

-30.5

m =

-100

ft

(20,906,000)/(20,906,000

+ H + N)

(20,906,000)/(20,906,765)

0.

9999634

55

194°03'28.5

11

NAMEOF$TATION;

Point

1

STATE:

Wisconsin

SOURCE:

G-17289

NORTH

AMERICAN

DATUM

1983

ADJUSTED

HORIZONTAL

CONTROL

DATA

1980

Second

-oAoE"

Geoid

Height

-30.5

meters

GEODETIC

LATITUDf::

GEODETIC

LONGITUDE:

STATE

&

ZONE

42

33

00.01150

89

15

56.24590

STATE

COOROINATES

CODE

Northing

830.S

FEET

Easting

Mapping

angle

•

"

Wisconsin

4803

61,367.006

660,318.626

+ 0

30

16.

5

Scale

Factor~

1.0000420

.

GEODETIC

A:!:IMUTN

PL,.NE

Al:U.OUYH

TO

STATION

OR

0....,CT

coot

. . .

.

Point

1

Azimuth

Mark

31

00

29.l

30

12.6

4803

DESCRIPTION

OF

TRAVERSE

STATION

NAME

OF

STATION:

Point

1

STATE:

Wisconsin

COUNTY:

LEO

CHIEF

OF

PARTY:

E.

J.

McKay

VEAR:

19BQ

OESCRIBEO

BY:

JES

.

OfOTE.

'"'EIGHT

OF

TELESCOPE

A•OvE

STllTION

MARK

METERS,t

NEIGHT

OF

LIGHT

ABOVE

STATIOf'I

MAllllC

•ETERS.

SURFACE-STATION'""'""·

I

DISTANCES

AHO

OIRECTIOt4S

TO

AZlfllUTN

MARii(,

REFEttEMCE

lllAIUCS AHO

f'AOl'IHEHT

UMOEfltGflt()UHO-STATIOM MARI(

09J£CTS

WHtCHCAH

9E

SEEM

FR°"'

THE

GttOUMO

AT

THE

STATIOtl

08JECT

8EAfltlMG

CMSTAHCE

OHIECT10HI

IF"Eo;..-

...

..,..-o;

..

5

Point

6 E

13.886.

79

.

0 00

oo.a·

RMl

ESE

30.

28"

33

46

2B.l

RM2

WSW

22.98•

123 33

29.2

Church

Spire

(unidentified)

N

(2.3

mile< I

260

19

01.S

Azimuth

Mark

NNE

Co.2 mile< I

290

28

42.9

Figure

4.4b.--Fixed

station

control

information.

56

,.,...,e

o~

sTAT10

.. ,

Point

6

STATE

Wisconsin

SOURCE

G-17289

G£00ET1C

LATITUDE

GEODETIC

LO.,GITUOE.

STATE

&

ZONE

Wisconsin,

s

NORTH

AMERICAN

DATUM

1983

ADJUSTED

HORIZONTAL

CONTROL

DATA

YEAA

1980

Second

-o

..

oEP.

Geoid

Height

-30.5

meters

42 31

89

05

COOE

4803

37.32888

58.04271

1983

STA

TE

COOAOINATES

Northing

58,949.532

(meters)

Easting

673,994.015

9QQ

• Q

~EfT

Mapping

Angle

•

"

+

0

37

07.5

Sc.ale

Factor

1.0000480

ADJUSTED HORIZONTAL CONTROL

DATA

Point

6

Azimuth

Mark

STATE

Wisconsin

$0UAC£

G-14402

GEODETIC

LATITUOE'

OE0r;>ETIC

LONGIT"UOE:

STATE

&

ZONE

Wisconsin,

s

42

31

89

06

CODE

4803

VEAR

1980

21.65360

03.59289

ST

...

TE

C00fl01NATES

Northing

58,464.485

Second

.o

.. oe"

750.0~EET

Easting

Mapning

angle

0

673,872.558

+

0

37

03.

7

Scale

Factor

1.0000491

Figure

4.4b.--Fixed

station

control

information

(continued).

57

Combined

factor

Step

5

Step

6

From

1

2

3

4

5

(1.0000450)(0.9999634)

1.

0000084

TO

2

3

4

5

6

Measured

hori_

zontal

lengths

4,805.468

3.963.694

4,966.083

3,501 .223

4,466.935

Grid

lengths

4,805.508

3,963.

727

4,966.125

3,501.252

4,466.973

The

computation

of

preliminary

coordinates

is

not

illustrated.

The

procedure

used

to

obtain

preliminary

coordinates

for

the

adjustment

is

generally

used.

Step

7

Figure

4,4c

illustrates

computation

of

(t-T)

using

the

abbreviated

formula

(t-

-10

T)

=

(25.4)(6N)(LiE)(10

)

seconds.

The

(t;N)

is

the

distance

of

the

midpoint

of

the

line

from

the

central

axis.

For

example:

No----

Central Axis

Church Spire

~

~-:.,·-==--==-

=~2

'

5

6

@ Fixed Control Point

o New Points

''L,=

__

:::;:_.:::::--~--~---

4

Azimuth Mark

3

From !2

AN

1 2 - 0.943

2 3 - 0.964

3 4 - 0.979

4 5

-0.957

5 6

-0.953

AE

0.048

0.003

0.049

0.002

0.035

25.4

CAN)

!AE)

-1

~1

-

0.1

-1.2

-0.1

-0.8

Figure

4.4c.--(t-T)

correction

(6

northing

and

6

easti.ng

expressed

in

meters).

58

~N.,

=

[(61,400

+

61,300)/2

-

155,664]10-

5

•

-0.943

(!IE)

is

the

difference

of

eastings

of

the

endpoints

of

the

line.

For example:

o.

048

-5

-10

Note

that

(!IN)

and

(6E)

are

each

scaled

by

(10

)

to

account

for

the

(10

)

in

the

equation

for

(t-T).

Step

8

The

corrections

computed

in

step

7

are

applied

to

an

observed

pointing

in

one

direction.

Using

this

approximate

equation

for

(t-T),

the

correction

from

the

other

end

of

the

line

is

identical

but

with

opposite

sign.

Figure

ll.4d

lists

the

observed

traverse

angles,

(t-T)

corrections

to

each

direct.ton,

angle

correction,

and

the

grid

angle.

Backsight

Foresight

Angle

Point

Observed

Ans

le

Correction

Grid

Angle

.

90

44

18.3

0

-1.1

-1.

1

17.2

2

265

15

SS.2

+l.l

-0.1

-1.2

54.0

3

82

48

26.9

+0.1

-1.2

-1.3

25.6

4

105

03

08.6

+1.2

-0.1

-1.3

07.3

5

304

33

46.2

+0.1

-o.a

-0.9

45.3

6

245

17

39.5

+0.8

0 -o.a

36.7

$TATIOH

PFt~t.U""AAY

AUMUTt<

CO~R!:CT•o~

Fo~

CORRECTED

A?O

..

l!Tt<

Ct.O•UR~

FflO"'

"

.

"

.

"

I

Azimuth

Mk.

00

20

31.2* 00

20

31.2*

l

90

44

17

.2

-1.B

90

44

15.4

I

2

91

04

48.4

I

91

04

46.6

2 1 271

04

48.4

271 04

46.6

l

265 15

54.0

-l

.8

265 15 52.2

2

3 176

20

42.4

176

20

38.8

3

2 356

20

42.4

356

20

38.8

l

82

...

25.6

-1.8

82

48

23.8

'

4

79

M

08.0

79

09

02.6

4

I

3 259 09

08.0 259 09

02.6

L

i

105

03

07

.3

-1.8

105

03

05.5

4

I

5

4

12

15.3

4 12

08.

l

'

I

4 184

12

15.3

184

12

08. l

L

I

304

33

45.3

-1.8

304

33

43.5

'

5

i

6 128

46

00.6

128

45

51.6

6

'

5

308

46

00.6 308 45

51.6

l

I

245

17

38. 7

-1.8

245 17

36.9

6

Azimuth

Mark

194 03

39.3

194

03

28.5*

Closure =

-10.8

L

*Fixed

Grid

Az

·muths

Figure

4.

4d.--Azimuth

adjustment.

59

n•TICH

'

2

-

•

,

I

6

!:!!Q!!

!2

l 2

2

J

•

5

6

Step

9

tlM

Ail

..

UTH

R~AM!

A%1WU•H <IRID

DllTAHC~

COi

AIUMITH

~ATITUDl

.i-o.

99982248

"

46.6

41105

508

-0.01884165

-90.544

rn

20 38.B 3963. 727

- -

+0.98212573

.,nn",..n•r

+0.1BB22607 .+934. 754

" "

•

12

08.l

3501.252

+0.9973116()

+3491.839

+O. 77972788

""

.,

- .

--

-2796.855

Dl~A•TU•t

Fix.a

+4804.655

+4877.359

•

+3483.0'>3

GRID

COO•O•MATU

61,367.006

660,318.626

<>

"

-

0.223

...

0.232

61.276.239

665

123.513

..

665

376.024

-

"'7

32-

·-4

665 376 447

.

670

253.

-

0.637

...

0.662

58

254.918

670

254.045

61,747.394

670,509.946

- " '

...

0

831

61,746.595

670,510.777

-

1.007

...

1.046

58

949.532

fl)

Prel.lllWlllrY

coord.lnate

12)

Aocl.mll11tive

Q:>rrection

(31

Adjusted

Coordin<lte

..

"

13

I

Figure

4.4e.--Traverse

computation

by

latitudes

and

departures.

FINAL

ADJUSTED RESULTS

LATI'l'tJDE

DEPARTURE

GRID

LENGTH

TAN

(OR COT")

GROUND

'LENGTH

AZIMUTH

90.

767

+4804.887

4805.744

-0.01889056"

4805.704

91

"

56.0

-3955.845

+

252.934

3963.923

-0.06393931

3963.890

176

20

29.

5

+

934.524

+4877.598

4966.316

+0.19159513"

4966.274

"

09

13.

9

+3491.677

+

256.732

3501.103

+0.07352685

3501.074

12

1e.

e

-2797.063

+3483.238

4467.271

-o.B0300657"

4467.

233

128

"

52.

9

Figure

4.4f.--Adjusted

traverse

data.

Using

the

starting

grid

azimuth

and

grid

angles,

the

computed.

(See

fig.

4.4d.)

The

misclosure

of

(-10.8)

the

grid

angles

and

final

corrected

azimuths

computed.

closing

azimuth

is

seconds

is

µ"Orated

among

The

adjusted

azimuths

and

60

grid

distances

are

transferred

to

figure

4.

4e

where

the

coordinate

misclosures

are

determined

and

misclo.sures

prorated

according

to

the

compass

rule

adjustment

method.

Step

10

Plane

coordinate

inverses

between

adjusted

coordinates

provide

adjusted

grid

azimuths

and

distances.

If

ground

level

distances

are

required,

the

adjusted

grid

distance

is

divided

by

the

combined

factor

that

was

previously

used

to

reduce

the

observed

distances.

Figure

4.4f

shows

the

adjusted

data.

61

BIBLIOGRAPHY

Adams,

o.s.,

1921:

Latitude

developments

connected

with

geodesy

and

cartography.

Special

Publication

67,

U.S.

Coast

and

Geodetic

Survey,

132

pp.

National

Geodetic

Information

Branch,

NGS,

NOAA,

Rockville,

MD

20852.

Adams,

Oscar

S,

and

Claire,

Charles

A.,

1948: Manual

of

plane

coordinate

computation.

Special

Publication

193,

Coast

and

Geodetic

Survey,

pp.

1-14.

National

Geodetic

Information

Branch,

NGS,

NOAA,

Rockville,

MD

20852.

Burkholder,

Earl

F.,

1984:

Geometrical

parameters

of

the

Geodetic

Reference

System 1980.

Surveying

and

Mapping, 44,

4,

339-340.

Claire,

C.N.,

1968:

State

plane

coordinates

by

automatic

data

processing.

Publication

62-4,

Coast

and

Geodetic

Survey,

68

pp.

National

Geodetic

Information

Branch,

NGS,

NOAA,

Rockville,

MD

20852.

Department

of

the

Army, 1958:

Universal

Transverse

Mercator

grid.

Technical

Manual TM5-241-8,

Washington,

D.C.

National

Technical

Information

Service,

Springfield,

VA

22161, Document No.

ADA176624.

Fronczek,

Charles

J.,

1977,

rev.

Memorandum

NOS

NGS-1

O,

38

pp.

NOAA,

Rockville,

MD

20852.

1980: Use

of

calibration

lines.

NOAA

Technical

National

Geodetic

Information

Branch,

NGS,

Jordan/Eggert/Kneissl,

1959: Handbuch

der

Vermessungskunde,

19th

ed.,

vol.

IV,

J.

B.

Metzlersche

Verlagsbuchhandlung,

Stuttgart.

Mitchell,

Hugh

C.

and

Simmons,

Lansing

G.,

1945,

rev.

1977:

The

State

coordinate

systems.

Special

Publication

235,

Coast

and

Geodetic

Survey,

62 pp.

National

Geodetic

Information

Branch,

NGS,

NOAA,

Rockville,

MD

20852.

Thomas,

Paul

D.,

1952: Conformal

projections

in

geodesy

and

cartography.

Special

Publication

251,

Coast

and

Geodetic

Survey,

142

pp.

National

Geodetic

Information

Branch,

NGS,

NOAA,

Rockville,

MD

20852.

Vincenty,

T.,

1985:

Precise

determination

of

the

scale

factor

from Lambert

conical

projection

coordinates.

Surveying

and

Mapping (American

Congress

on

Survyeing

and Mapping,

Fall

Church,

VA),

45, 4,

315-318.

Vincenty,

T.,

1986: Use

of

polynomial

coefficients

in

conversions

of

coordinates

on

the

Lambert

conformal

conic

projection.

Surveying

and

Mapping, 46,

1,

15-18.

Vincenty,

T.,

1986:

Lambert

conformal

conic

projection:

Arc-to-chord

correction.

Surveying

and

Mapping, 46,

2,

163-164.

62

APPENDIX

A.--DEFINING

CONSTANTS

FOR

THE

1983

STATE

PLANE

COORDINATE

SYSTEM

Transverse

Mercator

(T.M.),

Oblique

Mercator

(O.M.),

and

Lambert

(L.)

Projections

Central

Meridian

and

Scale

Factor

Grid

Origin

(T.

M.)

or

Standard

Longitude

Easting

State/Zone/Code

Projection

Parallels

(L.)

Latitude

Northing

(meters)

Alabama

AL

East

E

0101

T.M.

85

50

85

50

200,000.

1:25,000

30 30

0

West w

0102

T.M.

87

30

87

30

600,000.

1:15,000

30

00

0

-----------------------------------------------------------------------------

Alaska

AK

Zone 1

5001

O.M.

Axis

Azimuth

=

133

40

s.000,000.

arc

tan

-3/4

57

00

-5,000,000.

1:10,000

Zone 2

5002

T.M. 142 00

142 00

500,000.

1:10,000

54 00

0

Zone 3 5003

T.M.

146 00 146 00

500,000.

1:10,000

54 00

0

Zone

4

5004

T.M.

150 00

500,000.

1:10,000

54 00

0

Zone 5 5005

T.M.

154 00 154 00

500,000.

1:

10,000

54

00

0

Zone 6

5006

T.M.

158 00 158 00

500,000.

1:10,000

54 00 0

Zone 7 5007

T

.M.

162 00 162 00

500,000.

1:10,000

54

00 0

Zone 8

5008

T.M.

166 00 166 00

500,000.

1:10,000

54 00

0

Zone 9

5009

T.M.

170 00 170 00

500,000.

1:10,000

54 00

0

Zone

10

5010

L

51

50 176 00

1,000,000.

53

50

51

00

0

63

Central

Meridian

and

Scale

Factor

Grid

Origin

(T.M.)

or

Standard

Longitude

Easting

State/Zone/Code

Projection

Parallels

(L.)

Latitude

Northing

Arizona

AZ

East

E

Central

c

l.Jest

w

0201

T.M.

110

10

110

10

1:10,000

31

00

0202

T.M.

111

SS

111

SS

1:10,000

31

00

0203

T.M.

113

4S

113

4S

l:lS,000

31 00

(State

law

defines

the

origin

in

International

Feet)

(213,360M,

=

700,000

International

Feet)

213,360.

0

213,360.

0

213,360.

0

-----------------------------------------------------------------------------

Arkansas

AR

North

N 0301 L

34

S6

92

00

400,000.

36

14

34

20

0

South

s 0302 L

33

18

92

00

400,000.

34

46

32

40

400,000.

------------------------------------------------------------------------------

California

CA

Zone 1 0401 L

40

00

122 00

2,000.000.

41

40

39

20

soo.ooo.

Zone

2

0402

L

38

20

122 00

2,000.000.

39

so

37

40

S00,000.

Zone 3

0403

L

37

04

120

30

2,000,000.

38

26

36

30

S00,000.

Zone 4

0404

L

36

00 119

00

2,000.000.

37

lS

3S

20

S00,000.

Zone s 040S L

34

02 118 00

2,000,000.

3S

28

33

30

soo.ooo.

Zone 6

0406

L

32

47

116

lS

2,000,000.·

33

S3

32

10

soo.ooo.

-----------------------------------------------------------------------------

Colorado

co

North

N

OSOl

L

39

43

lOS

30

914,401.8289

40

47

39

20

304,800.6096

Central

c

OS02

L 38

27

lOS

30

914,401.8289

39 45

37

so

304

800.6096

South

s

0503 L

37

14

!OS

30 914

401.8289

38

26

36

40 304

800.

6096

64

State/Zone/Code

Connecticut

CT

0600

Delaware

DE

0700

Florida

FL

East

E

0901

West w

0902

North

N

0903

Georgia

GA

East

E

1001

West w 1002

Central

Meridian

and

Scale

Factor

(T.M,)

or

Standard

Projection

Parallels

(L.)

L

T.M.

L

T.M.

T

,M,

41

12

41

S2

7S

2S

1:200,000

81

00

1:17,000

82 00

1:17,000

29

3S

30 45

82

10

1:10,000

84 10

1:10,000

Grid

Origin

Longitude

Easting

Latitude

Northing

72

4S

40

so

7 s

2S

38 00

81

00

24

20

82

00

24 20

84 30

29 00

82

10

30 00

84

10

30

00

304.

800.

6096

lSZ,400.3048

200,000.

0

200,000.

0

200,000.

0

600,000.

0

200,000.

0

700,000.

0

------------------------------------------------------------------------------

Hawaii

HI

Zone

1

SlOl

T.M. 15S

30

lSS 30

S00,000.

1:

30,000

18

so

0

Zone

2

5102

T.M.

156

40

1S6

40

500,000.

1:30,000

20

0

Zone

3 5103 T

.M.

1S8

00

1S8

00

500,000.

1:100,000

21

10 0

Zone

4 5104

T.M.

159

30

1S9

30

500,000.

1:100,000

21

50 0

Zone

5 5105

T.M.

160

10

160 10

500,000.

0

21

40 0

65

Central

Meridian

and

Scale

Fae::

tor

Grid

Origin

(T

.M.)

or

Standard

Longitude

Easting

State/Zone/Code

Projection

Parallels

(L.)

Latitude

Northing

Idaho

ID

East

E

llOl

T .M.

112

10

ll2

10

200,000.

1:19,000

41

40

0

Central

c

1102

T.M. 114 00 114 00

500,000.

1:19,000

41

40

0

West

II

1103

T.M.

115 45

ll5

45

800,000.

1:15,000

41

40 0

Illinois

IL

East

E 1201

T.

M.

88 20 88

20

300,000.

1:40,000

36

40

0

West

II

1202

T.M.

90

10

90

10

700,000.

1:17,000

36

40

0

--------------------------------------------------------------------

----------

Indiana

IN

East

E

1301

T.M.

85 40

85

40

100, 000.

1:30,000

37

30

250,000.

West

II

1302

T.

M.

87

05

87

05

900,000.

1:30,000

37

30

250,000.

Iowa

IA

North

N

1401

L 42 04 93 30

1,500,000.

43

16

41

30

1,000,000.

South

s 1402 L 40

37

93 30

500,

000.

41

47

40

00

0

Kansas

KS

North

N 1501 L

38

43 98 00

400,000.

39

47

38

20

0

South

s 1502 L

37

16

98 30

400,000.

38 34 36

40

400,000.

Kentucky

KY

North

N 1601 L

37

58 84

15

500,000.

38

58

37 30

0

South

s 1602 L 36

44

85

45

500,000.

37

56

36

20

500,000.

-----------------------------------------------------------------------------

66

Central

Meridian

and

Scale

Factor

Grid

Origin

(T

.M.)

or

Standard

Longitude

Easting

State/Zone/Code

Projection

Parallels

(L.)

Latitude

Northing

Louisiana

LA

North

N 1701 L

31

10

92

30

1,000,000.

32

40 30 30* 0

South

s 1702 L

29

18

91

20

1,000,000.

30

42

28

30*

0

Offshore

SH

1703 L

26

10

91

20

1,000,000.

27

50

25

30* 0

------------------------------------------------------------------------------

Maine

ME

East

E

1801

T.M.

West w 1802 T.M.

Maryland

MD

1900 L

68 30

I:

10,000

70 10

1:30,000

38

18

39

27

68

30

43

40*

70

10

42 50

77

00

37

40*

300,000.

0

900,000.

0

400,000.

0

-----------------------------------------------------------------------------

Massachusetts

MA

Mainland

M

2001

L

41

43

71

30

200,000.

42 41

41

00

750,000.

Island

I 2002 L 41

17

70

30

500,000.

41

29

41

00 0

Michigan

MI

North

N 2111 L 45

29

87

00

8,000,000.

47

05

44 47 0

Central

c 2112 L 44

II

84 22*

6,000,000.

45 42

43

19

0

South

s 2113 L

42 06 84 22*

4,000,000.

43 40

41

30

0

Minnesota

MN

North

N 2201 L 47 02

93

06

800,000.

48

38

46

30

100,000.

Central

c 2202 L 45 37

94

15

800,000.

47 03

45 00

100,000.

South

s 2203

L 43

47

94

00

800,000.

45

13 43 00

100,000.

------------------------------------------------------------------------------

67

Central

Meridian

and

Scale

Factor

Grid

Origin

(T.M.)

or

Standard

Longitude

Easting

State/Zone/Code

Proi

ection

Parallels

(L.)

Latitude

Northing

Mississippi

MS

East

E

2301 T.M. 88

so

88

so

300,000.

1:20,000*

29

30* 0

West w 2302 T.M. 90

20

90 20

700,000.

1:20,000*

29

30*

0

------------------------------------------------------------------------------

Missouri

MO

East

E 2401

T.M.

Central

c 2402 T.M.

West

w 2403 T.M.

Montana

MT

2500

L

Nebraska

NE

2600 L

Nevada

NV

East

E 2701 T.M.

Central

c 2702

T,M,

West

w 2703 T .M.

90 30

1:15,000

92

30

l:lS,000

94

30

1:17,000

45

OD*

49

OD*

40

00*

43 00*

115

35

1:10,000

116 40

1:10,000

118

35

1:10,000

90

30

35

50

92

30

3S

so

94

30

36 10

109 30

44 15*

100

DO*

39

50*

115 35

34

45

116 40

34

4S

118

3S

34

45

2SO,OOO.

0

S00,000.

0

850,000.

0

600,000.

0

500,000.

0

200,000.

8,000,000.

500,000.

6,000,000.

800,000.

4,000,000.

------------------------------------------------------------------------------

New

Hampshire

NH

2800

T

.M.

New

Jersey

NJ

(New

York

East)

2900

T.M.

68

71

40

1:30,000

74

30*

1:10,000*

71

40

42 30

74

30*

38 50

300,000.

0

lS0,000.

0

Central

Meridian

and

Scale

Factor

Grid

Origin

(T,

M.)

or

Standard

Longitude

Easting

State/Zone/Code

Projection

Parallels

(L.)

Latitude

Northing

New

Mexico NM

East

E 3001 T.M. 104

20

104 20 16S,OOO.

1:11,000

31

00 0

Central

c 3002

T.M.

106

IS

106 IS

S00,000.

1:10,000

31

00 0

West w

3003

T.M.

107

so

107

so

830,000.

1:12,000

31

00

0

------------------------------------------------------------------------------

New

York

NY

East

E

3101 T

.t-1.

74

30*

74

30*

IS0,000.

(New

Jersey)

1:10,000*

38 50*

0

Central

c 3102 T

.1'1.

76

3S

76

3S

2SO,OOO.

1:16,000

40 00

0

West w 3103

T.M.

78

35

78

35

3SO,OOO.

1:16,000

40 00

0

Long

Island

L 3104 L 40 40

74

00

300,000.

41

02 40 10* 0

------------------------------------------------------------------------------

North

Carolina

NC

3200 L

North Dakota

ND

North

N 3301 L

South

S 3302 L

Ohio

OH

North

N

3401

L

South

s 3402

L

34

20

36

10

47

26

48 44

46

II

47

29

40

26

41

42

38 44

40

02

79

00

33

4S

100

30

47

00

.

JOO

30

4S

40

82

30

39

40

82

30

38

00

609,601.22

0

600,000.

0

600,000.

0

600,000.

0

600,000.

0

-----------------------------------------------------------------------------

Oklahoma

OK

North

N 3501

L

3S

34

98

00

600,000.

36

46

35

00 0

South

s 3S02 L 33 56

98 00

600,000.

35

14

33

20

0

69

State/Zone/Code

Projection

Oregon

OR

North

N

3601

L

South

s 3602

L

Pennsylvania

PA

North

N 3701 L

South

s 3702 L

Rhode

Island

RI

3800 T.M.

South

Carolina

SC

3900 L

South

Dakota

SD

North

N 4001 L

South

s

4002

L

Tennessee

TN

4100

L

Central

Meridian

and

Scale

Factor

(T.M.)

or

Standard

Parallels

(L.)

70

44 20

46 00

42 20

44 00

40

53

41

57

39 56

40

58

71

30

1:160,000

32 30*

34

50*

44

25

45

41

42

so

44

24

35

15

36

25

Grid

Origin

1.ongitude

Latitude

120

30

43

40

120 30

41

40

77

45

40

10

77

45

39 20

71

30

41

05

81

00*

31

50*

100 00

43 50

100

20

42 20

86

00

34

20*

Easting

Northing

2,500,000.

0

1,500,000.

0

600,000.

0

600,000.

0

100,000.

0

609,600.

0

600,000.

0

600,000.

0

600,000.

0

Central

Meridian

and

Scale

Factor

Grid

Origin

(T

.M.)

or

Standard

Longitude

Easting

State/Zone/Code

Projection

Parallels

(L.)

Latitude

Northing

Texas

TX

North

N 4201 L 34

39

101

30

200.000.

36

11

34

00

1,000,000.

North

Central

NC

4202 L

32

08

98

30*

600,000.

33

58

31

40

2,000,000.

Central

c 4203 L 30

07

100

20

700,000.

31

53

29

40

3,000,000.

South

Central

SC

4204 L

28

23

99

00

600,000.

30

17

27

50

4,000,000.

South

s 4205 L

26

10

98 30

300,000.

27

50

25

40

5,000,000.

-----------------------------------------------------------------------------

Utah

UT

North

N 4301 L

CentraJ. c 4302 L

South

s

4303

L

Vermont

VT

4400

T.M.

Virginia

VA

North

N

4501 L

South

s

4502

L

40

43

41

47

39

01

40

39

37

13

38

21

72 30

1:28,000

38

02

39

12

36

46

37

58

111 30

40

20

111 30

38

20

111 30

36

40

72

30

42

30

78

30

37

40

78

30

36 20

500,000.

1,000,000.

500,000.

2,000.000.

500,000.

3,000,000.

500,000.

0

3,500,000.

2,000,000.

3,500,000.

1,000,000.

------------------------------------------------------------------------------

Washington

WA

North

N 4601 L 47 30

120 50

500,000.

48 44

47

00

0

South

s 4602 L 45

so

120 30

500,000.

47 20

45 20

0

71

Central

Meridian

and

Scale

Factor

Grid

Origin

(T

.M.)

or

Standard

Longitude

Easting

State/Zone/Code

Projection

Parallels

(L.)

Latitude

Northing

West

Virginia

WV

North

N 4701

L

39

00

79

30

600,000.

40

15

38 30

0

South

s 4702 L

37

29

81

00

600,000.

38 53

37

00 0

------------------------------------------------------------------------------

Wisconsin

WI

North

N 4801 L

45

34

90

00

600,000.

46 46

45

10

0

Central

c 4802

L

44

15

90

00

600,000.

45 30

43

so

0

South

s 4803 L

42

44

90

00

600,000.

44

04

42 00 0

-----------------------------------------------------------------------------

Wyoming

WY

East

E

East

Central

EC

West

Central

WC

West

w

Puerto

Rico

PR

and

Virgin

Islands

4901

T.M.

4902

T.M.

4903

T.M.

4904

T.M.

5200 L

105

10

1:16,000*

107 20

1:16,000•

108 45

1:16,000*

110

05

1:16,000*

18 02

18

26

105

10

40 30*

107 20

40 30*

108 45

40 30*

110 05

40 30*

66

26

17

50

200,000.

0

400,000.

100,000

600,000.

0

800,000.

100,000.

200,000.

*

This

represents

a

change

from

the

defining

constant

used

for

the

1927

State

Plane

Coordinate

System.

All

metric

values

assigned

to

the

origins

also

are

changes.

72

APPENDIX

B.

MODEL

ACT

FOR

STATE

PLANE

COORDINATE

SYSTEMS

An

act

to

describe,

define,

and

officially

adopt

a

system

of

coordinates

for

designating

the

geographic

posit.ion

of

points

on

the

surface

of

the

Earth

within

the

State

of

.•••••••.....

BE

IT

ENACTED

BY

THE

LEGISLATURE

OF

THE

STATE

OF

•.....•••.

Section

1.

The

systems

of

plane

coordinates

which

have

been

established

by

the

National

Ocean

Service/National

Geodetic

Survey

(formerly

the

United

States

Coast

and

Geodetic

Survey)

or

its

successors

for

defining

and

stating

the

geographic

positions

or

locations

of

points

on

the

surface

of

the

Earth

within

the

State

of

••.....•••

are

hereafter

to

be

known

and

designated

as

the

..

,

...••••

(name

of

State)

Coordinate

System

of

1927 and

the

.•••••..

, , (name

of

State)

Coordinate

System

of

1983.

For

the

purpose

of

the

use

of

these

systems,

the

State

i.s

divided

i.nto

a ,

.•••

Zone and a

...•.

zone

(or

as

many

zone

identifications

as

now

defined

by

the

National

Ocean

Service.

The

area

now

included

in

the

following

cotlnties

shall

constitute

the

• ,

...

Zone:

(here

enumerate

the

name

of

the

counties

included),

The

area

now

included

.•••.

(likewise

for

all

zones).

Section

2.

As

established

for

use

in

the

••.••

zone,

the

..••••••••

(name

of

State)

Coordinate

System

of

1927

or

the

• , , , , , , • , • (name

of

State)

Coordinate

System

of

1983

shall

be named; and

in

any

land

description

in

which

it

is

used,

it

shall

be

designated

the"··········

(name

of

State)

Coordinate

System 1927

•...•

Zone

11

or

••••••••••

(name

of

State)

Coordinate

System

of

1983

•...•

Zone."

As

established

for

use

..•••

(likewise

for

all

zones).

Section

3.

The

plane

coordinate

values

for

a

point

on

the

Earth's

surface,

used

to

express

the

geographic

position

or

location

of

such

point

in

the

appropriate

zone

of

this

system,

shall

consist

of

two

distances

exµ"essed

in

U.S.

Survey

Feet

and

decimals

of

a

foot

when

using

the

..•••.••••

(name

of

State)

Coordinate

System

of

1927

and

expressed

in

meters

and

decimals

of

a

meter

when

using

the

••••••••..

(name

of

State)

Coordinate

System

of

1983.

For

SPCS

27,

one

of

these

distances,

to

be

known

as

the

"x-coordinate,"

shall

give

the

position

in

an

east-and-west

direction;

the

other,

to

be

known

as

the

"y-coordinate,"

shall

give

the

position

in

a

north-and-south

direction.

For

SPCS

83,

one

of

the

distances,

to

be

known

as

the

"northing"

or

"N",

shall

give

the

position

in

a

north-and-south

direction;

the

other,

to

be

known

as

the

"easting"

or

"E"

shall

give

the

position

in

an

east-and-west

direction.

These

coordinates

shall

be

made

to

depend upon and conform

to

plane

rectang11lar

coordinate

values

for

the

monumented

points

of

the

North

American

National

Geodetic

Horizontal

Network

as

published

by

the

National

Ocean

Service/National

Geodetic

Survey

(formerly

the

United

States

Coast

and

Geodetic

Survey),

or

its

successors,

and whose

plane

coordinates

have been computed on

the

systems

defined

in

this

chapter,

Any

such

station

may

be

used

for

establishing

a

survey

connection

to

either

•...•••••.

(name

of

State)

Coordinate

System.

73

Section

4. For

purposes

of

describing

the

location

of

any

survey

station

or

land

boundary

corner

in

the

State

of

.••••..•..

,

it

shall

be

considered

a

complete,

legal,

and

satisfactory

description

of

such

location

to

give

the

position

of

said

survey

station

or

land

boundary

corner

on

the

system

of

plane

coordinates

defined

in

this

act.

Nothing

contained

in

this

act

shall

require

a

purchaser

or

mortgagee

of

real

property

to

rely

wholly

on a

land

description,

any

part

of

which

depends

exclusively

upon

either

•••.•••...

(name

of

State)

coordinate

system.

Section

5.

When

any

tract

of

land

to

be

defined

by a

single

description

extends

from

one

into

the

other

of

the

above

coordinate

zones,

the

position

of

all

points

on

its

boundaries

may

be

referred

to

either

of

the

two

zones,

the

zone

which

is

used

being

specifically

named

in

the

description.

Section

6.

(a)

For

purposes

of

more

precisely

defining

the

••.....•••

(name

of

State)

Coordinate

System

of

1927,

the

following

definition

by

the

United

States

Coast

and

Geodetic

Survey

(now

National

Ocean

Service/National

Geodetic

Survey)

is

adopted:

(For

Lambert

zones)

The

11

••••••••••

(name

of

State)

Coordinate

System

of

1927

.....

(Zone ID)

Zone,"

is

a

Lambert

conformal

conic

projection

of

the

Clarke

s

pher

oi

d

of

1

866,

having

standard

parallels

at

north

latitudes

.•...

degrees

•••..

minutes

and

••...

degrees

....•

minutes

along

which

parallels

the

scale

shall

be

exact.

The

origin

of

coordinates

is

at

the

intersection

of

the

meridian

•..••

degrees

....•

minutes

west

of

Greenwich

and

the

parallel

•••.•

degrees

.••..

minutes

north

latitude.

This

origin

is

given

the

coordinates:

x =

••••••••••

feet

and y =

.•••......

feet

(as

now

defined).

(Use

similar

paragraphs

for

other

Lambert

zones

on

the

1927 Datum.)

(For

transverse

Mercator

zones)

The "

...•.•••••

(name

of

State)

Coordinate

System

of

1927

.••••

(Zone

ID)

zone,"

is

a

transverse

Mercator

projection

of

the

Clarke

spheroid

of

1866,

having

a

central

meridian

•.•..

degrees

•••••

minutes

west

of

Greenwich,

on

which

meridian

the

scale

is

set

one

part

in

•••••

too

small.

The

origin

of

coordinates

is

at

the

intersection

of

the

meridian

•••••

degrees

••..•

minutes

west

of

Greenwich

and

the

parallel

••...

degrees

•••••

minutes

north

latitude.

This

origin

is

given

the

coordinates:

x =

••••••••••

feet

and

y =

••••••••••

feet

(as

now

defined).

(Use

similar

paragraphs

for

other

transverse

Mercator

zones

on

the

1927 Datum).

(b)

For

purposes

of

more

precisely

defining

the

••••••..••

(name

of

State)

Coordinate

System

of

1983,

the

following

definition

by

the

National

Ocean

Service/National

Geodetic

Survey

is

adopted:

(For

Lambert

zones)

The"··········

(name

of

State)

Coordinate

System

of

1983

.•...

(Zone ID) Zone"

is

a Lambert

conformal

conic

projection

of

the

North

American Datum

of

1983,

having

standard

parallels

at

north

latitudes

•••••

degrees

..•..

minutes

and

•....

degrees

.....

minutes

along

which

parallels

the

scale

shall

be

exact.

The

origin

of

coordinates

is

at

the

intersection

of

the

meridian

•••.•

degrees

•.•••

minutes

west

of

Greenwich

and

the

parallel

••••.

degrees

...••

minutes

north

latitude.

This

origin

is

given

the

coordinates:

N

~

..•••.••••

meters

and

E

•..••.....

meters.

(Use

similar

paragraphs

for

other

Lambert

zones

on

the

1983

Datum).

(For

transverse

Mercator

zones)

The

''···

.......

(name

of

State)

Coordinate

System

of

1983

•.••.

(Zone ID)

Zone,"

is

a

transverse

Mercator

projection

of

the

North

American

Datum

of

1983,

having

a

central

meridian

....•

degrees

.....

minutes

west

of

Greenwich,

on which

meridian

the

scale

is

set

one

part

in

•••..

too

small.

The

origin

of

coordinates

is

at

the

intersection

of

the

meridian

•....

degrees

•••••

minutes

west

of

Greenwich

and

the

parallel

••...

degrees

..•..

minutes

north

latitude.

This

origin

is

given

the

coordinates:

N

~

....•......

meters

and

E =

••••••••••

meters.

(Use

similar

paragraphs

for

other

transverse

Mercator

zones

on

the

1983 Datum.)

Section

7,

No

coordinates

based

on

either

••••••••.•

(name

of

State)

coordinate

system,

purporting

to

define

the

position

of

a

point

on a

land

boundary,

shall

be

presented

to

be

recorded

in

any

public

land

records

or

deed

records

unless

such

point

is

within

1

kilometer

of

a monumented

horizontal

control

station

established

in

conformity

with

the

standards

of

accuracy

and

specifications

for

first-

or

second-order

geodetic

surveying

as

prepared

and

published

by

the

Federal

Geodetic

Control

Committee

(FGCC)

of

the

United

States

Department

of

Commerce.

Standards

and

specifications

of

the

FGCC

or

its

successor

in

force

on

date

of

said

survey

shall

apply.

Publishing

existing

control

stations,

or

the

acceptance

with

intent

to

publish

the

newly

established

stations,

by

the

Natinal

Ocean

Service/National

Geodetic

Survey

will

constitute

evidence

of

adherence

to

the

FGCC

specifications.

Above

limitations

may

be

modified

by a

duly

authorized

State

agency

to

meet

local

conditions.

Section

8.

The

use

of

the

term

11

••••••••••

(name

of

State)

Coordinate

System

of

1927 • ,

••••••.•

Zone

11

or

11

••••••••••

(name

of

State)

Coordinate

System

of

1983

.••••.....

Zone" on

any

map,

report

of

survey,

or

other

document

shall

be

limited

to

coordinates

based

on

the

.•.....••.

(name

of

State)

coordinate

system

as

defined

in

this

act.

Section

9.

If

any

provision

of

this

act

shall

be

declared

invalid,

such

invalidity

shall

not

affect

any

other

portion

of

this

act

which

can

be

given

effect

without

the

invalid

provision;

and

to

this

end,

the

provisions

of

this

act

are

declared

severable.

Section

10.

The

•......••.

(name

of

State)

Coordinate

System

of

1927

shall

not

be

used

after

.........•

(date);

the

.••.•...••

(name

of

State)

Coordinate

System

of

1983

will

be

the

sole

system

after

this

date.

(Note:

This

model

act

was

prepared

in

1977.

In

light

of

GPS

technology,

the

1

kilometer

limitation

of

Section

7

should

be

reevaluated.)

75

APPENDIX

C.--CONSTANTS

FOR THE LAMBERT PROJECTION

BY

THE POLYNOMIAL COEFFICIENT METHOD

Constants

Bs

=

Bn

=

Bb

=

Lo

=

Nb

=

Eo

=

Bo

=

SinBo=

Rb

=

Ro

=

K =

No

=

ko

Mo

=

ro

Description

Southern

standard

parallel

Northern

standard

parallel

Latitude

of

grid

origin

Longitude

of

the

true

and

grid

origin,

the

"central

meridian"

Northing

value

at

grid

orgin

"Bb"

Easting

value

at

the

origin

"Lo"

Latitude

of

the

true

projection

origin,

the

"central

parallel"

Sine

of

Bo

Mapping

radius

at

Bb

Mapping

radius

at

Bo

Mapping

radius

at

the

equator

Northing

value

at

the

true

projection

origin

"Bo"

Central

parallel

grid

scale

factor

Scaled

radius

of

curvature

in

the

meridian

at

"Bo"

Geometric

mean

radius

of

curvature

at

Bo

scaled

to

the

grid

Bs,

Bn, Bb,

and

Lo

in

degrees:

minutes

Bo

in

decimal

degrees

Linear

units

in

meters

(See

page

44

for

equivalent

notation

of

defining

and

derived

constants

used

in

the

figure

below.)

PARAMETERS OF A LAMBERT PROJECTION

+o

(With Scale Feotor ko)

76

AK 10

ALASKA 10

ZONE#

5010

Defining

Constants

Coefficients

for

GP

to

PC

Bs

=

51:50

L(l)

111266.2938

Bn

=

53:50

L(2)

=

9.42762

Bb

=

51:00

L(3)

=

5.60521

Lo

=

176:00

L(4)

=

0.032566

Nb

=

0.0000

L(5)

=

0.0008745

Eo

=

1000000.0000

Coefficients

for

PC

to

GP

Computed

Constants

G(l)

=

8.987447734E-06

Bo

=

52.8372090915

G(2)

-6.84405E-l5

SinBo=

0.796922389486

G (

3)

-3.65605E-20

Rb

5048740.3829

G(4)

=

-l,7676E-27

Ro

=

4844318.3515

G(5)

=

-9.l43E-36

No

204422.0314

K

=

11499355.8664

Coefficients

for

Grid

Scale

Factor

ko

=

0.999848059991

Mo

=

6375089.0366

F(l)

=

0.999848059991

ro

=

6382923,

F(2)

=

l.22755E-l4

F(3)

=

8.34E-22

77

AR

N

ARKANSAS NORTH

Defining

Constants

Bs

=

34:56

Bn

36:14

Bb

=

34:20

Lo

=

92:00

Nb

0.0000

Eo

=

400000.0000

Computed

Constants

Bo

=

35.5642263444

SinBo=

0.561699126039

Rb

9062395.1961

Ro

=

6923619.0696

No

=

136776.1285

K

=

13112784.4998

ko

=

0.999935935348

Mo

6356634.6561

ro

6370786.

AR

s ARKANSAS SOUTH

Defining

Constants

Bs

=

33:16

Bn

=

34:46

Bb

=

32:40

Lo =

92:00

Nb

=

400000.0000

Eo

=

400000.0000

Computed

Constants

Bo

=

34.0344093756

Sin

Bo=

0.559690686632

Rb

9604584.2290

Ro

=

9452884.9686

No

=

551699.2604

K =

13438989.7695

ko

0.999918469533

Mo

=

6354902.0291

ro

=

6369591.

ZONE#

0301

Coefficients

for

GP

to

PC

L(l)

=

110944.2037

L(2)

=

9.22246

L(3)

=

5.64616

L(4)

=

0.017597

Coefficients

for

PC

to

GP

G(l)

=

9.013539789E-06

G(2)

=

-6.75356E-15

G(3)

=

-3.72463E-20

G(4)

=

-9.0676E-28

Coefficients

for

Grid

Scale

Factor

F(l)

0.999935935348

F(2)

=

l.23195E-14

F(3)

=

4.52E-22

ZONE#

0302

Coefficients

for

GP

to

PC

L(l)

=

110913.9635

L(2)

=

9.03498

L(3)

=

5.64949

L(4)

=

0.016534

Coefficients

for

PC

to

GP

G(l)

=

9.015997271E-06

G(2)

=

-6.62159E-15

G(3)

=

-3.73079E-20

G(4)

=

-8.5429E-2B

Coefficients

for

Grid

Scale

Factor

F(l)

=

0.999918469533

F(2)

=

l.23240E-14

F(3)

=

4.26E-22

78

CA

01

CALIFORNIA 1

ZONE#

0401

Defining

Constants

Coefficients

for

GP

to

PC

Bs =

40:00

L(l)

=

111039.0203

Bn

41:40

L(2)

=

9.65524

Bb =

39:20

L(3)

=

5.63491

Lo =

122:00

L(4)

=

0.021275

Nb

500000.0000

Eo

=

2000000.0000

Coefficients

for

PC

to

GP

Computed

Constants

G (

1)

=

9.005843038E-06

Bo =

40.8351061249

G(2)

=

-7.05240E-15

Sin

Bo=

0.653884305400

G (

3)

-3.70393E-20

Rb

7556554.6408

G(4)

=

-l.1142E-27

Ro

=

7389802.0597

No

=

666752.5811

K

=

12287826.3052

Coefficients

for

Grid

Scale

Factor

ko

=

0.999894636561

Mo

=

6362067.2798

F(l)

=

0.999894636561

ro

=

6374328.

F(2)

=

l.23062E-14

F(3)

=

5.47E-22

CA

02

CALIFORNIA 2

ZONE#

0402

Defining

Constants

Coefficients

for

GP

to

PC

Bs

=

38:20

L(l)

=

111007.6240

Bn

=

39:50

L(2)

=

9.54628

Bb

=

37:40

L(3)

=

5.63874

Lo

=

122:00

L(4)

=

0.019988

Nb =

500000.0000

Eo

=

2000000.0000

Coefficients

for

PC

to

GP

Computed

Constants

G(l)

=

9.008390180E-06

Bo =

39.0846839219

G(2)

=

-6.97872E-15

SinBo=

0.630468335285

G(

3)

=

-3.71084E-20

Rb =

8019788.9307

G(4)

=

-l.0411E-27

Ro

=

7862381.4027

No

=

657407.5280

K =

12520351.6538

Coefficients

for

Grid

Scale

Factor

ko

=

0.999914672977

MO

=

6360268.3937

F(l)

=

0.999914672977

ro

=

6373169.

F(2)

=

l.23106E-14

F(3)

=

5.14E-22

79

CA

03 CALIFORNIA 3

ZONE#

0403

Defining

Constants

Coefficients

for

GP

to

PC

Bs

37:04

L(l)

=

110983.9104

Bn

38:26

L(2)

=

9.43943

Bb

=

36:30

L(3)

=

5.64142

Lo

=

120:30

L(4)

0.019048

Nb

=

500000.0000

Eo

=

2000000.0000

Coefficients

for

PC

to

GP

Computed

Constants

G(

1)

=

9.010315015E-06

Bo

=

37.7510694363

G(2)

=

-6.90503E-15

SinBo=

0.612232038295

G(

3)

=

-3.71614E-20

Rb

=

8385775.1723

G(4)

=

-9.8819E-28

Ro

=

8246930.3684

No

=

638844.8039

K

=

12724574.9735

Coefficients

for

Grid

Scale

Factor

ko

=

0.999929178853

Mo

6358909.6841

F(l)

=

0.999929178853

ro

6372292.

F(2)

=

l.23137E-14

F(3)

4.89E-22

CA

04 CALIFORNIA 4

ZONE#

0404

Defining

Constants

Coefficients

for

GP

to

PC

Bs

36:00

L(l)

=

110964.0696

Bn

=

37:15

L (

2)

=

9.33334

Bb =

35:20

L (

3)

=

5.64410

Lo

=

119:00

L(4)

=

0.018382

Nb

=

500000.0000

Eo

=

2000000.0000

Coefficients

for

PC

to

GP

Computed

Constants

G(l)

=

9.011926076E-06

Bo

36.6258593071

G (

2)

=

-6.83121E-15

SinBo=

0.596587149880

G (

3)

=

-3.72043E-20

Rb =

8733227.3793

G(

4)

=

-9.4223E-28

Ro

=

8589806.8935

No =

643420.4858

K =

12916986.0281

Coefficients

for

Grid

Scale

Factor

ko

=

0.999940761703

Mo

=

6357772.8978

F(l)

=

0.999940761703

ro

=

6371557.

F(2)

=

l.23168E-14

F(3)

=

4.70E-22

80

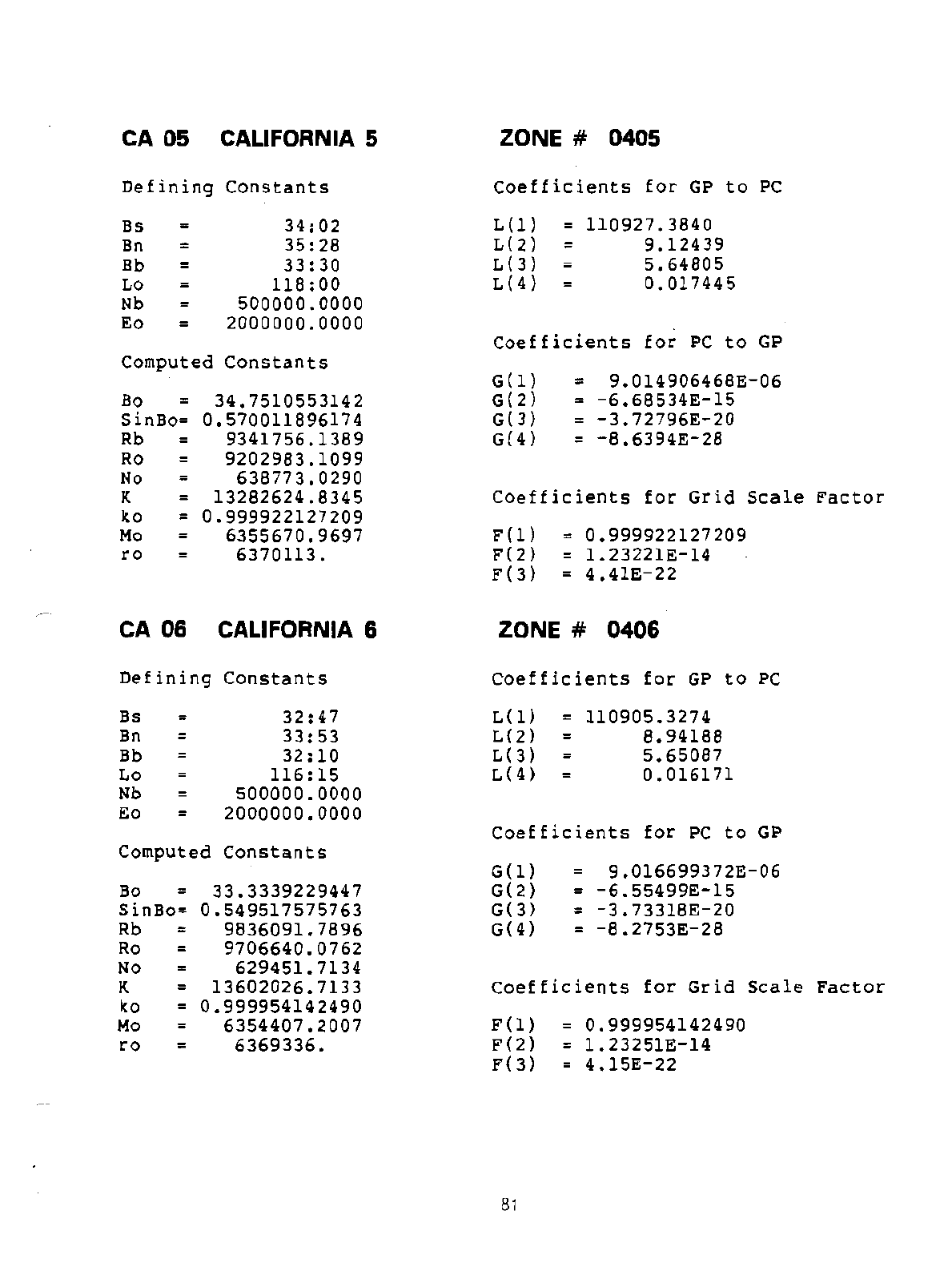
CA

05 CALIFORNIA 5

ZONE#

0405

Defining

Constants

Coefficients

for

GP

to

PC

Bs

=

34:02

L(l)

=

110927.3840

Bn

=

35:28

L(2)

=

9.12439

Bb

=

33:30

L(3)

=

5.64805

LO

=

118:00

L(4)

=

0.017445

Nb

=

500000.0000

Ee

=

2000000.0000

Coefficients

for

PC

to

GP

Computed

Constants

G (

1)

=

9.014906468E-06

Bo

=

34.7510553142

G (

2)

=

-6.68534E-15

SinBo=

0.570011896174

G(

3)

-3,72796E-20

Rb

=

9341756.1389

G(

4)

=

-B.6394E-28

Ro

=

9202983.1099

No

=

638773.0290

K

=

13282624.8345

Coefficients

for

Grid

Scale

Factor

kc

=

0.999922127209

Mo

=

6355670.9697

F(l)

=

0.999922127209

re

=

6370113.

F(2)

=

l.23221E-14

F(3)

=

4.4lE-22

CA

06

CALIFORNIA 6

ZONE#

0406

Defining

Constants

Coefficients

for

GP

to

PC

Bs

=

32:47

L(l)

=

110905.3274

Bn

=

33:53

L(2)

=

8.94188

Bb

32:10

L(3)

=

5.65087

Lo

116:15

L(4)

=

0.016171

Nb

=

500000.0000

Ee

=

2000000.0000

Coefficients

for

PC

to

GP

Computed

Constants

G(

l)

9.016699372E-06

Bo

=

33.3339229447

G(

2)

=

-6.55499E-15

SinBo=

0.549517575763

G(

3)

=

-3.73318E-20

Rb

=

9836091.7896

G(4)

=

-8.2753E-28

Ro

=

9706640.0762

No

=

629451.7134

K

=

13602026.7133

Coefficients

for

Grid

Scale

Factor

kc

=

0.999954142490

Mo

=

6354407.2007

F(l)

=

0.999954142490

re

=

6369336.

F(2)

=

l.

23251E-14

F(3)

=

4.15E-22

81

co

N COLORADO NORTH

Defining

Constants

Bs

39:43

Bn

40:47

Bb

=

39:20

Lo

105:30

Nb

=

304800.6096

Eo

=

914401.8289

Computed

Constants

Bo

=

40.2507114537

SinBo=

0.646133456811

Rb

=

7646051.6244

Ro

=

7544194.6172

No

=

406657.6168

K

=

12361909.8309

ko

=

0.999956846063

Mo

=

6361817.5470

ro

=

6374293.

co

c COLORADO CENTRAL

Defining

Constants

Bs

=

38:27

Bn

=

39:45

Bb

=

37:50

Lo =

105:30

Nb

=

304800.6096

Eo

914401.8289

Computed

Constants

Bo

=

39.1010150117

SinBo=

0.630689555225

Rb

7998699.7391

Ro

=

7857977.9317

No

=

445522.4170

K =

12518269.8410

ko

0.999935909777

Mo

=

6360421.3434

ro

=

6373316.

ZONE#

0501

Coefficients

for

GP

to

PC

L(l)

=

111034.6624

L(2)

=

9.62324

L(3)

=

5.63555

L(4)

=

0.021040

Coefficients

for

PC

to

GP

G(l)

=

9.006196586E-06

G(2)

=

-7.02998E-15

G(3)

=

-3.70588E-20

G(4)

=

-l.0841E-27

Coefficients

for

Grid

Scale

Factor

F(l)

=

0.999956846063

F(2)

=

l.23060E-14

F(3)

=

5.37E-22

ZONE#

0502

Coefficients

for

GP

to

PC

L(l)

=

111010.2938

L(2)

=

9.54770

L(3)

=

5.63848

L(4)

=

0.019957

Coefficients

for

PC

to

GP

G(l)

9.008173565E-06

G(2)

=

-6.97922E-15

G(3)

=

-3.71064E-20

G(4)

=

-l.0428E-27

Coefficients

for

Grid

Scale

Factor

F(l)

=

0.999935909777

F(2)

=

l.23099E-14

F(3)

=

5.14E-22

82

co s COLORADO SOUTH

Defining

Constants

BS

=

37:14

Bn

38:26

Bb

=

36:40

Lo =

105:30

Nb

304800.6096

Eo

=

914401.8289

Computed

Constants

Bo

=

37.8341602703

SinBo=

0.613378042371

Rb

8352015.4059

Ro

=

8222442.4013

No

=

434373.6143

K

=

12711335.3256

ko

=

0.999945398499

Mo

=

6359102.7444

ro

6372455.

ZONE#

0503

Coefficients

for

GP

to

PC

L(l)

=

110987.2800

L(2)

9.44685

L(3)

=

5.64118

L(4)

=

0.019105

Coefficients

for

PC

to

GP

G(l)

=

9.010041469E-06

G(2)

=

-6.90983E-15

G(3)

-3.71567E-20

G(4)

=

-9.9134E-28

Coefficients

for

Grid

Scale

Factor

F(l)

=

0.999945398499

F(2)

=

l.23131E-14

F(3)

=

4.91E-22

83

CT

CONNECTICUT

ZONE#

0600

Defining

Constants

Coefficients

for

GP

to

PC

Bs

=

41:12

L(l)

=

111062.3637

Bn

41:52

L(2)

=

9.68962

Bb

=

40:50

L(3)

=

5.63247

Lo =

72:45

L(4)

=

0.021924

Nb =

152400.3048

Eo =

304800.6096

Coefficients

for

PC

to

GP

Computed

Constants

G(l)

=

9.003950270E-06

Bo =

41.5336239347

G(2)

-7.07309E-15

SinBo=

0.663059457532

G (

3)

=

-3.70044E-20

Rb

=

7288924.5189

G (

4)

=

-l.1414E-27

Ro

=

7211151.4122

No

=

230173.4115

K =

12206545.8602

Coefficients

for

Grid

Scale

Factor

ko

=

0.999983140478

Mo

6363404.7042

F(l)

=

0.999983140478

ro

6375409.

F(2)

=

l.23017E-14

F(3)

=

5.61E-22

84

FL

N

FLORIDA

NORTH

Defining

Constants

Bs

=

29:35

Bn

=

30:45

Bb

=

29:00

Lo

=

84:30

Nb

0.0000

Ea

=

600000.0000

Computed

Constants

Bo

=

30.1672535540

SinBo=

0.502525902671

Rb

=

11111265.2070

Ro

=

10981878.2256

No

=

129386.9814

K

14473086.8984

ko

=

0.999948432740

Mo

=

6351211.3497

ro

6367189.

ZONE#

0903

Coefficients

for

GP

to

PC

L(l)

=

110849.5492

L(2)

=

8.45478

L(3)

=

5.65723

L(4)

=

0.014285

Coefficients

for

PC

to

GP

G(l)

=

9.02l236462E-06

G(2)

=

-6.20727E-15

G(3)

-3.74501E-20

G(4)

=

-7.2421E-28

Coefficients

for

Grid

Scale

Factor

F(l)

=

0.999948432740

F(2)

=

l.23332E-14

F(3)

=

3.67E-22

85

IA

N IOWA NORTH

ZONE#

1401

Defining

Constants

Coefficients

for

GP

to

PC

Bs

=

42:04

L(l)

=

111080.1947

Bn

=

43:16

L(2)

=

9.73155

Bb =

41:30

L(3)

=

5.63034

Lo =

93:30

L (

4)

=

0.022691

Nb =

1000000.0000

Eo =

1500000.0000

Coefficients

for

PC

to

GP

Computed

Constants

G(l)

=

9.002504865E-06

Bo

=

42.6676459541

G(2)

-7.10023E-15

SinBo=

0.677744566795

G(

3)

=

-3.69607E-20

Rb

7059740.0263

G(4)

=

-l.1953E-27

Ro

=

6930042.0331

No

=

1129697.9931

K

=

12083972.0985

Coefficients

for

Grid

Scale

Factor

ko

=

0.999945367870

Mo

=

6364426.3661

F(l)

=

0.999945367870

ro

=

6376011.

F(2)

=

l.22997E-14

F(3)

=

5.83E-22

IA s

IOWA SOUTH

ZONE#

1402

Defining

Constants

Coefficients

for

GP

to

PC

Bs

40:37

L(l)

=

111052.0582

Bn

=

41:47

L(2)

9.67367

Bb

40:00

L(3)

5.63393

Lo

=

93:30

L(4)

=

0.021895

Nb

=

0.0000

Eo

500000.0000

Coefficients

for

PC

to

GP

Computed

Constants

G(

1)

=

9.004785763E-06

Bo =

41.2008797613

G(2)

=

-7.06375E-15

SinBo=

0.658701013169

G(

3)

=

-3.70197E-20

Rb =

7429044.5139

G (

4)

-l.1221E-27

Ro

=

7295688.5838

No

=

133355.9301

K =

12244655.5752

Coefficients

for

Grid

Scale

Factor

ko

=

0.999948369709

Mo

=

6362814.2760

F(l)

=

0.999948369709

ro

=

6374941.

F(2)

l.23041E-14

F(3)

=

5.56E-22

86

KS

N KANSAS NORTH

ZONE#

1501

Defining

Constants

Coefficients

for

GP

to

PC

Bs

=

38:43

L(l)

=

111015.4786

Bn

=

39:47

L(2)

=

9.55844

Bb

38:20

L(3)

=

5.63780

Lo

=

98:00

L(4)

0.020306

Nb

0.0000

Eo =

400000.0000

Coefficients

for

PC

to

GP

Computed

Constants

G (

1)

=

9.007752883E-06

Bo =

39.2506869474

G (

2)

=

-6,98626E-15

SinBo=

0.632714613092

G(

3)

=

-3.70994E-20

Rb =

7918239.4709

G(4)

=

-l,0424E-27

Ro

=

7816402.7262

No

=

101836.7447

K =

12497179.1821

Coefficients

for

Grid

Scale

Factor

ko

=

0.999956851054

Mo

=

6360718.3963

F(l)

0.999956851054

ro

=

6373559.

F(2)

=

l.23088E-14

F(3)

=

5.18E-22

KS

s KANSAS SOUTH

ZONE#

1502

Oef

ining

Constants

Coefficients

for

GP

to

PC

Bs

37:16

L(l)

=

110987.8057

Bn

=

38:34

L(2)

=

9.45414

Bb

=

36:40

L(3)

=

5.64091

Lo

=

98:30

L(4)

=

0.018964

Nb =

400000.0000

Eo =

400000.0000

Coefficients

for

PC

to

GP

Computed

Constants

G(l)

=

9.009998800E-06

Bo

=

37.9176400609

G (

2)

=

-6.91489E-15

SinBo=

0.614528111936

G (

3)

=

-3.71545E-20

Rb

=

8336559.0467

G(4)

=

-l.0003E-27

Ro

=

8197720.0530

No

=

538838.9936

K

=

12697806.8013

Coefficients

for

Grid

Scale

Factor

ko

=

0.999935918480

Mo

=

6359132.8597

F(l)

=

0.999935918480

ro

=

6372455.

F(2)

=

1.

23130E-14

F(3)

=

4.91E-22

87

KY

N KENTUCKY NORTH

ZONE#

1601

Defining

Constants

Coefficients

for

GP

to

PC

Bs

=

37:58

L(l)

111001.1272

Bn

=

38:58

L(2)

=

9.49969

Bb

=

37:30

L(3)

=

5.63960

Lo

=

84:15

L(4)

=

0.019624

Nb

0.0000

Eo

=

500000.0000

Coefficients

for

PC

to

GP

Computed

Constants

G (

l)

=

9.008917501E-06

Bo

=

38.4672539691

G (

2)

=

-6.94594E-15

SinBo=

0.622067254038

G (

3)

=

-3.71303E-20·

Rb

8145306.4712

G(4)

=

-l.0140E-27

Ro

=

8037943.9917

No

=

107362.4795

K

=

12612341.7840

Coefficients

for

Grid

Scale

Factor

ko

=

0.999962079530

Mo

6359896.1212

F(l)

=

0.999962079530

ro

6373021.

F(2)

=

l.23109E-14

F(3)

=

5.03E-22

KY

s

KENTUCKY SOUTH

ZONE#

1602

Defining

Constants

Coefficients

for

GP

to

PC

Bs

=

36:44

L(l)

=

110977.8556

Bn

=

37:56

L(2)

=

9.40195

Bb

=

36:20

L(3)

=

5.64201

Lo

=

85:45

L(4)

=

0.018759

Nb

=

500000.0000

Eo

=

500000.0000

Coefficients

for

PC

to

GP

Computed

Constants

G(l)

9.010806634E-06

Bo

=

37.3341456532

G(2)

=

-6.87874E-15

SinBo=

0.606462358287

G (

3)

=

-3.71775E-20

Rb

=

8483079.4552

G(4)

=

-9.7208E-28

Ro

=

8372015.2303

No

=

611064.2249

K

12793783.0812

Coefficients

for

Grid

Scale

Factor

ko

0.999945401603

Mo

=

6358562.7562

F(l)

=

0.999945401603

ro

6372094.

F(2)

=

l.23142E-14

F(3)

=

4.82E-22

88

LA

N LOUISIANA NORTH

Defining

Constants

Bs

31:10

Bn

=

32:40

Bb

=

30:30

Lo =

92:30

Nb

=

0.0000

Eo

=

1000000.0000

Computed

Constants

Bo

=

31.9177055892

SinBo=

0.528700659421

Rb

=

10405759.0459

Ro

=

10248571.1515

No

=

157187.8944

K

=

13961752.4737

ko

=

0.999914740906

Mo

=

6352722.0540

ro

=

6368127.

LA

s LOUISIANA SOUTH

Defining

Constants

Bs

=

29:18

Bn

=

30:42

Bb

=

28:30

Lo

=

91:20

Nb

=

0.0000

Eo =

1000000.0000

Computed

Constants

Bo

30.0008395428

Sin

Bo=

0.500012689631

Rb

11221678.1079

Ro

=

11055318.6368

No

=

166359.4711

K

=

14525497.0844

ko

=

0.999925744553

Mo

=

6350906.2899

ro

=

6366937.

ZONE#

1701

Coefficients

for

GP

to

PC

L(l)

=

110875.9156

L(2)

=

8.73673

L(3)

=

5.65399

L(4)

=

0.015313

Coefficients

for

PC

to

GP

G(l)

=

9.0l9091156E-06

G(2)

=

-6.40970E-15

G(3)

-3.73877E-20

G(4)

=

-7.8031E-28

Coefficients

for

Grid

Scale

Factor

F(l)

0.999914740906

F(2)

=

l.23296E-l4

F(3)

=

3.93E-22

ZONE#

1702

Coefficients

for

GP

to

PC

L(l)

=

110844.2246

L(2)

=

8.42633

L(3)

=

5.65782

L(4)

=

0.014018

Coefficients

for

PC

to

GP

G(l)

=

9.021669771E-06

G(2)

=

-6.l8701E-l5

G(3)

=

-3.74568E-20

G(4)

=

-7.2616E-28

Coefficients

for

Grid

Scale

Factor

F(l)

=

0.999925744553

F(2)

l.23343E-l4

F(3)

=

3.64E-22

89

LA

SH

LOUISIANA

OFFSHORE

ZONE#

1703

Defining

Constants

Coefficients

for

GP

to

PC

Bs

26:10

L(l)

110791.8786

Bn

=

27:50

L(2)

=

7.86506

Bb =

25:30

L(3)

=

5.66365

Lo

91:20

L(4)

0.012775

Nb

=

0.0000

Eo =

1000000.0000

Coefficients

for

PC

to

GP

Computed

Constants

G(l)

=

9.025932193E-06

Bo

=

27.0010512832

G(2)

-5.78388E-15

SinBo=

0.454006848165

G(3)

=

-3.75631E-20

Rb =

12690863.7281

G(4)

=

-6.1764E-28

Ro

12524558.0674

No

=

166305.6607

K =

15621596.5270

Coefficients

for

Grid

Scale

Factor

ko

0.999894794114

Mo

=

6347907.1071

F(l)

=

0.999894794114

ro

=

6364866.

F(2)

=

1.

23421E-14

F(3)

=

3.23E-22

90

MD

MARYLAND

Defining

Constants

Bs

=

38:18

Bn

=

39:27

Bb

=

37:40

Lo

=

77:00

Nb

=

0.0000

Eo =

400000.0000

Computed

Constants

Bo

=

38.8757880051

SinBo=

0.627634132356

Rb

8055622.7373

Ro

=

7921405.1556

No

=

134217.5816

K

=

12551136.6396

ko

=

0.999949847842

Mo

=

6360263.7936

ro

=

6373240.

ZONE # 1900

Coefficients

for

GP

to

PC

L(l)

=

111007.5442

L(2)

=

9.53130

L(3)

=

5.63889

L(4)

0.019736

Coefficients

for

PC

to

GP

G(l)

=

9.008396710E-06

G(2)

=

-6.96769E-15

G(3)

=

-3.71144E-20

G(4)

=

-l.0352E-27

Coefficients

for

Grid

Scale

Factor

F(l)

=

0.999949847842

F(2)

=

l.23102E-14

F(3)

=

5.09E-22

91

MA

M

MASS MAINLAND

Defining

Constants

Bs =

41:43

Bn

=

42:41

Bb

=

41:00

Lo =

71:30

Nb

=

750000.0000

Eo =

200000.0000

Computed

Constants

Bo

=

42.2006252872

SinBo=

0.671728673921

Rb

7177701.7404

Ro =

7044348.7021

No

=

883353.0384

K

=

12132804.7336

ko

=

0.999964550086

Mo

=

6364028.0516

ro

=

6375786.

MA

I

MASS ISLAND

Defining

Constants

Bs

=

41:17

Bn

=

41:29

Bb

=

41:00

Lo

=

70:30

Nb

=

0.0000

Eo

=

500000.0000

Computed

Constants

Bo

41.3833593510

SinBo=

0.661093979591

Rb =

7291990.4498

Ro

7249415.2230

No

42575.2267

K =

12223979.6222

ko

0.999998482670

Mo

=

6363335.5426

ro

=

6375395.

ZONE#

2001

Coefficients

for

GP

to

PC

L(l)

=

111073.2431

L(2)

=

9.71650

L(3)

=

5.63098

L(4)

0.021759

Coefficients

for

PC

to

GP

G(l)

=

9.003068344E-06

G(2)

=

-7.09026E-15

G(3)

=

-3.69789E-20

G(4)

=

-l.1855E-27

Coefficients

for

Grid

Scale

Factor

F(l)

0.999964550086

F(2)

=

l.23003E-14

F(3)

=

5.69E-22

ZONE#

2002

Coefficients

for

GP

to

PC

L(l)

=

111061.1569

L(2)

=

9.68480

L(3)

5.62745

Coefficients

for

PC

to

GP

G(l)

=

9.004048113E-06

G(2)

=

-7.06961E-15

G(3)

-3.69799E-20

Coefficients

for

Grid

Scale

Factor

F(l)

=

0.999998482670

F(2)

=

l.23015E-14

F(3)

=

5.56E-22

92

Ml

N MICHIGAN NORTH

ZONE#

2111

Defining

Constants

Coefficients

for

GP

to

PC

Bs

=

45:29

L(l)

=

111146.0908

Bn

47:05

L(2)

9.76397

Bb =

44:47

L(3)

=

5.62053

Lo

=

87:00

L(4)

0.025777

Nb

=

0.0000

L(5)

=

0.0007325

Eo

=

8000000.0000

Coefficients

for

PC

to

GP

Computed

Constants

G(l)

=

8.997167538E-06

Bo =

46.2853056176

G(

2)

=

-7.11123E-15

SinBo=

0.722789934733

G(

3)

=

-3.68190E-20

Rb =

6275243.8434

G(

4)

=

-l.3725E-27

Ro

=

6108308.6036

G(

5)

=

8.019E-35

No

=

166935.2398

K =

11779843.7720

Coefficients

for

Grid

Scale

Factor

kc

=

0.999902834466

Mo

=

6368201.9117

F(l)

0.999902834466

ro

=

6378442.

F(2)

=

l.22919E-14

F(3)

=

6.70E-22

Ml

c MICHIGAN CENTRAL

ZONE#

2112

Defining

Constants

Coefficients

for

GP

to

PC

Bs

=

44:11

L(l)

=

111120.9691

Bn =

45:42

L(2)

=

9.77091

Bb =

43:19

L(3)

=

5.62494

Lo

84:22

L(4)

=

0.023788

Nb =

0.0000

Eo

=

6000000.0000

Coefficients

for

PC

to

GP

Computed

Constants

G(l)

8.999201531E-06

Bo =

44.9433587575

G (

2)

=

-7.12032E-15

SinBo=

0.706407406862

G(

3)

=

-3.68711E-20

Rb

=

6581660.2321

G(

4)

=

-l.3161E-27

Ro =

6400902.4399

No

=

180757.7922

K

=

11878338.0174

Coefficients

for

Grid

Scale

Factor

kc

=

0.999912706253

Mo

=

6366762.5687

F(l)

=

0.999912706253

ro

=

6377502.

F(2)

=

l.22939E-14

F(3)

=

6.25E-22

93

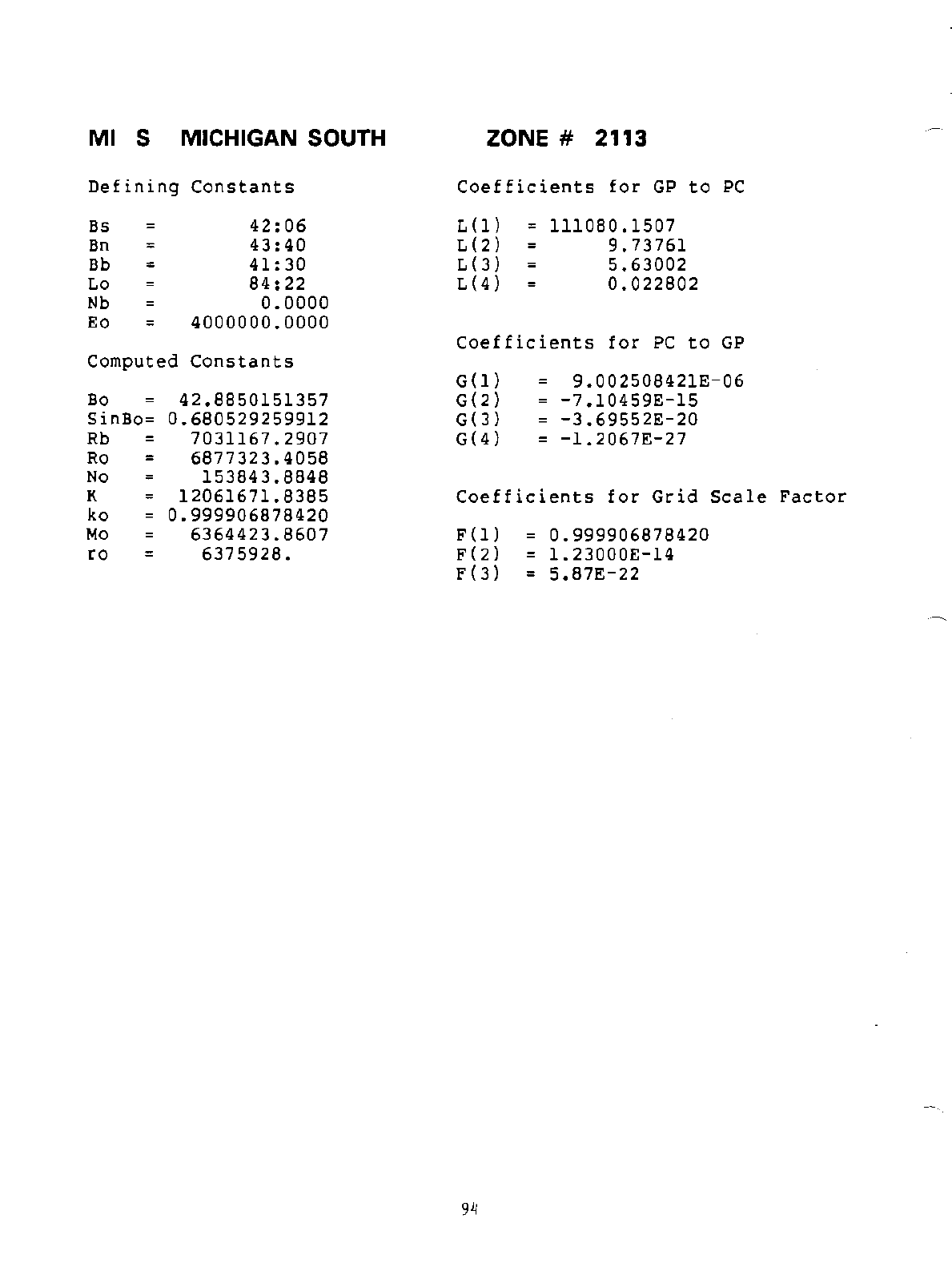
Ml

s

MICHIGAN SOUTH

Defining

Constants

Bs

42:06

Bn

=

43:40

Bb

=

41:30

Lo

84:22

Nb

0.0000

Eo

=

4000000.0000

Computed

Constants

Bo

42.8850151357

SinBo=

0.680529259912

Rb =

7031167.2907

Ro

=

6877323.4058

No

=

153843.8848

K

=

12061671.8385

ko

0.999906878420

Mo

6364423.8607

ro

=

6375928.

ZONE # 2113

Coefficients

for

GP

to

PC

L(l)

=

111080.1507

L(2)

=

9,73761

L(3)

=

5.63002

L(4)

=

0.022802

Coefficients

for

PC

to

GP

G(l)

=

9.002508421E-06

G(2)

-7.10459E-15

G(3)

-3.69552E-20

G(4)

=

-l.2067E-27

Coefficients

for

Grid

Scale

Factor

F(l)

0.999906878420

F(2)

=

l.23000E-14

F(3)

=

5.87E-22

MN

N MINNESOTA NORTH

Defining

Constants

Bs

Bn

Bb

Lo

Nb

Eo

=

47:02

48:38

46:30

93:06

100000.0000

800000.0000

Computed

Constants

Bo

=

47.8354141053

SinBo=

0.741219640371

Rb

=

5934713.4739

Ro

=

5786251.1143

No

=

248462.3596

K

=

11685145.4281

ko

=

0.999902816593

Mo

6369933.6096

ro

=

6379598.

MN

C MINNESOTA CENTRAL

Defining

Constants

Bs

Bn

Bb

Lo

Nb

Eo

=

45:37

47:03

45:00

94:15

100000.0000

800000.0000

Computed

Constants

Bo

=

SinBo=

Rb

46.3349188114

0.723388068681

6246233.9437

6097862.9029

248371.0408

11776732.4900

=

0.999922022624

6368379.6277

6378602.

Ro

No

K

ko

Mo

ro

=

ZONE#

2201

Coefficients

for

GP

to

PC

L(l)

L(2)

L(3)

L(4)

=

111176.3136

=

9.

72967

=

5.61897

=

0.

027729

Coefficients

for

PC

to

GP

G(l)

=

8.994721600E-06

G(2)

=

-7.08107E-15

G(3)

=

-3.67535E-20

G(4)

=

-l.4515E-27

Coefficients

for

Grid

Scale

Factor

F(l)

=

0.999902816593

F(2)

=

l.22867E-14

F(3)

7.04E-22

ZONE#

2202

Coefficients

for

GP

to

PC

L(l)

L(2)

L(3)

L(4)

=

111149.1920

=

9.

76378

=

5.62196

=

0.025568

Coefficients

for

PC

to

GP

G(l)

=

8.996916454E-06

G(2)

=

-7.11028E-15

G(3)

=

-3.68130E-20

G(4)

=

-l.3780E-27

Coefficients

for

Grid

Scale

Factor

F(l)

0.999922022624

F(2)

l.22899E-14

F(3)

=

6.61E-22

95

MN

S MINNESOTA SOUTH

Defining

Constants

Bs

Bn

Bb

Lo

Nb

Eo

=

43:47

45:13

43:00

94:00

100000.0000

800000.0000

Computed

Constants

Bo

=

44.5014884140

SinBo=

0.700927792688

Rb

=

6667126.8494

Ro

=

6500294.5043

No

=

266832.3451

K

=

11914387.7514

ko

=

0.999922039553

Mo

=

6366327.3480

ro

=

6377231.

ZONE#

2203

Coefficients

for

GP

to

PC

L(l)

L(2)

L(3)

L(4)

=

111113.3724

=

9.

76742

=

5.62679

=

0.

024208

Coefficients

for

PC

to

GP

G(l)

=

8.999816728E-06

G(2)

=

-7.12002E-15

G(3)

=

-3,68868E-20

G(4)

=

-l.2821E-27

Coefficients

for

Grid

Scale

Factor

F(l)

=

0.999922039553

F(2)

=

l.22957E-14

F(3)

=

6.22E-22

96

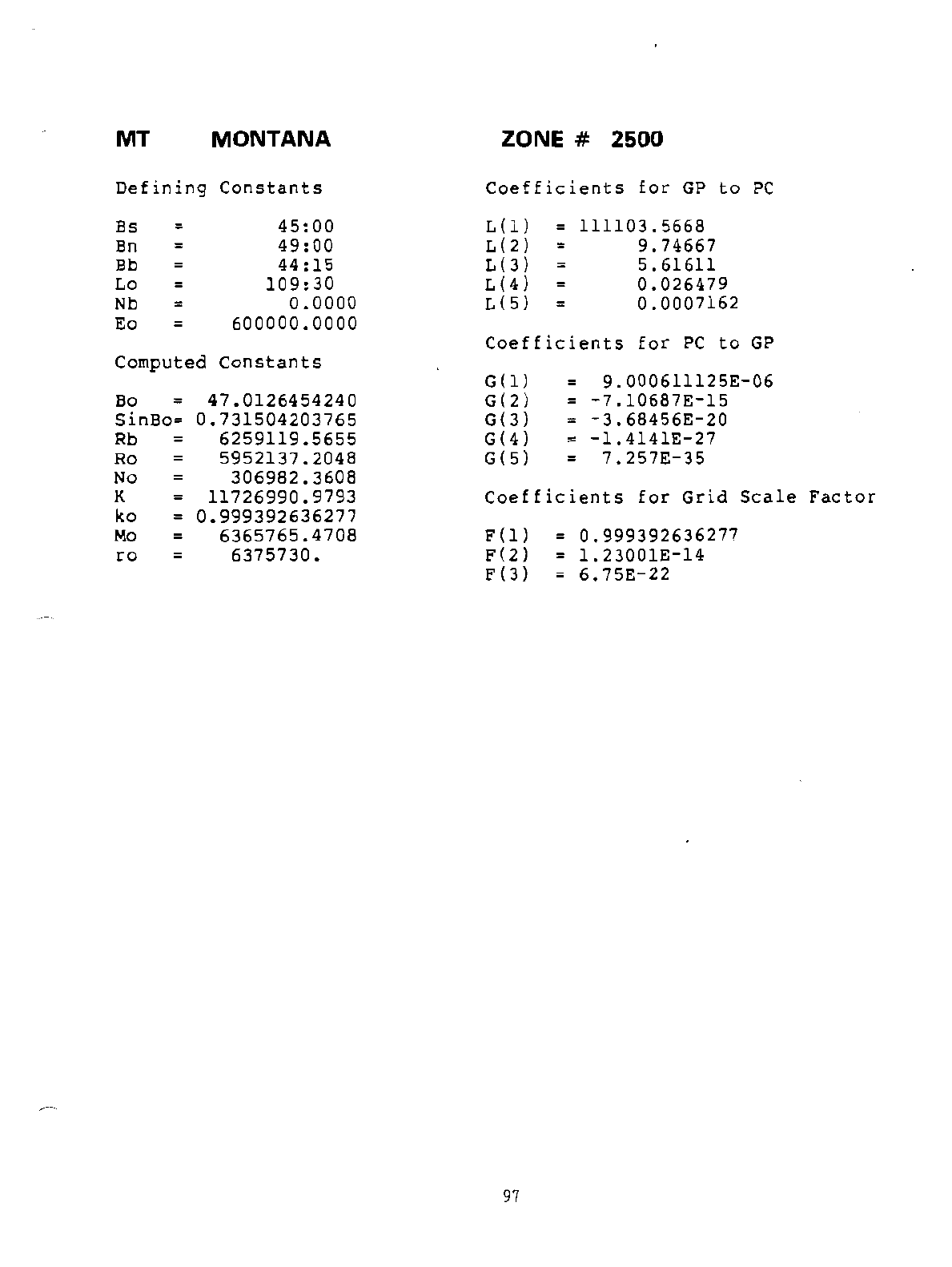
MT

MONTANA

ZONE#

2500

Defining

Constants

Coefficients

for

GP

to

PC

Bs

=

45:00

L(l)

=

111103.5668

Bn

=

49:00

L(2)

=

9.74667

Bb

=

44:15

L(3)

=

5.61611

Lo

=

109:30

L(4)

=

0.026479

Nb

=

0.0000

L( 5)

=

0.0007162

Eo

=

600000.0000

Coefficients

for

PC

to

GP

Computed

Constants

G(

l)

=

9.000611125E-06

Bo

=

47.0126454240

G ( 2)

=

-7.10687E-15

SinBo=

0.731504203765

G(

3)

=

-3.68456E-20

Rb

=

6259119.5655

G(4)

=

-l.4141E-27

Ro

5952137.2048

G(

5)

=

7.257E-35

No

306982.3608

K

=

11726990.9793

Coefficients

for

Grid

Scale

Factor

ko

=

0.999392636277

Mo

=

6365765.4708

F(l)

=

0.999392636277

ro

=

6375730.

F(2)

=

l.23001E-14

F(3)

=

6.75E-22

97

NE

NEBRASKA

ZONE#

2600

Defining

Constants

Coefficients

for

GP

to

PC

BS

=

40:00

L(l)

=

111025.7809

Bn

=

43:00

L(2)

9.68528

Bb =

39:50

L(3)

5.63025

LO

=

100:00

L(4)

=

0.021792

Nb

0.0000

L(5)

0.0006372

Eo

=

500000.0000

Coefficients

for

PC

to

GP

Computed

Constants

G(l)

=

9.006917060E-06

Bo

=

41.5058603333

G (

2)

=

-7.07688E-15

SinBo=

0.662696910933

G (

3)

-3.70427E-20

Rb =

7401530.8340

G(4)

-l.1443E-27

Ro =

7215635.9104

G(5)

=

l.251E-34

No

165694.9237

K =

12205746.1616

Coefficients

for

Grid

Scale

Factor

ko

=

0.999656595062

MO

6361308.6623

F(l)

=

0.999656595062

ro

=

6373319.

F(2)

=

l.23079E-14

F(3)

=

5.62E-22

98

NV L NEW YORK LONG ISLAND

ZONE#

3104

Defining

Constants

Bs

=

40:40

Bn

41:02

Bb

40:10

Lo

=

74:00

Nb

=

0.0000

Eo

=

300000.0000

Computed

Constants

Bo

=

40.8500858421

SinBo=

0.654082091204

Rb

7462536.3011

Ro

=

7386645.0143

No

=

75891.

2868

K

=

12287232.6151

ko

=

0.999994900400

Mo

=

6362721.8083

ro

=

6374978.

Coefficients

for

GP

to

PC

L(l)

=

111050.4466

L(2)

9.66003

L(3)

=

5.62096

Coefficients

for

PC

to

GP

G(l)

=

9.004916524E-06

G(2)

=

-7.05345E-15

G(3)

-3.69553E-20

Coefficients

for

Grid

Scale

Factor

F(l)

=

0,999994900400

F(2)

=

l.23032E-14

F(3)

=

5.44E-22

99

NC NORTH CAROLINA

Defining

Constants

Bs

=

34:20

Bn

=

36:10

Bb

=

33:45

Lo

=

79:00

Nb

0.0000

Eo

=

609601.2199

Computed

Constants

Bo

=

SinBo=

Rb

35.2517586002

0.577170255241

9199785.5932

9033195.6010

166589.9922

13178320.6222

=

0.999872591882

6355881.3611

6370148.

Ro

No

K

ko

MO

ro

ZONE#

3200

Coefficients

for

GP

to

PC

L(l)

110931.0558

L(2)

=

9.18403

L(3)

=

5.64691

L(4)

=

0.017289

Coefficients

for

PC

to

GP

G(l)

=

9.014608051E-06

G(2)

=

-6.72767E-15

G(3)

=

-3.72650E-20

G(4)

=

-8.9805E-28

Coefficients

for

Grid

Scale

Factor

F(l)

=

0.999872591882

F(2)

=

l.23215E-14

F(3)

=

4.46E-22

100

ND N NORTH DAKOTA NORTH

ZONE#

3301

Defining

Constants

Coefficients

for

GP

to

PC

Bs =

47:26

L(l)

=

111184.8361

Bn

=

48:44

L(2)

=

9.72243

Bb

47:00

L(3)

=

5.61786

Lo

=

100:30

L(4)

=

0.027700

Nb

=

0.0000

Eo =

600000.0000

Coefficients

for

PC

to

GP

Computed

Constants

G (

1)

=

8.994032200E-06

Bo =

48.0847188415

G(2)

=

-7.07375E-15

SinBo=

0.744133404458

G (

3)

=

-3.67405E-20

Rb =

5856720.4592

G (

4)

=

-l.4677E-27

Ro

=

5736120.4804

No

120599.9788

K

11672088.5605

Coefficients

for

Grid

Scale

Factor

ko

=

0.999935842096

Mo

=

6370421.8763

F(l)

=

0.999935842096

ro

=

6379995.

F(2)

=

1.

22846E-14

F(3)

=

7.08E-22

ND S NORTH DAKOTA SOUTH

ZONE#

3302

Defining

Constants

Coefficients

for

GP

to

PC

Bs

=

46:11

L(l)

=

111160.4842

Bn

=

47:29

L(2)

=

9.75568

Bb =

45:40

L(3)

5.62076

Lo =

100:30

L(4)

0.026264

Nb =

0.0000

Eo =

600000.0000

Coefficients

for

PC

to

GP

Computed

Constants

G(l)

8.996002517E-06

Bo

46.8346602257

G(2)

-7.10242E-15

SinBo=

0.729382600558

G(

3)

=

-3.67913E-20

Rb

=

6122339.5950

G(4)

-l.4014E-27

Ro

5992509.2670

No

=

129830.3280

K =

11744429.2917

Coefficients

for

Grid

Scale

Factor

ko

0.999935851558

Mo

=

6369026.6161

F(l)

=

0.999935851558

ro

=

6379063.

F(2)

=

l.22881E-14

F(3)

=

6.75E-22

1

01

OH

N OHIO NORTH

ZONE#

3401

Defining

Constants

Coefficients

for

GP

to

PC

Bs

40:26

L(l)

111048.4575

Bn

41:42

L(2)

=

9.66786

Bb

=

39:40

L(3)

=

5.63397

Lo

82:30

L(4)

=

0.021060

Nb

=

0.0000

Eo

=

600000.0000

Coefficients

for

PC

to

GP

Computed

Constants

G(l)

=

9.005077760E-06

Bo

=

41.0676989228

G(2)

=

-7.059431!:-15

SinBo=

0.656950312341

G(3)

-3.70266E-20

Rb

=

7485451.5983

G(4)

=

-1.13291!:-27

Ro

=

7329672.6916

No

=

155576.9068

K

=

12260321.3670

Coefficients

for

Grid

Scale

Factor

ko

=

0.999939140422

Mo

=

6362607.9595

F(l)

=

0.999939140422

ro

=

6374783.

F(2)

=

l.23043E-14

F(3)

5.46E:-22

OH

s OHIO SOUTH

ZONE#

3402

Defining

Constants

Coefficients

for

GP

to

PC

Bs

=

36:44

L(l)

=

111015.7097

Bn

=

40:02

L(2)

9.56783

Bb

=

38:00

L(3)

5.63800

Lo

=

82:30

L(4)

=

0.020061

Nb

=

0.0000

Eo

600000.0000

Coefficients

for

PC

to

GP

Computed

Constants

G(l)

=

9.007734067E-06

Bo

=

39.3643565116

G(2)

=

-6.99261E-15

SinBo=

0.634519536768

G(3)

=

-3.70945E-20

Rb

=

7932669.0374

G(4)

=

-l.0564E-27

Ro

=

7779186.9467

No

=

153662.0906

K

=

12478096.2534

Coefficients

for

Grid

Scale

Factor

ko

=

0.999935907660

Mo

=

6360731.6569

F(l)

0.999935907660

ro

=

6373523.

F(2)

1.

23093E-14

F(3)

=

5.18E-22

1

02

OK

N OKLAHOMA NORTH

Defining

Constants

Bs

Bn

Bb

Lo

Nb

Eo

=

35:34

36:46

35:00

98:00

0.0000

600000.0000

Computed

Constants

Bo

36.1674456022

SinBo=

0.590147072450

Rb

=

8864259.9258

Ro

8734728.4814

No

129531.4444

K =

13001040.2487

ko

0.999945408786

Mo

=

6357313.3855

ro

=

6371260.

OK

s

OKLAHOMA SOUTH

Defining

Constants

Bs =

33:56

Bn

35:14

Bb

=

33:20

Lo =

98:00

Nb

0.0000

Eo

600000.0000

Computed

Constants

Bo

=

34.5841961094

SinBo=

0.567616677812

Rb

=

9399243.5141

Ro

=

9260493.6985

No

138749.8157

K

13318364.4294

ko

=

0.999935942436

Mo

=

6355584.5004

ro

=

6370084.

ZONE#

3501

Coefficients

for

GP

to

PC

L(l)

L(2)

L(3)

L(4)

=

110956.0498

=

9.28617

=

5.64482

=

0.017979

Coefficients

for

PC

to

GP

G(l)

=

9.012577476E-06

G(2)

-6.79804E-15

G(3)

-3.72229E-20

G(4)

=

-9.2812E-28

Coefficients

for

Grid

Scale

F(l)

=

0.999945408786

F(2)

=

l.23177E-14

F(3)

=

4.62E-22

ZONE#

3502

Coefficients

for

GP

to

PC

L(l)

=

110925.8751

L(2)

=

9.10472

L(3)

5.64812

L(4)

=

0.016766

Coefficients

for

PC

to

GP

G (

1)

=

9.015029132E-06

G (

2)

=

-6.67047E-15

G (

3)

=

-3.72859E-20

G(4)

=

-8.7733E-28

Coefficients

for

Grid

Scale

F(l)

=

0.999935942436

F(2)

=

l.23220E-14

F(3)

=

4.34E-22

103

Factor

OR

N

OREGON NORTH

ZONE#

3601

Defining

Constants

Coefficients

for

GP

to

PC

Bs

=

44:20

L(l)

=

111123.3583

Bn

=

46:00

L(2)

=

9.77067

Bb

=

43:40

L(3)

=

5.62487

Lo

120:30

L(4)

=

0.024544

Nb

=

0.0000

Eo

=

2500000.0000

Coefficients

for

PC

to

GP

Computed

Constants

G(l)

=

8.999007999E-06

Bo

45.1687259619

G(

2)

-7.12020E-15

SinBo=

0,709186016884

G (

3)

-3.68630E-20

Rb

=

6517624.6963

G(4)

=

-l,3188E-27

Ro

=

6350713.9300

No

=

166910.7663

K

=

11860484.1452

Coefficients

for

Grid

Scale

Factor

ko

=

0.999894582577

Mo

=

6366899.4862

F(l)

0.999894582577

ro

=

6377555.

F(2)

=

l.22939E-14

F(3)

=

6.35E-22

OR

s

OREGON SOUTH

ZONE#

3602

Defining

Constants

Coefficients

for

GP

to

PC

Bs

=

42:20

L(l)

=

111084.3129

Bn

44:00

L(2)

=

9.74486

Bb

=

41:40

L(3)

=

5.62774

Lo

=

120:30

L(4)

=

0.023107

Nb

=

0.0000

L( 5)

0.0006671

Eo

1500000.0000

Coefficients

for

PC

to

GP

Computed

Constants

G(

1)

9.002171179E-06

Bo

=

43.1685887665

G(

2)

=

-7.10916E-15

Sin

Bo=

0.684147361010

G(

3)

-3.69482E-20

Rb

6976289.2382

G(4)

=

-l.2185E-27

Ro

=

6809452.2816

G(

5)

=

l.lllE-34

No

=

166836.9566

K

=

12033772.6984

Coefficients

for

Grid

Scale

Factor

ko =

0.999894607592

Mo

=

6364662.2994

F(l)

=

0.999894607592

ro

=

6376061.

F(2)

=

l.23002E-14

F(3)

=

5.97E-22

104

PA

N PENNSYLVANIA NORTH

ZONE#

3701

Defining

Constants

Coefficients

for

GP

to

PC

Bs =

40:53

L(l)

=

111057,1908

Bn

=

41:57

L(2)

=

9.68441

Bb

=

40:10

L (

3)

=

5.63320

Lo

77:45

L(4)

=

0.021500

Nb

=

0.0000

Eo

=

600000.0000

Coefficients

for

PC

to

GP

Computed

Constants

G(l)

=

9.004369625E-06

Bo

=

41.4174076242

G(2)

-7.07004E-15

SinBo=

0.661539733811

G(3)

-3.

70106E-20

Rb

=

7379348.3668

G(4)

=

-l.1439E-27

Ro

=

7240448.7701

No

138899.5967

K =

12219540.4665

Coefficients

for

Grid

Scale

Factor

ko

=

0.999956840202

Mo

=

6363108.3386

F(l)

=

0.999956840202

ro

=

6375155.

F(2)

=

l.23030E-14

F(3)

5.56E-22

PA

s

PENNSYLVANIA SOUTH

ZONE#

3702

Defining

Constants

Coefficients

for

GP

to

PC

Bs =

39:56

L(l)

=

111038.8080

Bn

=

40:58

L(2)

=

9.63502

Bb

=

39:20

L(3)

=

5.63528

Lo =

77:45

L(4)

=

0.020898

Nb

=

0.0000

Eo

=

600000.0000

Coefficients

for

PC

to

GP

Computed

Constants

G(

1)

9.005860337E-06

Bo

=

40.4506723597

G(2)

=

-7.03760E-15

SinBo=

0.648793151619

G(

3)

-3,70500E-20

Rb

=

7615193.7581

G(4)

-l.0995E-27

Ro

=

7491129.9649

No

=

124063.7931

K

=

12336392.1867

Coefficients

for

Grid

Scale

Factor

ko =

0.999959500101

Mo

=

6362055.0747

F(l)

=

0.999959500101

ro

=

6374457.

F(2)

l.23055E-14

F(3)

=

5.38E-22

105

SC

SOUTH CAROLINA

ZONE#

3900

Defining

Constants

Coefficients

for

GP

to

PC

Bs =

32:30

L(l)

=

110893.5412

Bn

34:50

L(2)

8.98578

Bb =

31:50

L(3)

=

5.64832

Lo =

81:00

L(4)

=

0.016390

Nb

0.0000

L(5)

=

0.0005454

Eo =

609600.0000

Coefficients

for

PC

to

GP

Computed

Constants

G(l)

=

9.017657737E-06

Bo

=

33.6693534716

G(2)

=

-6.58928E-15

SinBo=

0.554399350127

G(3)

=

-3.73407E-20

Rb =

9786198.7935

G(4)

-8.3932E-28

Ro

=

9582591.5259

G(

5)

=

l.748E-34

No

203607.2676

K =

13520786.8598

Coefficients

for

Grid

Scale

Factor

ko

=

0.999793656965

Mo

6353731.8876

F(l)

0.999793656965

ro

=

6368544.

F(2)

=

l.23274E-14

F(3)

=

4.21E-22

106

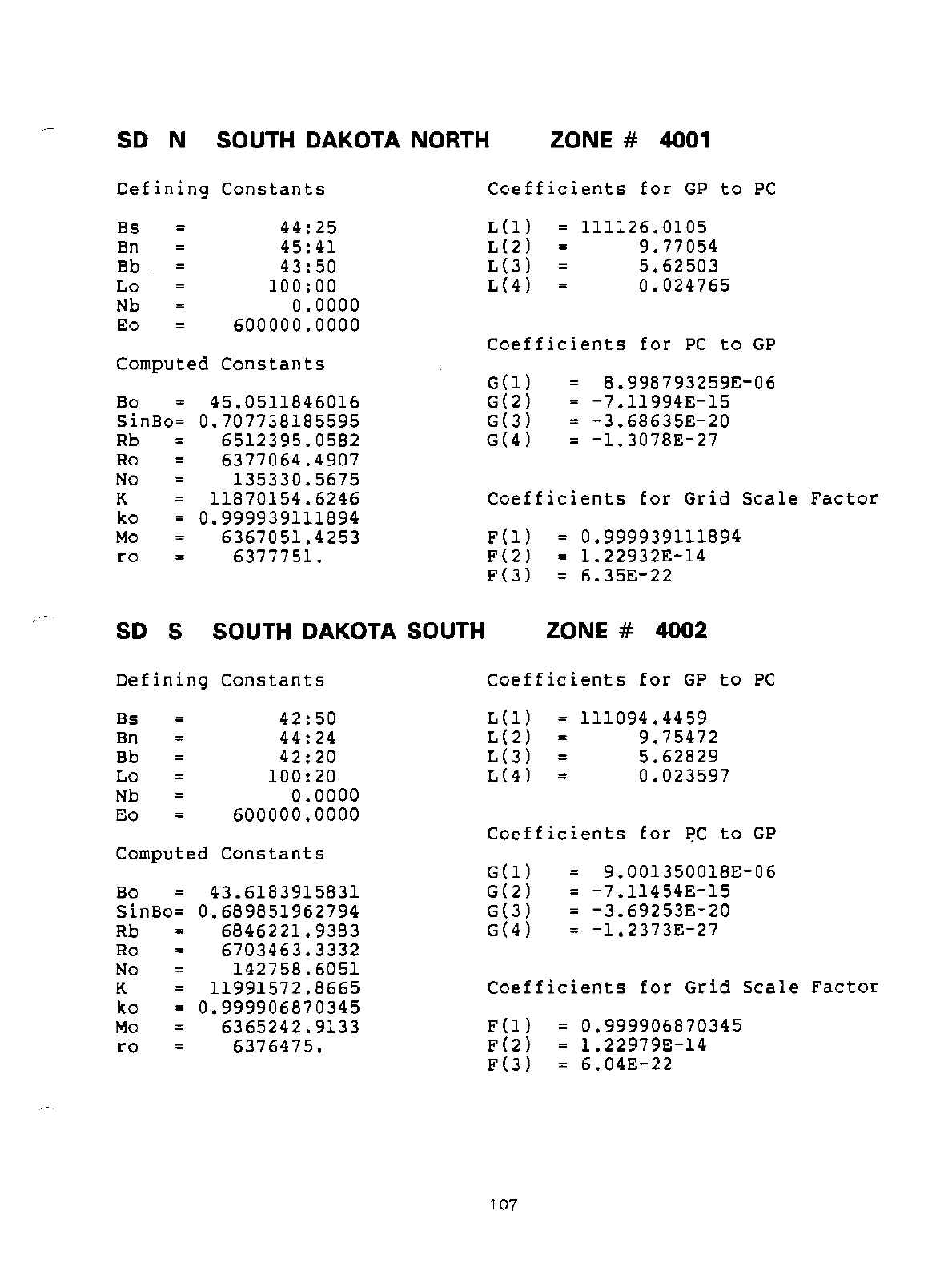
SD N SOUTH DAKOTA NORTH

ZONE#

4001

Defining

Constants

Coefficients

for

GP

to

PC

Bs

=

44:25

L(l)

111126.0105

Bn

45:41

L(2)

=

9.77054

Bb

=

43:50

L(3)

=

5.62503

Lo

100:00

L(4)

=

0.024765

Nb

=

0.0000

Eo

=

600000.0000

Coefficients

for

PC

to

GP

Computed

Constants

G(l)

=

6.996793259E-06

Bo

=

45.0511646016

G(2)

=

-7.11994E-15

SinBo=

0.707736165595

G(3)

=

-3.66635E-20

Rb

=

6512395.0562

G (

4)

=

-l.3076E-27

Ro

=

6377064.4907

No

=

135330.5675

K

=

11670154.6246

Coefficients

for

Grid

Scale

Factor

ko

=

0.999939111694

Mo

6367051.4253

F(l)

=

0.999939111694

ro

6377751.

F(2)

=

l.22932E-14

F(3)

=

6.35E-22

SD

s SOUTH DAKOTA SOUTH

ZONE#

4002

Defining

Constants

Coefficients

for

GP

to

PC

Bs

=

42:50

L(l)

111094.4459

Bn

=

44:24

L(2)

=

9.75472

Bb

42:20

L(3)

=

5.62629

Lo

100:20

L(4)

=

0.023597

Nb

=

0.0000

Eo

=

600000.0000

Coefficients

for

!'C

to

GP

Computed

Constants

G(l)

=

9.001350016E-06

Bo

=

43.6183915831

G(2)

=

-7.ll454E-15

SinBo=

0.689651962794

G(3)

=

-3.69253E-20

Rb

=

6846221.9383

G(4)

=

-l.2373E-27

Ro

=

6703463.3332

No

=

142756.6051

K

=

11991572.8665

Coefficients

for

Grid

Scale

Factor

ko

=

0.999906870345

Mo

=

6365242.9133

F(l)

=

0.999906670345

ro

=

6376475.

F(2)

l.22979E-14

F(3)

6.04E-22

107

TN

TENNESSEE

Defining

Constants

Bs

Bn

Bb

Lo

Nb

Eo

=

35:15

36:25

34:20

86:00

0.0000

600000.0000

Computed

Constants

Bo

=

35.8340607459

SinBo=

0.585439726459

Rb =

9008631.3113

Ro

=

8842127.1422

No

=

166504.1691

K

=

13064326.2967

ko

=

0.999948401424

Mo

=

6356978.3321

ro

=

6371042.

ZONE#

4100

Coefficients

for

GP

to

PC

L(l)

L(2)

L(3)

L(4)

110950.2019

=

9.25072

=

5.64572

=

0.017374

Coefficients

for

PC

to

GP

G(l)

=

9.0l3052490E-06

G(2)

=

-6.77268E-15

G(3)

=

-3.72351E-20

G(4)

=

-9.2828E-28

Coefficients

for

Grid

Scale

Factor

F(l)

=

0.999948401424

F(2)

=

l.23188E-14

F(3)

=

4.54E-22

1 08

TX

N

TEXAS NORTH

Defining

Constants

Bs

=

34:39

Bn

36:11

Bb =

34:00

Lo

=

101:30

Nb

=

1000000.0000

Eo =

200000.0000

Computed

Constants

Bo

=

35.4179042823

SinBo=

0.579535862261

Rb

=

9135570.8896

Ro

8978273.3931

No

=

1157297.4965

K =

13145417.7356

ko =

0.999910875663

Mo

=

6356299.7601

ro

=

6370509.

ZONE#

4201

Coefficients

for

GP

to

PC

L(l)

=

110938.3584

L(2)

=

9.20339

L(3)

=

5.64670

L(4)

=

0.017491

Coefficients

for

PC

to

GP

G(l)

=

9.014014675E-06

G(2)

-6.74066E-l5

G(3)

-3.

72545E-20

G(4)

=

-9.0079E-28

Coefficients

for

Grid

Scale

Factor

F(l)

0.999910875663

F(2)

=

l.23205E-l4

F(3)

=

4.49E-22

TX

NC TEXAS NORTH CENTRAL

ZONE#

4202

Defining

Constants

Bs =

32:08

Bn

=

33:58

Bb

=

31:40

Lo =

98:30

Nb

=

2000000.0000

Eo

600000.0000

Computed

Constants

Bo

=

33.0516205542

SinBo=

0.545394412971

Rb

9964225.7538

Ro

9810648.6091

No

=

2153577.1446

K

13669256.3042

ko =

0.999872622628

Mo

=

6353600.5552

ro

=

6368624.

Coefficients

for

GP

to

PC

L(l)

=

110891.2484

L(2)

8.90195

L(3)

5.65144

L(4)

=

0.016070

Coefficients

for

PC

to

GP

G(l)

=

9.017844103E-06

G(2)

=

-6.52831E-l5

G(3)

-3.

73499E-20

G(4)

-8.l560E-28

Coefficients

for

Grid

Scale

Factor

F(l)

=

0.999872622628

F(2)

=

l.23272E-l4

F(3)

=

4.llE-22

109

TX

c

TEXAS CENTRAL

Defining

Constants

Bs

30:07

Bn

31:53

Bb

=

29:40

Lo =

100:20

Nb

=

3000000.0000

Eo =

700000.0000

Computed

Constants

Bo

31.0013908377

SinBo=

0.515058882235

Rb =

10770561.1034

Ro

=

10622600.3250

No

=

3147960.7784

K =

14219009.8813

ko

=

0.999881743629

Mo

=

6351602.5419

ro

=

6367308.

ZONE#

4203

Coefficients

for

GP

to

PC

L(l)

110856.3764

L(2)

8.59215

L(3)

=

5.65568

L(4)

0.015131

Coefficients

for

PC

to

GP

G(l)

=

9.020680826E-06

G(2)

-6,30758E-l5

G(3)

-3.74251E-20

G(4)

=

-7.3651E-28

Coefficients

for

Grid

Scale

Factor

F(l)

=

0,999881743629

F(2)

=

l.23324E-l4

F(3)

=

3.81E-22

TX

SC

TEXAS SOUTH CENTRAL

ZONE#

4204

Defining

Constants

Bs =

28:23

Bn

=

30:17

Bb

=

27:50

Lo =

99:00

Nb

=

4000000.0000

Eo =

600000.0000

Computed

Constants

Bo

=

29.3348388416

SinBo=

0.489912625143

Rb =

11523512.5584

Ro

=

11357106.1291

No

=

4166406.4293

K

=

14743501.7826

ko =

0.999863243591

Mo

=

6349870.7242

ro

=

6366112.

Coefficients

for

GP

to

PC

L(l)

=

110826.1504

L(2)

=

8.30885

L(3)

=

5.65894

L(4)

=

0.013811

Coefficients

for

PC

to

GP

G(l)

=

9,023141055E-06

G(2)

=

-6.10399E-15

G(3)

=

-3.74868E-20

G(4)

=

-6.9867E-28

Coefficients

for

Grid

Scale

Factor

F(l)

0,999863243591

F(2)

=

l.23368E-14

F(3)

=

3.55E-22

11

0

TX

S TEXAS SOUTH

Defining

Constants

Bs

Bn

Bb

Lo

Nb

Eo

=

26:10

27:50

25:40

98:30

5000000.0000

300000.0000

Computed

Constants

Bo

=

27.0010512832

SinBo=

0.454006848165

Rb

12672396.4573

Ro

=

12524558.0674

No

=

5147838.3899

K

15621596.5270

ko

=

0.999894794114

Mo

6347907.1071

ro

6364866.

ZONE#

4205

Coefficients

for

GP

to

PC

L(l)

L(2)

L(3)

L(4)

=

110791.8791

=

7.86549

=

5.66316

=

0.

012519

Coefficients

for

PC

to

GP

G(l)

=

9.025932226E-06

G(2)

=

-5,78365E-15

G(3)

=

-3.75655E-20

G(4)

=

-6.2913E-28

Coefficients

for

Grid

Scale

Factor

F(l)

0.999894794114

F(2)

=

l.23417E-14

F(3)

=

3.21E-22

111

UT N

UTAH NORTH

Defining

Constants

Bs

=

40:43

Bn

=

41:47

Bb

=

40:20

Lo

=

111:30

Nb

=

1000000.0000

Eo

=

500000.0000

Computed

Constants

Bo

=

41.2507366798

SinBo=

0.659355481817

Rb

=

7384852.1452

Ro

=

7282974.6766

No

1101877.4686

K

=

12238904.9538

ko

=

0.999956841041

Mo

6362923.4572

ro

=

6375032.

UT c UTAH CENTRAL

Defining

Constants

Bs

=

39:01

Bn

=

40:39

Bb

=

38:20

Lo

=

111:30

Nb

=

2000000.0000

Eo

500000.0000

Computed

Constants

Bo

=

39.8349774741

SinBo=

0.640578595825

Rb

7822240.6085

Ro

7655530.3911

No

=

2166710.2174

K

12415886.8989

ko

=

0.999898820765

Mo

=

6360990.5575

ro

=

6373617.

ZONE#

4301

Coefficients

for

GP

to

PC

L(l)

111053.9642

L(2)

=

9.67638

L(3)

=

5.63329

L(4)

0.021795

Coefficients

for

PC

to

GP

G(l)

=

9.004631262E-06

G(2)

-7.065llE-l5

G(3)

-3.70181E-20

G(4)

=

-l.l272E-27

Coefficients

for

Grid

Scale

Factor

F(l)

0.999956841041

F(2)

=

l.23032E-l4

F(3)

5.56E-22

ZONE#

4302

Coefficients

for

GP

to

PC

L(l)

=

111020.2282

L(2)

=

9,59755

L(3)

=

5.63694

L(4)

=

0.020325

Coefficients

for

PC

~o

GP

G(l)

9.007367459E-06

G(2)

=

-7.01354E-l5

G(3)

-3.70800E-20

G(4)

-l.0771E-27

Coefficients

for

Grid

Scale

Factor

F(l)

=

0.999898820765

F(2)

=

l.23087E-l4

F(3)

=

5.26E-22

11

2

UT s UTAH SOUTH

Defining

Constants

Bs

37:13

Bn

38:21

Bb

=

36:40

Lo

111:30

Nb

=

3000000.0000

Eo

=

500000.0000

Computed

Constants

Bo

=

37.7840696241

SinBo=

0.612687337234

Rb

=

8361336.2313

Ro

=

8237322.9910

No

=

3124013.2403

K

=

12719504.1729

ko

=

0.999951297078

Mo

6359086.0437

ro

=

6372457.

ZONE#

4303

Coefficients

for

GP

to

PC

L(l)

110986.9886

L(2)

=

9.44259

L(3)

=

5.64118

L(4)

=

0.018991

Coefficients

for

PC

to

GP

G(l)

=

9.010065135E-06

G(2)

-6.90671E-15

G(3)

=

-3.71585E-20

G(4)

=

-9.9163E-28

Coefficients

for

Grid

Scale

Factor

F(l)

=

0.999951297078

F(2)

=

l.23130E-14

F(3)

=

4.89E-22

11

3

VA

N VIRGINIA NORTH

ZONE#

4501

Defining

Constants

Coefficients

for

GP

to

PC

Bs

=

38:02

L(l)

=

111002.4628

Bn

39:12

L(2)

=

9.51137

Bb =

37:40

L(3)

=

5.63918

Lo

78:30

L(4)

=

0.019770

Nb =

2000000.0000

Eo =

3500000.0000

Coefficients

for

PC

to

GP

Computed

Constants

G (

l)

=

9.008809l02E-06

Bo =

38.6174703154

G (

2)

=

-6.95425E-l5

SinBo=

0.624117864647

G (

3)

-3.7l258E-20

Rb =

8100315.6826

G(4)

=

-l.Ol90E-27

Ro

=

7994777.9034

No

2105537.7792

K =

12589455.5135

Coefficients

for

Grid

Scale

Factor

ko

=

0.999948385156

Mo

6359972.6472

F(l)

=

0.999948385156

ro

=

6373043.

F(2)

=

l.23l06E-l4

F(3)

5.06E-22

VA s VIRGINIA SOUTH

ZONE#

4502

Defining

Constants

Coef·ficients

for

GP

to

PC

Bs

=

36:46

L(l)

=

110978.4824

Bn

=

37:58

L(2)

=

9.40495

Bb

36:20

L(3)

=

5.64206

Lo

=

78:30

L(4)

=

0.018900

Nb =

1000000.0000

Eo

=

3500000.0000

Coefficients

for

PC

to

GP

Computed

Constants

G (

l)

9.0l075573lE-06

Bo

=

37.3674799550

G(

2)

=

-6.88091E-l5

SinBo=

0.606924846589

G(3)

=

-3.7l758E-20

Rb

8476701.8059

G(4)

=

-9.6990E-28

Ro

8361937.6230

No

=

1114764.1829

K

12788171.0476

Coefficients

for

Grid

Scale

Factor

ko

=

0.999945401397

Mo

=

6358598.6747

F(l)

=

0.999945401397

ro

=

6372118.

F(2)

=

l.23l43E-14

F(3)

=

4.83E-22

11

4

WA N

WASHINGTON NORTH

ZONE#

4601

Defining

Constants

Coefficients

for

GP

to

PC

Bs

47:30

L(l)

111186.1944

Bn

=

48:44

L(2)

=

9.72145

Bb

=

47:00

L(3)

=

5.61785

Lo =

120:50

L(4)

=

0.027630

Nb

=

0.0000

Eo =

500000.0000

Coefficients

for

PC

to

GP

Computed

Constants

G (

1)

=

8.993922319E-06

Bo

=

48.1179151437

G(2)

=

-7.07270E-15

SinBo=

0.744520326553

G (

3)

-3.67384E-20

Rb =

5853778.6038

G(

4)

=

-1.

4

705E-27

Ro

=

5729486.2170

No

124292.3869

K =

11670409.5559

Coefficients

for

Grid

Scale

Factor

ko =

0.999942253481

Mo

=

6370499.7054

F(l)

0.999942253481

ro

6380060.

F(2)

l.22844E-14

F(3)

=

7.08E-22

WA

s WASHINGTON SOUTH

ZONE#

4602

Defining

Constants

Coefficients

for

GP

to

PC

Bs

=

45:50

L(l)

=

111153.2505

Bn

=

47:20

L(2)

=

9.75921

Bb =

45:20

L(3)

=

5.62165

Lo =

120:30

L(4)

=

0.026539

Nb

=

0.0000

Eo =

500000.0000

Coefficients

for

PC

to

GP

Computed

Constants

G(l)

=

8.996587928E-06

Bo

=

46.5850847865

G(2)

-7.10693E-15

SinBo=

0.726395784020

G (

3)

=

-3.68032E-20

Rb

6183952.2755

G(4)

=

-l,3823E-27

Ro

6044820.3632

No

=

139131.9123

K =

11760132.9643

Coefficients

for

Grid

Scale

Factor

ko

=

0.999914597644

Mo

=

6368612.1773

F(l)

=

0.999914597644

ro

6378741.

F(2)

=

l.22897E-14

F(3)

=

6.73E-22

11

5

WV

N WEST VIRGINIA NORTH

ZONE#

4701

Defining

Constants

Coefficients

for

GP

to

PC

Bs

=

39:00

L(l)

111020.8737

Bn

=

40:15

L(2)

=

9.58417

Bb

=

38:30

L(3)

=

5.63702

Lo

79:30

L(4)

=

0.020271

Nb

=

0.0000

Eo =

600000.0000

Coefficients

for

PC

to

GP

Computed

Constants

G (

1)

=

9.007315138E-06

Bo =

39.6259559060

G (

2)

-7.00383E-15

SinBo=

0.637772979172

G (

3)

=

-3.70855E-20

Rb

=

7837787.7954

G(4)

=

-l.0658E-27

Ro

=

7712787.3235

No

=

125000.4720

K =

12444726.9475

Coefficients

for

Grid

Scale

Factor

ko =

0.999940741388

Mo

=

6361027.5180

F(l)

=

0.999940741388

ro

=

6373731.

F(2)

=

1.

23081E-14

F(3)

5.23E-22

WV

s

WEST VIRGINIA SOUTH

ZONE#

4702

Defining

Constants

Coefficients

for

GP

to

PC

Bs

=

37:29

L(l)

=

110991.7203

Bn

=

38:53

L(2)

=

9.47644

Bb

37:00

L(3)

=

5.64030

Lo =

81:00

L(4)

=

0.019308

Nb

=

0.0000

Eo

=

600000.0000

Coefficients

for

PC

to

GP

Computed

Constants

G (

1)

=

9.009681018E-06

Bo

=

38.1844729967

G (

2)

=

-6.93061E-15

Sin

Bo=

0.618195407531

G (

3)

-3.71449E-20

Rb =

8250940.5496

G (

4)

=

-l.0063E-27

Ro

=

8119477.8143

No

=

131462.7353

K

12655491.0285

Coefficients

for

Grid

Scale

Factor

ko =

0.999925678359

Mo

6359357.1532

F(l)

=

0.999925678359

ro

=

6372583.

F(2)

=

l.23124E-14

F(3)

=

4.97E-22

11

6

WI

N WISCONSIN NORTH

ZONE#

4801

Defining

Constants

Coefficients

for

GP

to

PC

Bs

=

45:34

L(l)

=

111148.5205

Bn

46:46

L(2)

=

9.76579

Bb

=

45:10

L(3)

=

5.62201

Lo =

90:00

L(4)

=

0.025652

Nb

=

0.0000

Eo =

600000.0000

Coefficients

for

PC

to

GP

Computed

Constants

G(l)

=

8.996970839E-06

Bo

46.1677715519

G(2)

=

-7.11207E-15

SinBo=

0.721370788570

G (

3)

=

-3.68179E-20

Rb =

6244929.5105

G(4)

=

-l.3661E-27

Ro

=

6133662.3561

No

111267.1544

K =

11788334.3169

Coefficients

for

Grid

Scale

Factor

ko

=

0.999945345317

Mo

=

6368341,1351

F(l)

0.999945345317

ro

=

6378625.

F(2)

=

1.22894E-14

F(3)

=

6.59E-22

WI

c

WISCONSIN CENTRAL

ZONE#

4802

Defining

Constants

Coefficients

for

GP

to

PC

Bs =

44:15

L(l)

=

111122.7674

Bn

=

45:30

L(2)

=

9.76998

Bb =

43:50

L(3)

5.62509

Lo =

90:00

L(4)

=

0.024632

Nb

=

0.0000

Eo =

600000.0000

Coefficients

for

PC

to

GP

Computed

Constants

G(l)

=

8.999055911E-06

Bo

44.8761466967

G (

2)

=

-7.12016E-15

SinBo=

0.705576614409

G(3)

=

-3.68710E-20

Rb

6531967.9926

G(4)

-1.2987E-27

Ro

6416091.9604

No

=

115876.0322

K =

11884020.9704

Coefficients

for

Grid

Scale

Factor

ko

=

0.999940704902

Mo

=

6366865.5955

F(l)

=

0.999940704902

ro

6377630.

F(2)

=

1.22933E-14

F(3)

=

6.31E-22

11

7

WI

S WISCONSIN SOUTH

Defining

Constants

Bs

Bn

Bb

Lo

Nb

Eo

=

42:44

44:04

42:00

90:00

0.0000

600000.0000

Computed

Constants

Bo

=

43.4012400263

SinBo=

0.687103235566

Rb

=

6910290.1546

Ro

=

6754625.8558

No

=

155664.2988

K =

12012072.0457

ko

=

0.999932547079

Mo

=

6365163.6776

ro

=

6376476.

ZONE#

4803

Coefficients

for

GP

to

PC

L(l)

L(2)

L(3)

L(4)

=

111093.0630

=

9.75085

=

5.62892

0.023110

Coefficients

for

PC

to

GP

G(l)

=

9.001462070E-06

G(2)

=

-7.11165E-15

G(3)

=

-3.69314E-20

G(4)

=

-l.2326E-27

Coefficients

for

Grid

Scale

Factor

F(l)

0.999932547079

F(2)

=

l.22981E-14

F(3)

=

5.97E-22

118

PR

VI

PUERTO

RICO

& VIRGIN I ZONE # 5200

Defining

Constants

Coefficients

for

GP

to

PC

Bs

=

18:02

L(l)

=

110682.3958

Bn

18:26

L(2)

=

5.76845

Bb

=

17:50

L(3)

=

5.67659

Lo

=

66:26

L(4)

=

0.008098

Nb

200000.0000

Eo

=

200000.0000

Coefficients

for

PC

to

GP

Computed

Constants

G(

1)

=

9.034860445E-06

Bo

=

18.2333725907

G(

2)

-4.25426E-15

Sin

Bo=

0.312888187729

G(

3)

-3.78192E-20

Rb

=

19411706.1974

G(

4)

=

-3.9493E-28

Ro

19367429.4540

No

=

244276.7435

K

21418025.2279

Coefficients

for

Grid

Scale

Factor

ko

=

0.999993944472

Mo

=

6341634.1470

F(l)

=

0.999993944472

ro

=

6360883.

F(2)

=

l.23576E-14

F(3)

2.08E-22

11

9